Which Statement About Equations And Expressions Is True
News Leon
Mar 31, 2025 · 5 min read
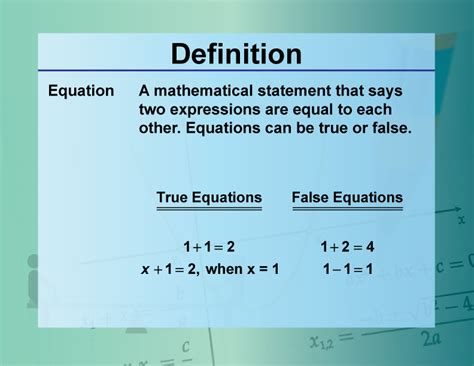
Table of Contents
Which Statement About Equations and Expressions is True? A Deep Dive into Mathematical Fundamentals
Understanding the difference between equations and expressions is fundamental to mastering algebra and beyond. Many students stumble at this initial hurdle, leading to difficulties in more advanced mathematical concepts. This article will thoroughly explore the distinctions between equations and expressions, clarifying common misconceptions and providing a robust understanding of their properties. We'll delve into the core differences, examine examples, and unravel why one statement definitively stands out as true regarding their nature. By the end, you'll not only know the correct answer but also possess a solid foundation for tackling more complex mathematical problems.
Equations vs. Expressions: The Fundamental Differences
Before we can determine which statement about equations and expressions is true, let's establish a clear understanding of what each represents.
Expressions: The Building Blocks of Mathematics
A mathematical expression is a combination of numbers, variables, and mathematical operations (like addition, subtraction, multiplication, and division) that represents a single value. Crucially, an expression does not contain an equals sign (=). It's simply a mathematical phrase.
Examples of Expressions:
3x + 52(a + b)x² - 4√(16 + 9)4/5
These expressions can be simplified or evaluated if values are assigned to the variables, but they don't assert a relationship of equality. They simply represent a quantity.
Equations: Statements of Equality
In contrast to an expression, an equation is a statement that asserts the equality of two expressions. The hallmark of an equation is the presence of an equals sign (=). Equations can be true or false, depending on the values of the variables involved. The primary goal when working with equations is often to find the value(s) of the variable(s) that make the equation true. This process is known as solving the equation.
Examples of Equations:
3x + 5 = 142(a + b) = 10x² - 4 = 0y = 2x + 11/2 = 0.5
These equations declare that the expression on the left-hand side is equal to the expression on the right-hand side.
Common Misconceptions and Clarifications
A frequent source of confusion arises from the fact that an equation contains expressions. The expressions on either side of the equals sign are what make the equation meaningful. However, the equation itself is a complete statement, whereas the expression is only a part of a larger mathematical construct. It's essential not to conflate the two.
Another common misconception is assuming that all equations contain variables. While many do, some simple equations, like 2 + 2 = 4, are purely numerical and assert a straightforward equality. This highlights the broader definition of an equation as a statement of equality between two expressions, regardless of the presence of variables.
Furthermore, expressions can be incredibly complex, involving multiple operations and nested functions. Similarly, equations can encompass intricate mathematical relationships, often requiring sophisticated techniques to solve them.
Analyzing Potential True Statements
Now, let's consider some potential statements about equations and expressions and evaluate their veracity. To illustrate, we'll consider four statements and determine which one is definitively true.
Statement 1: An expression always contains an equals sign.
This statement is false. As discussed earlier, expressions are defined by the absence of an equals sign.
Statement 2: An equation always contains at least one variable.
This statement is false. While many equations use variables, simple numerical equations, like 5 + 7 = 12, are perfectly valid equations without any variables.
Statement 3: An equation is a statement that two expressions are equal.
This statement is true. This is the defining characteristic of an equation: it establishes a relationship of equality between two mathematical expressions.
Statement 4: An expression can be simplified, but an equation cannot.
This statement is false. Both expressions and equations can be simplified. Simplifying an expression often involves combining like terms or applying the order of operations. Simplifying an equation usually involves manipulating both sides to isolate a variable or achieve a desired form.
The Importance of Understanding Equations and Expressions
A firm grasp of the distinction between equations and expressions is crucial for numerous reasons:
-
Solving Algebraic Problems: The ability to differentiate between the two is fundamental to solving algebraic equations. You need to identify the expressions involved and manipulate them to find the solution.
-
Understanding Functions: Functions are essentially equations that relate one variable to another. Understanding equations is essential to understanding functions, a cornerstone of calculus and other advanced mathematical fields.
-
Data Analysis and Interpretation: Equations are ubiquitous in analyzing data. Linear regression, for example, relies heavily on manipulating equations to model relationships between variables.
-
Programming and Computer Science: The logic behind manipulating equations and expressions is directly applicable to programming. Many programming tasks involve the evaluation of expressions and the solving of equations.
Advanced Concepts and Applications
The fundamental differences between equations and expressions lay the groundwork for more advanced mathematical concepts. Let's touch on a few:
Inequalities
Inequalities are similar to equations, but instead of an equals sign, they use symbols like < (less than), > (greater than), ≤ (less than or equal to), and ≥ (greater than or equal to). They represent a range of values rather than a single solution.
Systems of Equations
Multiple equations can be considered simultaneously to form a system of equations. Solving a system of equations involves finding the values that satisfy all the equations in the system.
Quadratic Equations and Beyond
Equations can become increasingly complex. Quadratic equations, containing squared variables, require specialized techniques like factoring or the quadratic formula to solve. Further, higher-order polynomial equations, exponential equations, logarithmic equations, and many other types of equations exist, each with its own solution methods.
Conclusion: Mastering the Fundamentals
The statement that accurately reflects the relationship between equations and expressions is: An equation is a statement that two expressions are equal. This underscores the fundamental difference: expressions represent quantities, while equations assert relationships of equality between these quantities. Developing a deep understanding of these core concepts is crucial for success in mathematics, providing a solid foundation for tackling more complex problems and advanced mathematical concepts in the future. Remember, practice is key! The more you work with equations and expressions, the more intuitive the differences will become.
Latest Posts
Latest Posts
-
Every Integer Is A Real Number
Apr 01, 2025
-
Count Vowels In A String Python
Apr 01, 2025
-
Which Of The Following Elements Is Most Electronegative
Apr 01, 2025
-
For Which Value Of X Is Abcd A Kite
Apr 01, 2025
-
64 To The Power Of 1 2
Apr 01, 2025
Related Post
Thank you for visiting our website which covers about Which Statement About Equations And Expressions Is True . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
