In The Figure Light Is Incident At Angle
News Leon
Mar 28, 2025 · 6 min read
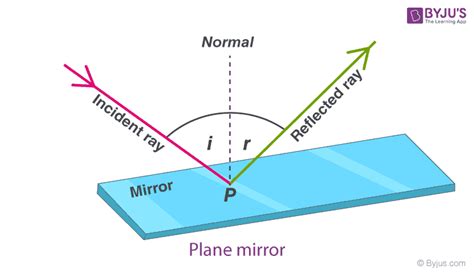
Table of Contents
In the Figure, Light is Incident at an Angle: Exploring Snell's Law and its Applications
Understanding how light behaves when it interacts with different mediums is fundamental to various fields, from designing optical instruments to understanding atmospheric phenomena. This article delves into the intricacies of light incidence at an angle, focusing primarily on Snell's Law and its diverse applications. We'll explore the concepts of refraction, reflection, and total internal reflection, providing a comprehensive overview for both beginners and those seeking a deeper understanding.
Snell's Law: The Governing Principle
When light travels from one medium to another (e.g., from air to water), it changes speed. This change in speed causes the light to bend, a phenomenon known as refraction. Snell's Law, a cornerstone of geometrical optics, mathematically describes this bending. The law states:
n₁sinθ₁ = n₂sinθ₂
Where:
- n₁ is the refractive index of the first medium.
- θ₁ is the angle of incidence (the angle between the incident ray and the normal to the surface).
- n₂ is the refractive index of the second medium.
- θ₂ is the angle of refraction (the angle between the refracted ray and the normal to the surface).
The refractive index (n) is a dimensionless number that represents the ratio of the speed of light in a vacuum to the speed of light in a given medium. A higher refractive index indicates a slower speed of light in that medium.
Understanding the Components:
-
Angle of Incidence (θ₁): This is the angle formed between the incoming light ray and a line perpendicular (normal) to the surface where the two mediums meet. It's measured from the normal.
-
Angle of Refraction (θ₂): This is the angle formed between the light ray after it passes into the second medium and the normal to the surface. It's also measured from the normal.
-
Refractive Index (n): This value is specific to each medium and depends on the medium's properties and the wavelength of the light. For example, the refractive index of air is approximately 1.0003, while the refractive index of water is approximately 1.33.
Exploring Different Scenarios:
Let's consider several scenarios illustrating the application of Snell's Law and the behavior of light at different angles of incidence.
1. Light Passing from a Rarer to a Denser Medium:
When light travels from a medium with a lower refractive index (rarer medium, like air) to a medium with a higher refractive index (denser medium, like water), the light bends towards the normal. This means θ₂ will be smaller than θ₁. The light slows down as it enters the denser medium.
Example: Imagine a ray of light passing from air (n₁ ≈ 1) into water (n₂ ≈ 1.33) at an angle of incidence of 30°. Using Snell's Law, we can calculate the angle of refraction:
(1)sin(30°) = (1.33)sin(θ₂)
Solving for θ₂, we find that the angle of refraction is approximately 22°.
2. Light Passing from a Denser to a Rarer Medium:
Conversely, when light travels from a denser medium to a rarer medium, the light bends away from the normal. This means θ₂ will be larger than θ₁. The light speeds up as it enters the rarer medium.
Example: Now let's consider light passing from water (n₁ ≈ 1.33) into air (n₂ ≈ 1) at an angle of incidence of 30°. Applying Snell's Law:
(1.33)sin(30°) = (1)sin(θ₂)
Solving for θ₂, we find that the angle of refraction is approximately 41.7°.
3. Total Internal Reflection (TIR):
A fascinating phenomenon occurs when light travels from a denser medium to a rarer medium at a specific angle called the critical angle. Beyond this critical angle, no refraction occurs; instead, all the light is reflected back into the denser medium. This is known as total internal reflection.
The critical angle (θc) can be calculated using Snell's Law:
sin(θc) = n₂/n₁
where n₁ > n₂ (light travels from denser to rarer medium).
Applications of TIR:
-
Fiber Optics: Total internal reflection is the fundamental principle behind fiber optic communication. Light signals are transmitted through long strands of glass or plastic fibers, with repeated internal reflections ensuring minimal signal loss.
-
Prisms: Prisms utilize TIR to redirect light efficiently, as seen in binoculars and periscopes.
-
Medical Imaging: Endoscopes rely on TIR to transmit images from the inside of the body, allowing for minimally invasive procedures.
Beyond Snell's Law: Other Factors Affecting Light Propagation
While Snell's Law provides a fundamental understanding of light refraction, several factors can influence light propagation in real-world scenarios:
-
Wavelength Dependence: The refractive index of a medium varies slightly with the wavelength of light. This phenomenon is known as dispersion and causes white light to separate into its constituent colors when passing through a prism.
-
Absorption and Scattering: As light travels through a medium, some of its energy can be absorbed or scattered by the medium's particles. This absorption and scattering can lead to attenuation of the light intensity.
-
Nonlinear Optics: At high light intensities, the refractive index of a medium can become dependent on the light intensity itself. This leads to nonlinear optical effects, such as second-harmonic generation and self-focusing.
Applications of Light Incidence at an Angle in Real-World Systems:
The principles discussed above are crucial to numerous technological and natural phenomena:
-
Lens Design: Lenses in cameras, telescopes, and microscopes rely on the precise control of light refraction to focus images. The shape and refractive index of the lens material are carefully chosen to manipulate the angles of incidence and refraction to achieve the desired focusing effect.
-
Optical Instruments: Spectrometers, interferometers, and polarimeters all utilize the interaction of light with different media at various angles to perform specific analytical tasks.
-
Atmospheric Optics: Refraction of sunlight in the atmosphere causes phenomena like rainbows and mirages. The angle at which sunlight enters the atmosphere and interacts with water droplets or variations in air density determines the appearance and location of these optical effects.
-
Optical Fibers: As mentioned earlier, total internal reflection in optical fibers enables long-distance data transmission with minimal signal degradation. The precise control of the angle of incidence ensures that the light remains confined within the fiber core.
-
Optical Coatings: Anti-reflective coatings on lenses and other optical surfaces utilize interference effects caused by light reflection at different interfaces to minimize unwanted reflections and maximize light transmission. The angles of incidence and the thicknesses of the coatings are carefully designed to achieve this effect.
Conclusion: A Multifaceted Phenomenon
The incidence of light at an angle, governed by Snell's Law, is a cornerstone principle in optics with far-reaching consequences. From the simple bending of light through a glass of water to the complex technology of fiber optic communications, understanding this phenomenon is essential for numerous scientific and technological advancements. The intricate interplay between the angle of incidence, refractive index, and other factors opens up a world of possibilities, leading to innovative applications that continue to shape our understanding of the world around us. Further exploration into the nuanced aspects of light interaction and its diverse applications promises even more exciting discoveries in the future. This fundamental concept continues to drive innovation across numerous fields, underscoring its importance in shaping our technological landscape. The ability to precisely control and manipulate light's behavior is crucial to advancements in areas ranging from medical imaging to high-speed communication networks.
Latest Posts
Latest Posts
-
Right Hand Rule For Angular Velocity
Mar 31, 2025
-
Read The Extract And Answer The Following Questions
Mar 31, 2025
-
Condensed Structural Formula For 1 2 Dibromoethane
Mar 31, 2025
-
What Two Factors Does Air Pressure Depend On
Mar 31, 2025
-
Which Lewis Electron Dot Diagram Is Correct For Co2
Mar 31, 2025
Related Post
Thank you for visiting our website which covers about In The Figure Light Is Incident At Angle . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
