Does Denser Medium Have Higher Refractive Index
News Leon
Mar 27, 2025 · 5 min read
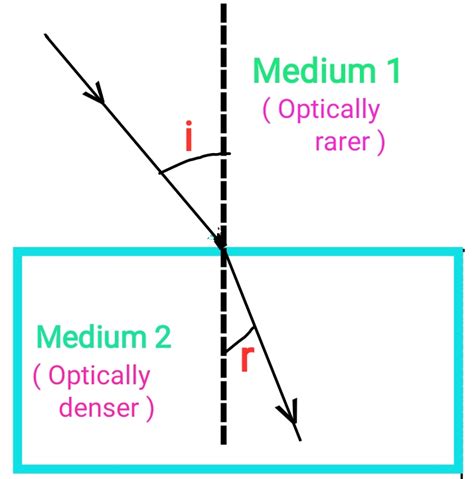
Table of Contents
Does a Denser Medium Have a Higher Refractive Index? Exploring the Relationship Between Density and Refractive Index
The relationship between a medium's density and its refractive index is a common point of confusion in physics and optics. While intuition might suggest a direct correlation—that denser materials bend light more—the reality is more nuanced. This article delves into the intricate connection between density and refractive index, exploring the underlying physics and clarifying why a simple "yes" or "no" answer isn't sufficient.
Understanding Refractive Index
Before exploring the density-refractive index relationship, let's establish a firm understanding of what refractive index actually represents. The refractive index (n) of a medium is a dimensionless number that describes how fast light propagates through that medium compared to its speed in a vacuum. Mathematically, it's defined as:
n = c/v
where:
- c is the speed of light in a vacuum (approximately 3 x 10⁸ m/s)
- v is the speed of light in the medium
A higher refractive index indicates that light travels slower in that medium. For example, the refractive index of air is approximately 1.0003, while the refractive index of water is approximately 1.33. This means light travels slower in water than in air.
The Role of Polarizability
The key to understanding the relationship between density and refractive index lies in the concept of polarizability. Polarizability refers to how easily the electrons within a material's atoms or molecules can be displaced by an electromagnetic field, such as the oscillating electric field of light. When light enters a medium, its electric field interacts with these electrons, causing them to oscillate. These oscillating electrons then re-radiate electromagnetic waves, which interfere with the incident light wave. This interference leads to a change in the speed of light, hence the refractive index.
Highly polarizable materials have electrons that are relatively easy to displace. These materials generally exhibit a higher refractive index. Conversely, materials with low polarizability have tightly bound electrons and a lower refractive index.
Density's Indirect Influence
Density, while seemingly directly related, doesn't directly determine polarizability. Density represents the mass per unit volume of a material. While a denser material generally has more electrons per unit volume, the crucial factor is how tightly those electrons are bound to their atoms or molecules.
Consider two materials:
- Material A: High density, but electrons are tightly bound to the atoms.
- Material B: Lower density, but electrons are loosely bound to the atoms.
Even though Material A is denser, Material B might have a higher refractive index because its electrons are more easily polarized. The strength of the interatomic/intermolecular forces plays a significant role here. Strong forces hold electrons tightly, reducing polarizability.
Examples Challenging the Simple Correlation
Many examples contradict the notion of a direct correlation between density and refractive index. Let's examine some:
-
Diamond vs. Water: Diamond (n ≈ 2.42) is significantly denser than water (n ≈ 1.33), yet its refractive index is much higher. This is because the electrons in diamond are more polarizable than those in water, even though water has a higher density compared to diamond.
-
Air vs. Glass: Glass (n ≈ 1.5) is denser than air, but it has a significantly higher refractive index because of the different nature of chemical bonding and stronger polarizability.
-
Ice vs. Water: Ice (n ≈ 1.31) is less dense than liquid water (n ≈ 1.33), yet it has a slightly lower refractive index. This unusual behavior is due to the structural differences between the solid and liquid phases of water. The arrangement of water molecules in ice affects the polarizability differently compared to liquid water.
Factors Influencing Refractive Index Beyond Density
Several other factors besides density significantly influence the refractive index:
-
Wavelength of Light: Refractive index is wavelength-dependent; this phenomenon is known as dispersion. Different wavelengths of light experience different refractive indices in the same medium. This is why prisms can separate white light into its constituent colors.
-
Temperature: Temperature affects the refractive index. Generally, an increase in temperature leads to a decrease in the refractive index. This is because increased temperature causes the molecules to move more rapidly, reducing their ability to interact effectively with the light's electric field.
-
Chemical Composition: The chemical composition of a material profoundly impacts its refractive index. Different molecules possess different polarizabilities, and hence, different refractive indices.
-
Crystal Structure: The arrangement of atoms or molecules within a crystal structure affects the refractive index. Anisotropic crystals exhibit different refractive indices along different crystallographic axes.
Advanced Concepts: Lorentz-Lorenz Equation
The Lorentz-Lorenz equation provides a more sophisticated relationship between refractive index, density, and polarizability:
(n² - 1) / (n² + 2) = (4π/3) N α
where:
- n is the refractive index
- N is the number density of molecules (related to density)
- α is the molecular polarizability
This equation illustrates that refractive index depends on both the number density of molecules (related to density) and the molecular polarizability. It highlights that density alone doesn't determine the refractive index; the polarizability is just as crucial, if not more so.
Conclusion: Density is Not the Sole Determinant
In conclusion, while density can influence the refractive index indirectly by affecting the number of polarizable particles per unit volume, it is not the sole determinant. The polarizability of the material's constituent atoms or molecules plays a far more critical role. A denser material may not necessarily have a higher refractive index if its electrons are tightly bound, resulting in low polarizability. The Lorentz-Lorenz equation provides a more accurate representation of the complex relationship between density, polarizability, and refractive index, demonstrating the need to consider all factors when evaluating a material's optical properties. Understanding these nuances is vital in various applications, from designing optical lenses and fibers to analyzing the behavior of light in different materials.
Latest Posts
Latest Posts
-
How Many Protons Electrons And Neutrons Are In Sodium
Mar 30, 2025
-
Mass Of Hydrogen Atom In G
Mar 30, 2025
-
Do Alkaline Earth Metals Occur Freely In Nature
Mar 30, 2025
-
Air Is Good Conductor Of Heat
Mar 30, 2025
-
Lines Of Symmetry On An Octagon
Mar 30, 2025
Related Post
Thank you for visiting our website which covers about Does Denser Medium Have Higher Refractive Index . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
