Classify The Following Triangle As Acute Obtuse Or Right
News Leon
Mar 28, 2025 · 6 min read
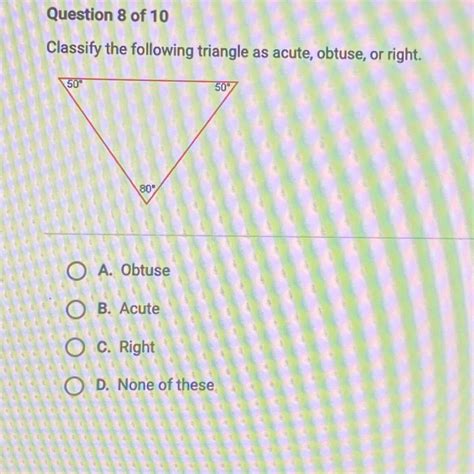
Table of Contents
Classify Triangles: Acute, Obtuse, or Right – A Comprehensive Guide
Understanding the classification of triangles is fundamental in geometry. This comprehensive guide delves into the intricacies of classifying triangles based on their angles: acute, obtuse, or right. We'll explore the defining characteristics of each type, provide practical examples, and equip you with the knowledge to confidently classify any triangle you encounter.
Understanding Triangle Angles: The Foundation
Before diving into the classifications, let's establish a crucial understanding: the sum of the interior angles of any triangle always equals 180 degrees. This fundamental principle underpins the entire process of triangle classification. Knowing this, we can determine the type of triangle simply by analyzing the measures of its angles.
Key Terminology:
- Acute Angle: An angle measuring less than 90 degrees.
- Right Angle: An angle measuring exactly 90 degrees. Represented by a small square in the corner of the angle.
- Obtuse Angle: An angle measuring more than 90 degrees but less than 180 degrees.
Classifying Triangles Based on Angles:
Now, let's examine the three classifications based on the types of angles present in the triangle:
1. Acute Triangles:
An acute triangle is a triangle where all three interior angles are acute angles – meaning each angle measures less than 90 degrees. This is a key characteristic that sets it apart from other triangle types. Because the sum of the angles must equal 180 degrees, no single angle in an acute triangle can be greater than or equal to 90 degrees.
Examples of Acute Triangles:
- A triangle with angles of 60°, 60°, and 60° (an equilateral triangle – a special case of an acute triangle).
- A triangle with angles of 45°, 65°, and 70°.
- A triangle with angles of 30°, 60°, and 90° (This is not an acute triangle, despite having one acute angle; it's a right-angled triangle)
Identifying Acute Triangles: The simplest way to identify an acute triangle is to measure each angle. If all three angles are less than 90 degrees, it's an acute triangle.
2. Obtuse Triangles:
An obtuse triangle is defined by the presence of one obtuse angle – an angle that measures greater than 90 degrees but less than 180 degrees. Only one angle can be obtuse; otherwise, the sum of the angles would exceed 180 degrees, violating the fundamental principle of triangle geometry.
Examples of Obtuse Triangles:
- A triangle with angles of 100°, 40°, and 40°.
- A triangle with angles of 120°, 30°, and 30°.
- A triangle with angles of 91°, 45°, and 44°.
Identifying Obtuse Triangles: Again, measuring the angles is the most direct approach. If one angle exceeds 90 degrees, and the others are less than 90 degrees, you've identified an obtuse triangle.
3. Right Triangles:
A right triangle is characterized by the presence of one right angle – an angle that measures exactly 90 degrees. This right angle is usually denoted by a small square drawn in the corner. Right triangles hold a special place in geometry due to the Pythagorean theorem, which relates the lengths of the sides. The side opposite the right angle is called the hypotenuse, and it's always the longest side.
Examples of Right Triangles:
- A triangle with angles of 90°, 45°, and 45°. (Isosceles right-angled triangle)
- A triangle with angles of 90°, 30°, and 60°. (30-60-90 triangle)
- A triangle where the square of the hypotenuse equals the sum of the squares of the other two sides (This is a direct application of the Pythagorean theorem).
Identifying Right Triangles: Look for the presence of the 90-degree angle indicated by the small square. Alternatively, use the Pythagorean theorem to check if the relationship a² + b² = c² holds true (where a and b are the lengths of the legs and c is the length of the hypotenuse). If the relationship is true, it's a right triangle.
Beyond the Basics: Practical Applications and Problem Solving
Classifying triangles isn't just an academic exercise; it has practical applications in various fields:
- Engineering and Architecture: Understanding triangle properties is critical in structural design, ensuring stability and strength in buildings and bridges. Right-angled triangles are particularly important for calculating distances and angles.
- Surveying and Mapping: Surveyors use trigonometry, heavily reliant on triangle properties, to determine distances and elevations for accurate land measurement.
- Computer Graphics and Game Development: Triangle classification is fundamental in creating realistic 3D models and animations.
- Navigation: Determining locations and directions often relies on calculations involving triangles and their angles.
Let's work through a few examples to solidify your understanding:
Example 1:
A triangle has angles measuring 50°, 60°, and 70°. Classify this triangle.
Solution:
Since all angles are less than 90°, this triangle is an acute triangle.
Example 2:
A triangle has angles measuring 110°, 35°, and 35°. Classify this triangle.
Solution:
The presence of an angle greater than 90° (110°) makes this triangle an obtuse triangle.
Example 3:
A triangle has sides of length 3, 4, and 5. Classify this triangle.
Solution:
We can use the Pythagorean theorem to check if this is a right triangle. 3² + 4² = 9 + 16 = 25 = 5². Since the theorem holds true, this is a right triangle.
Example 4:
A triangle has angles of 25°, 65°, and x°. Find x and classify the triangle.
Solution:
The sum of angles in a triangle is 180°. Therefore, 25° + 65° + x° = 180°. Solving for x, we get x = 90°. This means the triangle is a right triangle.
Advanced Considerations and Further Exploration:
While the basics of classifying triangles are relatively straightforward, more advanced geometrical concepts can build upon this foundation. For example, understanding the relationships between angles and sides (like the sine, cosine, and tangent functions) allows for even more precise calculations and classifications, especially in situations where only side lengths are known. Further exploration might involve studying the different types of special triangles (such as equilateral, isosceles, and scalene triangles), and how their classifications intersect.
Conclusion:
Successfully classifying triangles as acute, obtuse, or right is a vital skill in geometry and has applications across many fields. By understanding the fundamental principles of triangle angles and applying the simple steps outlined in this guide, you can confidently classify any triangle you encounter, opening doors to deeper exploration of geometric concepts and their real-world applications. Remember the key: the sum of angles always equals 180 degrees, and that's your key to unlocking the classification.
Latest Posts
Latest Posts
-
Classify The Following As A Homogeneous Or A Heterogeneous Mixture
Mar 31, 2025
-
What Physical Quantity Does The Slope Represent
Mar 31, 2025
-
An Object Becomes Positively Charged By Gaining Protons
Mar 31, 2025
-
Right Hand Rule For Angular Velocity
Mar 31, 2025
-
Read The Extract And Answer The Following Questions
Mar 31, 2025
Related Post
Thank you for visiting our website which covers about Classify The Following Triangle As Acute Obtuse Or Right . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
