2 Is The Only Even Prime Number
News Leon
Apr 02, 2025 · 5 min read
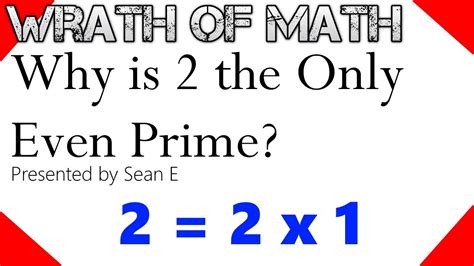
Table of Contents
2 is the Only Even Prime Number: A Deep Dive into Number Theory
The statement "2 is the only even prime number" is a fundamental concept in number theory, a branch of mathematics exploring the properties of numbers. While seemingly simple, this statement underpins much of our understanding of prime numbers and their distribution. This article will delve into the reasons behind this assertion, exploring the definitions of prime and even numbers, providing proofs, and examining the consequences of this unique property of the number 2.
Understanding Prime and Even Numbers
Before we delve into the proof, let's clearly define our terms:
Prime Number: A prime number is a natural number greater than 1 that has no positive divisors other than 1 and itself. In simpler terms, it's only divisible by 1 and itself. Examples include 2, 3, 5, 7, 11, and so on.
Even Number: An even number is an integer that is divisible by 2. This means it can be expressed as 2 * k, where k is another integer. Examples include 2, 4, 6, 8, 10, and so on.
The key distinction lies in their divisibility. Prime numbers are indivisible except by 1 and themselves, while even numbers are always divisible by 2. This seemingly small difference leads to the unique position of the number 2.
Proof: Why 2 is the Only Even Prime Number
The proof that 2 is the only even prime number is relatively straightforward, relying on the definitions above. Let's examine it step-by-step:
1. The Case of 2: The number 2 is an even number (divisible by 2) and it is only divisible by 1 and itself. Therefore, it satisfies the definition of a prime number.
2. All Other Even Numbers: Consider any other even number greater than 2. By definition, it can be expressed as 2 * k, where k is an integer greater than 1. This means it is divisible by 2 and k. Since k is greater than 1, it has at least three divisors: 1, 2, and k (and potentially more). This violates the definition of a prime number which states that a prime number only has two divisors: 1 and itself.
Therefore, by contradiction, no even number greater than 2 can be a prime number. Only 2 satisfies the conditions of being both even and prime.
The Uniqueness of 2 in Number Theory
The fact that 2 is the only even prime number has significant implications within number theory and beyond:
1. Fundamental Theorem of Arithmetic: The fundamental theorem of arithmetic states that every integer greater than 1 can be uniquely represented as a product of prime numbers. This theorem relies heavily on the distinct nature of 2. If there were multiple even prime numbers, the uniqueness of this factorization would be compromised.
2. Sieve of Eratosthenes: The Sieve of Eratosthenes is a simple algorithm for finding all prime numbers up to any given limit. It begins by eliminating multiples of 2, then 3, then 5, and so on. The initial elimination of multiples of 2 highlights the special role of 2 as the only even prime. All subsequent prime numbers are odd.
3. Distribution of Prime Numbers: The distribution of prime numbers is a complex and fascinating area of research. The prime number theorem provides an approximate estimate of the number of primes less than a given number. However, understanding the unique properties of 2, such as its evenness, can aid in refining our understanding of prime number distribution, particularly in relation to other even numbers.
4. Cryptography: Prime numbers are fundamental to many cryptographic algorithms. The unique properties of 2 are sometimes exploited in specific cryptographic techniques. While not always central, understanding its distinction as the sole even prime is important in a broader comprehension of the mathematical foundations of cryptography.
Exploring Further: Beyond the Basics
The seemingly simple statement "2 is the only even prime number" opens doors to more profound mathematical explorations:
1. Twin Primes: Twin primes are pairs of prime numbers that differ by 2 (e.g., 3 and 5, 11 and 13). The existence and distribution of twin primes are still an active area of research, and the unique properties of 2 play a role in understanding these pairs.
2. Goldbach's Conjecture: This famous unsolved conjecture states that every even integer greater than 2 can be expressed as the sum of two prime numbers. Again, the number 2 holds a special place, as it's the only even prime number and often requires special consideration in any proof related to this conjecture.
3. Mersenne Primes: Mersenne primes are prime numbers of the form 2<sup>p</sup> - 1, where p is also a prime number. The search for Mersenne primes is a significant undertaking in computational number theory, and the number 2 forms the base of this equation, highlighting its fundamental role in the definition itself.
Practical Applications and Relevance
While the concept might seem purely theoretical, the unique nature of 2 as the only even prime number has practical applications:
1. Computer Science: Algorithms in computer science often rely on prime numbers, particularly for tasks related to cryptography and data security. Understanding the properties of prime numbers, especially the unique even prime 2, allows for the optimization of algorithms and more efficient code.
2. Coding Theory: Error-correcting codes, crucial in data transmission and storage, utilize prime numbers. The unique properties of 2 influence the choice and performance of certain coding schemes.
Conclusion: A Cornerstone of Number Theory
The statement that 2 is the only even prime number is far more significant than it initially appears. This seemingly simple fact underpins many fundamental concepts in number theory, influencing our understanding of prime number distribution, the fundamental theorem of arithmetic, and various algorithms in computer science and cryptography. Its unique properties continue to be explored and contribute to ongoing research in the fascinating world of mathematics. The seemingly simple number 2, therefore, serves as a powerful cornerstone in the grand edifice of number theory, reminding us of the profound implications that can arise from seemingly elementary mathematical truths. It’s a testament to the elegant simplicity and profound depth that defines the beauty of mathematics.
Latest Posts
Latest Posts
-
Oxidation Number Of Carbon In Co
Apr 03, 2025
-
Is Friction A Non Conservative Force
Apr 03, 2025
-
Which Of The Following Graphs Represents A One To One Function
Apr 03, 2025
-
Is B2 2 Paramagnetic Or Diamagnetic
Apr 03, 2025
-
Capital Of Karnataka State In India
Apr 03, 2025
Related Post
Thank you for visiting our website which covers about 2 Is The Only Even Prime Number . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
