What Is The Reciprocal Of 1 6
News Leon
Mar 31, 2025 · 5 min read
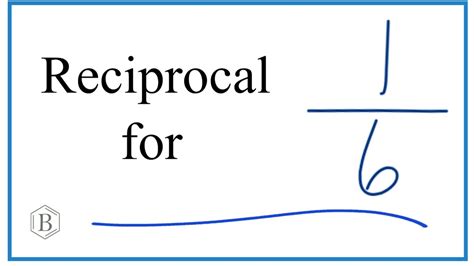
Table of Contents
What is the Reciprocal of 1/6? A Deep Dive into Mathematical Inverses
The question, "What is the reciprocal of 1/6?" might seem deceptively simple. However, understanding reciprocals goes beyond a simple calculation; it unlocks a fundamental concept in mathematics with far-reaching applications in various fields. This comprehensive guide will not only answer the initial question but also explore the broader meaning of reciprocals, their properties, and their practical uses.
Understanding Reciprocals: The Flip-Side of Numbers
A reciprocal, also known as a multiplicative inverse, is a number that, when multiplied by the original number, results in a product of 1. In simpler terms, it's the number you need to flip to get 1. This concept applies to all numbers except zero, as there is no number that, when multiplied by zero, yields 1.
Finding the Reciprocal: The process of finding a reciprocal is straightforward. For a fraction a/b, its reciprocal is simply b/a. For a whole number (which can be expressed as a fraction with a denominator of 1), its reciprocal is 1 over that number.
The Reciprocal of 1/6
Now, let's address the question directly: What is the reciprocal of 1/6?
Following the rule for fractions, we flip the numerator and denominator:
The reciprocal of 1/6 is 6/1, which simplifies to 6.
Reciprocals in Different Number Systems
The concept of reciprocals extends beyond simple fractions and whole numbers. Let's explore how reciprocals behave in various number systems:
Reciprocals of Integers
Integers are whole numbers (positive, negative, or zero). The reciprocal of a non-zero integer 'n' is simply 1/n. For example:
- The reciprocal of 5 is 1/5.
- The reciprocal of -3 is -1/3.
Reciprocals of Fractions
As previously demonstrated, the reciprocal of a fraction a/b is b/a. This holds true for all fractions, except those with a numerator of zero (which are undefined).
Reciprocals of Decimals
Decimals can be easily converted to fractions, and then their reciprocals can be found using the fraction method. For example:
- The decimal 0.5 is equivalent to the fraction 1/2. Its reciprocal is 2/1, or 2.
- The decimal 0.25 is equivalent to 1/4. Its reciprocal is 4/1, or 4.
Reciprocals of Irrational Numbers
Irrational numbers, like π (pi) and √2 (the square root of 2), are non-terminating and non-repeating decimals. Their reciprocals are also irrational numbers. For example, the reciprocal of π is approximately 1/3.14159... While we can't express these reciprocals exactly as fractions or terminating decimals, their existence is crucial in many mathematical calculations.
Properties of Reciprocals
Reciprocals possess several important properties that make them essential tools in algebra and other branches of mathematics:
- The reciprocal of a reciprocal is the original number: The reciprocal of the reciprocal of 'x' is 'x'. For example, the reciprocal of 1/6 is 6, and the reciprocal of 6 is 1/6.
- The product of a number and its reciprocal is always 1: This is the defining property of reciprocals. x * (1/x) = 1 (for x ≠ 0).
- The reciprocal of a positive number is positive, and the reciprocal of a negative number is negative: This preserves the sign of the original number.
- The reciprocal of 1 is 1: This is a special case where the number and its reciprocal are the same.
Applications of Reciprocals
The concept of reciprocals is not merely a theoretical curiosity; it plays a critical role in numerous practical applications across various fields:
Algebra and Equation Solving
Reciprocals are fundamental in solving algebraic equations. For example, when dealing with an equation like 3x = 12, we can isolate 'x' by multiplying both sides by the reciprocal of 3 (which is 1/3):
(1/3) * 3x = 12 * (1/3)
x = 4
Physics and Engineering
Reciprocals are frequently used in physics and engineering formulas. For example:
- Ohm's Law: Resistance (R) is the reciprocal of conductance (G): R = 1/G.
- Lens Optics: The focal length (f) of a lens is related to its power (P) by the reciprocal: f = 1/P.
- Frequency and Period: In physics, frequency (f) and period (T) are reciprocals: f = 1/T.
Chemistry and Other Sciences
Reciprocals appear in various chemical calculations and other scientific formulas. For example, in reaction kinetics, rate constants are often used, which can have reciprocals to describe the rate of the reverse reaction.
Computer Science and Programming
Reciprocals are used in various computational algorithms and data structures. Many mathematical operations rely heavily on the efficient calculation of reciprocals. For example, division can often be sped up by calculating the reciprocal of the divisor and then multiplying instead of dividing.
Advanced Concepts Related to Reciprocals
The exploration of reciprocals can delve deeper into more advanced mathematical concepts:
Matrices and Linear Algebra
In linear algebra, the concept of a reciprocal extends to matrices. The inverse of a matrix is a matrix that, when multiplied by the original matrix, results in the identity matrix (a matrix with 1s along the diagonal and 0s elsewhere). Finding matrix inverses is crucial in solving systems of linear equations.
Complex Numbers
The concept of reciprocals applies to complex numbers as well. The reciprocal of a complex number a + bi is found by multiplying both the numerator and denominator by the complex conjugate of the denominator (a - bi). This process eliminates the imaginary component in the denominator.
Group Theory
In abstract algebra, the concept of reciprocals extends to groups. A group is a set with an operation that satisfies certain properties (closure, associativity, identity element, and inverses). The reciprocal in a group is the element that, when combined with the original element using the group operation, results in the identity element.
Conclusion
The reciprocal of 1/6 is 6. While this initial answer seems straightforward, delving into the concept of reciprocals reveals a rich and multifaceted mathematical concept with significant applications across various scientific and technical disciplines. Understanding reciprocals is not just about performing a simple calculation; it's about grasping a fundamental mathematical principle that underpins many complex equations and algorithms. From basic algebra to advanced linear algebra and beyond, the concept of the reciprocal continues to be a vital component in our understanding and application of mathematics. This detailed exploration hopefully clarifies the concept, expands your understanding, and encourages further exploration of its diverse applications.
Latest Posts
Latest Posts
-
Which One Of The Following Is An Igneous Rock
Apr 02, 2025
-
Which Is Greater 2 3 Or 3 5
Apr 02, 2025
-
Find The Area Of A Shaded Triangle
Apr 02, 2025
-
Which Of The Following Would Decrease Glomerular Filtration Rate
Apr 02, 2025
-
The Slope Of Speed Time Graph Indicates
Apr 02, 2025
Related Post
Thank you for visiting our website which covers about What Is The Reciprocal Of 1 6 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
