The Product Of Two Irrational Numbers Is Always Irrational
News Leon
Apr 02, 2025 · 5 min read
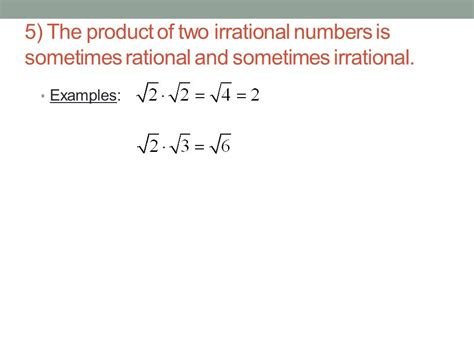
Table of Contents
Is the Product of Two Irrational Numbers Always Irrational? A Deep Dive
The statement "the product of two irrational numbers is always irrational" is false. While it might seem intuitive that multiplying two numbers without a clear pattern (irrational numbers) would always yield another number without a clear pattern, this isn't the case. This article will explore this fascinating mathematical concept, demonstrating why the statement is incorrect and providing examples and counterexamples to fully illuminate the topic. We will delve into the definitions of rational and irrational numbers, explore different types of irrational numbers, and examine the implications of their multiplication.
Understanding Rational and Irrational Numbers
Before diving into the complexities of multiplying irrational numbers, let's solidify our understanding of the fundamental definitions:
-
Rational Numbers: These are numbers that can be expressed as a fraction p/q, where 'p' and 'q' are integers, and 'q' is not zero. Examples include 1/2, -3, 0, and 0.75 (which can be expressed as 3/4). Rational numbers have either terminating or repeating decimal representations.
-
Irrational Numbers: These numbers cannot be expressed as a fraction of two integers. Their decimal representations are non-terminating and non-repeating. Famous examples include π (pi), approximately 3.14159..., e (Euler's number), approximately 2.71828..., and the square root of 2 (√2), approximately 1.41421...
The set of real numbers encompasses both rational and irrational numbers. The combination of these two sets forms the complete number line.
Counterexamples: Proving the Statement False
The crux of this article lies in presenting counterexamples that disprove the initial statement. The simplest and most effective way to demonstrate that the product of two irrational numbers isn't always irrational is to provide examples where the product is, in fact, rational.
Consider the following:
Let's take √2 as our first irrational number. This number is well-known for its non-repeating, non-terminating decimal representation. Now, let's consider another irrational number: √2.
When we multiply these two together:
√2 * √2 = 2
The result, 2, is a rational number. It can be expressed as the fraction 2/1. This single counterexample is sufficient to definitively prove that the product of two irrational numbers is not always irrational.
Let's explore another example:
Consider the irrational number x = 2 + √3 and the irrational number y = 2 - √3.
Multiplying x and y:
(2 + √3)(2 - √3) = 2² - (√3)² = 4 - 3 = 1
Again, the product (1) is a rational number. This further reinforces the fact that the statement is false.
Exploring Different Types of Irrational Numbers
The behavior of irrational numbers when multiplied depends heavily on their nature. While the above examples highlight simple cases, it's crucial to understand that there are various categories of irrational numbers, each with unique characteristics. These categories aren't rigorously defined in elementary mathematics but can help understand the complexities.
For example, algebraic irrational numbers are those that are roots of polynomial equations with integer coefficients. Transcendental irrational numbers are those that are not roots of any polynomial equation with integer coefficients. Numbers like π and e fall into this category, and they present interesting challenges when considering their products.
However, even with transcendental numbers, the initial statement isn't universally true. Although finding such counterexamples might require more complex calculations, it is still possible to construct scenarios where the product of two transcendental irrational numbers results in a rational number. The mathematical proof of such instances might involve advanced calculus or number theory concepts.
The Significance of Mathematical Proof
The examples above provide concrete demonstrations that disprove the original statement. However, the beauty and strength of mathematics lie in rigorous proof. We have shown a counterexample to the statement, effectively disproving it, demonstrating that a single counterexample is enough to invalidate a universal claim.
It's crucial to understand the difference between a conjecture (an educated guess) and a proven theorem. The statement "the product of two irrational numbers is always irrational" was a conjecture, which, as we've seen, has been proven false. To establish a mathematical truth, we require a robust proof—something beyond just a few illustrative examples. The counterexamples serve as a powerful tool in refuting the initial conjecture.
Further Exploration: When is the Product Irrational?
While the product of two irrational numbers is not always irrational, there are certainly instances where it is. Exploring the conditions under which the product remains irrational would require a more nuanced approach. This would involve deeper mathematical analysis and may necessitate considering the specific properties of the irrational numbers involved. For instance, multiplying two irrational numbers that are algebraically independent may yield an irrational result. However, a universal statement defining all such cases is beyond the scope of this article, requiring considerably more advanced mathematical knowledge.
Implications and Conclusion
The exploration of this seemingly simple statement reveals the intricacies of mathematical reasoning and the importance of rigorous proof. The fact that the product of two irrational numbers is not always irrational demonstrates the surprising complexities inherent within seemingly straightforward mathematical concepts. It highlights the need for careful consideration and the use of counterexamples to disprove universal claims.
This article has demonstrated, through simple and clear examples, that the initial statement is false. The mathematical world is full of such seemingly counterintuitive results, emphasizing the continuous need for critical thinking, rigorous analysis, and a willingness to question assumptions. Further exploration of this topic could lead to deeper insights into number theory and the fascinating properties of irrational numbers. Understanding such concepts helps in building a strong foundation in mathematics and appreciating the nuances of mathematical reasoning.
Latest Posts
Latest Posts
-
Find The Value Of P
Apr 03, 2025
-
What Is The First Step In The Boot Process
Apr 03, 2025
-
How Many Atoms In 0 075 Mol Of Titanium
Apr 03, 2025
-
What Is The Worlds Largest Cell
Apr 03, 2025
-
How Many Turns Of The Krebs Cycle Per Glucose
Apr 03, 2025
Related Post
Thank you for visiting our website which covers about The Product Of Two Irrational Numbers Is Always Irrational . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
