The Coil In The Figure Carries Current
News Leon
Mar 28, 2025 · 6 min read
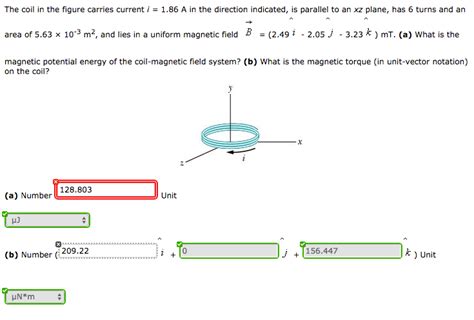
Table of Contents
The Coil in the Figure Carries Current: Exploring Electromagnetic Principles
Understanding how current flowing through a coil generates a magnetic field is fundamental to numerous applications in physics and engineering. This article delves deep into the principles governing this phenomenon, exploring the factors influencing the magnetic field's strength, direction, and applications. We’ll examine the concept of inductance, explore different coil configurations, and discuss real-world examples of coils carrying current.
Understanding the Basics: Current, Coils, and Magnetic Fields
When an electric current flows through a conductor, it generates a magnetic field around it. This is a fundamental principle of electromagnetism, described by Ampère's Law. A simple straight wire carrying current produces a circular magnetic field around it. However, by shaping the conductor into a coil (also known as a solenoid or inductor), we significantly enhance the magnetic field strength and create a more focused, uniform field within the coil’s core.
The Role of the Coil
A coil consists of multiple loops of wire wound tightly together. The magnetic fields produced by each individual loop add up constructively, creating a much stronger net magnetic field than a single loop could produce. This cumulative effect is what makes coils so useful in various applications. The magnetic field lines generated by each loop combine to form a nearly uniform field inside the coil, while the field lines outside the coil are relatively weaker and more complex.
Factors Affecting Magnetic Field Strength
Several factors influence the strength of the magnetic field produced by a coil carrying current:
-
Current (I): The magnetic field strength is directly proportional to the current flowing through the coil. A higher current produces a stronger magnetic field. This relationship is expressed mathematically in many equations related to electromagnetism.
-
Number of Turns (N): The more turns the coil has, the stronger the magnetic field. Each turn contributes to the overall magnetic field, leading to a cumulative effect.
-
Coil Length (l): For a solenoid (a long coil), the magnetic field strength is inversely proportional to the length of the coil. A shorter coil with the same number of turns will generate a stronger magnetic field within its core compared to a longer coil.
-
Permeability of the Core Material (μ): The material inside the coil (the core) significantly impacts the magnetic field strength. Materials with high permeability, such as iron, significantly amplify the magnetic field compared to air or vacuum (which have permeability close to 1). This is why many coils incorporate ferromagnetic cores.
Mathematical Representation: Biot-Savart Law and Ampère's Law
The magnetic field generated by a coil carrying current can be mathematically described using Biot-Savart Law and Ampère's Law. While Biot-Savart Law offers a more general approach for calculating the magnetic field due to a current element, Ampère's Law provides a simpler way to calculate the magnetic field inside a long solenoid.
Biot-Savart Law: A General Approach
Biot-Savart Law calculates the magnetic field contribution from an infinitesimally small current element. To determine the total magnetic field from a coil, one needs to integrate the contributions from all the current elements along the coil. This is a complex calculation, often requiring sophisticated mathematical techniques. However, this law is fundamental in understanding the behavior of magnetic fields produced by current-carrying conductors of any shape.
Ampère's Law: Simplifying the Calculation for Solenoids
For a long solenoid (a coil with a length significantly greater than its diameter), Ampère's Law offers a simplified approach. It states that the line integral of the magnetic field around a closed loop is proportional to the current enclosed by the loop. This allows for a straightforward calculation of the magnetic field inside a long solenoid, yielding a relatively uniform magnetic field. The mathematical expression is:
B = μ₀NI/l
Where:
- B is the magnetic field strength inside the solenoid
- μ₀ is the permeability of free space (a constant)
- N is the number of turns in the coil
- I is the current flowing through the coil
- l is the length of the solenoid
Inductance: The Coil's Resistance to Change
When a current flows through a coil, it generates a magnetic field. Changing this current induces a voltage in the coil itself, opposing the change in current. This property is called inductance (L), measured in Henries (H). The inductance of a coil depends on its physical characteristics, such as the number of turns, the coil's geometry, and the permeability of the core material. A larger inductance means the coil offers greater resistance to changes in current.
Self-Inductance and Mutual Inductance
-
Self-Inductance: This describes the coil's ability to oppose changes in its own current. A higher self-inductance means a greater opposition to current fluctuations.
-
Mutual Inductance: This arises when two or more coils are placed near each other. A changing current in one coil induces a voltage in the other coil, due to the interaction of their magnetic fields. This is the principle behind transformers.
Applications of Coils Carrying Current
Coils carrying current find widespread applications in various fields:
Electromagnets: Harnessing Magnetic Force
Electromagnets utilize the magnetic field generated by a current-carrying coil to create powerful, controllable magnetic forces. These are used in numerous applications:
-
Electric Motors: Electromagnets are essential components in electric motors, generating the rotating magnetic field that drives the motor's rotation.
-
Magnetic Relays: Relays use electromagnets to switch electrical circuits on and off remotely.
-
Magnetic Levitation (Maglev) Trains: These trains utilize powerful electromagnets for both propulsion and levitation.
-
Lifting Magnets: These are used in various industrial settings to lift and move heavy metallic objects.
Inductors in Electrical Circuits: Controlling Current Flow
Inductors play a crucial role in electrical circuits, primarily for controlling and shaping the flow of alternating current (AC). Their ability to store energy in their magnetic fields makes them essential components in:
-
Filters: Inductors are used in various filter circuits to selectively allow or block certain frequencies.
-
Resonant Circuits: They are used in resonant circuits, such as those found in radios and other electronic devices, to select specific frequencies.
-
Power Supplies: Inductors smooth out fluctuating DC voltages in power supplies.
-
Energy Storage: While not their primary function, inductors can store energy in their magnetic fields, making them useful in some energy storage systems.
Transformers: Efficient Energy Transmission
Transformers utilize the principle of mutual inductance to efficiently step up or step down voltage in AC circuits. They consist of two or more coils wound around a common core, allowing for efficient energy transfer between different voltage levels. Transformers are crucial for long-distance electricity transmission and in various electronic devices.
Other Applications
-
Magnetic Resonance Imaging (MRI): Powerful electromagnets are used to generate the strong magnetic fields necessary for MRI scans.
-
Wireless Charging: Inductive coupling, based on mutual inductance, is used in wireless charging technology.
-
Loudspeakers: The movement of a coil within a magnetic field is the basis of loudspeaker operation, converting electrical signals into sound.
Conclusion: A Deep Dive into the World of Coils
The humble coil, seemingly simple in its construction, plays a pivotal role in a vast array of technologies. Understanding the principles governing the magnetic field generated by a current-carrying coil is crucial for grasping the workings of many essential devices and systems. From the powerful electromagnets used in industrial applications to the tiny inductors found in electronic circuits, the coil's contribution to modern technology is undeniable. This article has attempted to provide a comprehensive overview of this fundamental component, covering its underlying principles, mathematical description, and wide-ranging applications. Further exploration into specific applications would reveal even more intricate details and fascinating aspects of this critical element in the world of electromagnetism.
Latest Posts
Latest Posts
-
3x 2y 12 In Slope Intercept Form
Mar 31, 2025
-
Which Type Of Muscle Tissue Is Multinucleated
Mar 31, 2025
-
True Or False Evaporation Is A Physical Change
Mar 31, 2025
-
Do Gram Positive Bacteria Have Porins
Mar 31, 2025
-
Which Of The Following Compounds Is Most Soluble In Water
Mar 31, 2025
Related Post
Thank you for visiting our website which covers about The Coil In The Figure Carries Current . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
