In Uniform Circular Motion Which Of The Following Is Constant
News Leon
Mar 30, 2025 · 5 min read
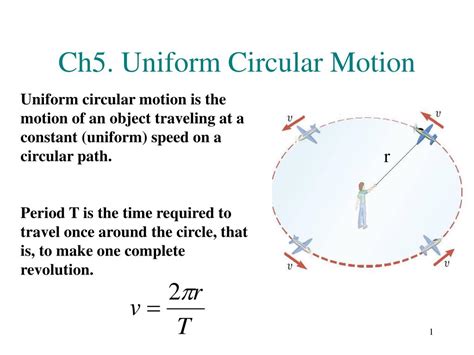
Table of Contents
In Uniform Circular Motion, Which of the Following is Constant?
Uniform circular motion is a fascinating concept in physics, describing the movement of an object traversing a circular path at a constant speed. While the speed remains unchanged, several other quantities undergo continuous alterations. This article will delve into the specifics of uniform circular motion, highlighting which quantities remain constant and which are subject to change, accompanied by detailed explanations and illustrative examples.
Understanding Uniform Circular Motion
Before we identify the constant quantity, let's solidify our understanding of uniform circular motion. It's crucial to distinguish between speed and velocity. Speed is a scalar quantity representing the rate of change of distance, while velocity is a vector quantity encompassing both speed and direction.
In uniform circular motion:
- Speed is constant: The object covers equal distances in equal intervals of time.
- Velocity is constantly changing: Although the speed is constant, the direction of motion is constantly changing, resulting in a continuously changing velocity. This change in velocity signifies an acceleration, even though the speed remains the same.
The Constant in Uniform Circular Motion: Speed
The only quantity that remains truly constant in uniform circular motion is speed. This is the fundamental characteristic defining this type of motion. No matter where the object is on the circular path, its speed remains the same.
Example: Imagine a car driving around a perfectly circular racetrack at a constant 60 mph. The car's speed is consistently 60 mph throughout the entire lap.
Quantities that Change in Uniform Circular Motion:
Several other physical quantities undergo constant change during uniform circular motion:
1. Velocity:
As mentioned earlier, velocity is a vector quantity comprising both speed and direction. In uniform circular motion, the direction of the object is constantly changing, thus, its velocity is also constantly changing. This change in velocity indicates the presence of acceleration, known as centripetal acceleration.
Example: Continuing with the racetrack example, even though the car's speed is 60 mph, its velocity is constantly changing because its direction is always changing as it moves around the track.
2. Acceleration:
The continuous change in velocity results in a constant acceleration known as centripetal acceleration. This acceleration is always directed towards the center of the circular path. It's crucial to understand that while the object's speed isn't changing, its velocity is, hence the acceleration. The magnitude of centripetal acceleration (a<sub>c</sub>) is given by:
a<sub>c</sub> = v²/r
where:
- v is the speed of the object
- r is the radius of the circular path
This formula reveals that centripetal acceleration is directly proportional to the square of the speed and inversely proportional to the radius of the circular path. A larger speed or a smaller radius results in greater centripetal acceleration.
Example: A car traveling at a higher speed (e.g., 80 mph) around the same racetrack will experience a larger centripetal acceleration than when traveling at 60 mph. Similarly, a car traveling at the same speed around a smaller, tighter track will also experience greater centripetal acceleration.
3. Angular Velocity:
Angular velocity (ω) is a measure of how quickly an object rotates around a central point. It's usually measured in radians per second (rad/s). While the speed remains constant, the angular velocity in uniform circular motion is also constant.
ω = v/r
This shows a direct relationship between angular velocity and linear speed; the faster the object moves, the greater its angular velocity. The constancy of angular velocity reflects the constant rate of rotation.
4. Displacement:
Displacement is a vector quantity representing the shortest distance between the object's initial and final positions. In uniform circular motion, the displacement is constantly changing. After one complete revolution, the displacement is zero because the object returns to its starting point. At any point during the motion, the displacement is a vector pointing from the initial position to the current position.
Example: If we consider the car completing one lap, its displacement is zero despite a considerable distance covered.
5. Force:
To maintain uniform circular motion, a net force, known as centripetal force, must act on the object. This force is always directed towards the center of the circular path. The magnitude of the centripetal force (F<sub>c</sub>) is given by:
F<sub>c</sub> = mv²/r = mω²r
where:
- m is the mass of the object
This means the centripetal force is directly proportional to the mass and the square of the speed, and inversely proportional to the radius. The centripetal force provides the continuous change in direction necessary for circular motion.
Example: The centripetal force acting on the car on the racetrack is provided by friction between the tires and the road surface.
Differentiating between Uniform Circular Motion and Non-Uniform Circular Motion
It’s essential to understand that the discussion above applies only to uniform circular motion. In non-uniform circular motion, both speed and velocity are changing, leading to tangential acceleration in addition to centripetal acceleration. In such cases, neither speed nor velocity remains constant. The concepts of centripetal force and acceleration still apply, but the calculations become more complex.
Practical Applications of Uniform Circular Motion
Understanding uniform circular motion is crucial in various fields:
-
Satellite Orbits: Satellites orbiting Earth experience uniform circular motion (approximately). The centripetal force is provided by Earth's gravitational pull.
-
Roller Coasters: The design of roller coaster loops utilizes the principles of uniform circular motion and centripetal force to create the thrilling experience.
-
Rotating Machinery: Many mechanical systems involve rotating components such as wheels, gears, and turbines. Understanding uniform circular motion helps in designing and analyzing these systems.
-
Circular Particle Accelerators: Particle accelerators utilize magnetic fields to create centripetal forces that accelerate charged particles in circular paths.
-
Circular Motion in Sports: Various sports involve circular motion, such as the swing of a baseball bat or the curve of a thrown ball.
Conclusion
In uniform circular motion, only the speed remains constant. While the object moves at a constant speed, its velocity, acceleration, displacement, and the centripetal force acting upon it are continuously changing. This seemingly simple concept has profound implications across many branches of physics and engineering, highlighting the importance of understanding its fundamental principles. Mastering the concepts of uniform circular motion opens doors to grasping more complex dynamic systems. Remember to distinguish it from non-uniform circular motion where both speed and velocity change, adding further complexity.
Latest Posts
Latest Posts
-
How To Find Density Of Air
Apr 01, 2025
-
Which Of The Following Are Disaccharides
Apr 01, 2025
-
Which Of The Following Statements About Carbon Are True
Apr 01, 2025
-
13 Out Of 20 Is What Percent
Apr 01, 2025
-
Chemical Energy To Electrical Energy Examples
Apr 01, 2025
Related Post
Thank you for visiting our website which covers about In Uniform Circular Motion Which Of The Following Is Constant . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
