In The Figure Four Long Straight Wires Are Perpendicular
News Leon
Mar 26, 2025 · 6 min read
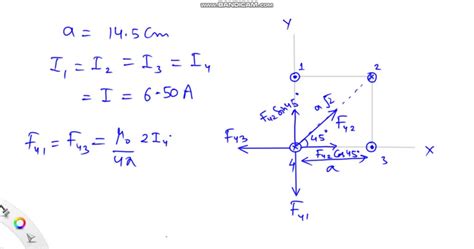
Table of Contents
In the Figure, Four Long Straight Wires are Perpendicular: Exploring Magnetic Fields and Forces
This article delves into the fascinating world of electromagnetism, specifically focusing on the magnetic fields and forces generated by four long, straight, perpendicular wires carrying currents. We'll explore the principles governing these interactions, calculate the magnetic field strength at various points, and analyze the forces exerted on each wire. Understanding this scenario is crucial for comprehending more complex electromagnetic systems found in various applications, from electric motors to advanced scientific instruments.
Understanding the Basics: Magnetic Fields from Straight Wires
Before diving into the four-wire configuration, let's review the fundamental principles of magnetic fields generated by straight current-carrying wires. A current-carrying wire creates a magnetic field around itself, forming concentric circles. The direction of this magnetic field can be determined using the right-hand rule: if you point your thumb in the direction of the current flow, your curled fingers indicate the direction of the magnetic field lines.
The magnitude of the magnetic field (B) at a distance (r) from a long, straight wire carrying a current (I) is given by Ampere's Law:
B = (μ₀I)/(2πr)
where μ₀ is the permeability of free space (a constant). This equation is crucial for calculating the magnetic field contribution from each individual wire in our four-wire system.
The Four-Wire Configuration: A Detailed Analysis
Now let's consider the scenario presented: four long, straight wires arranged perpendicularly to each other. For the sake of clarity, let's assume the wires are positioned along the x, y, and z axes of a three-dimensional Cartesian coordinate system. Each wire carries a current (I), but we’ll consider different scenarios involving the direction of the current to explore the different effects.
Scenario 1: Currents in the Same Direction
If all four wires carry currents in the same direction (e.g., all pointing upwards along the z-axis), the magnetic fields generated by each wire will interact. The net magnetic field at any point in space will be the vector sum of the individual magnetic fields. At points equidistant from multiple wires, calculating this sum becomes crucial. For example, finding the total magnetic field at the origin (0,0,0) which lies directly in the center would require considering the contribution of each wire's field. The closer the point is to a wire, the larger that wire’s contribution.
Calculating the Magnetic Field: This would involve using the formula given above for each wire and then applying vector addition to find the resultant field. Because the wires are perpendicular and positioned on Cartesian axes, this process involves significant vector calculations, often best addressed using computer software capable of handling vector algebra.
Scenario 2: Currents in Opposite Directions
If we vary the current direction in some of the wires, the situation gets more complex and interesting. Let's assume two wires carry currents in one direction (e.g., upwards), while the other two carry currents in the opposite direction (e.g., downwards). The magnetic fields will now interact in a way that could potentially lead to cancellation in certain regions. This would affect the resultant field strength and the overall force dynamics between the wires.
Calculating the Magnetic Field and Forces: In this case, calculating the net magnetic field involves similar vector addition, however, the components would either add or subtract based on the direction of each current and the location of the calculation point. Moreover, the forces between the wires are now equally important. The interaction between two current-carrying wires results in a force that is either attractive (currents flowing in the same direction) or repulsive (currents flowing in opposite directions). The force is calculated using the following formula:
F = (μ₀I₁I₂L)/(2πd)
where I₁ and I₂ are the currents in the two wires, L is the length of the wires considered, and d is the distance between them. We would need to calculate the force between each pair of wires and apply vector addition based on the direction of each force.
Scenario 3: Varying Current Magnitudes
We can make the model more realistic by considering that the currents in each of the four wires could have different magnitudes. This variation introduces additional complexity as the magnitude of each individual magnetic field will change accordingly. The resultant magnetic field would be a complex vector sum of the fields generated by each wire, weighted by its respective current magnitude.
Calculating the Magnetic Field and Forces: In this case, the calculations remain similar in principle, but we incorporate the different current values directly into the magnetic field and force equations. This would directly change the strength of the magnetic field around each wire, further impacting the net magnetic field and the attractive/repulsive forces.
Applications and Real-World Implications
Understanding the principles governing the magnetic fields and forces in this four-wire configuration has practical significance in various areas:
-
Electric Motors and Generators: The principles explored here are fundamental to the functioning of electric motors and generators. These devices rely on the interaction of magnetic fields produced by current-carrying wires to create rotational motion (motors) or generate electricity (generators).
-
Electromagnetic Shielding: The manipulation of magnetic fields through strategically placed wires can be used to create shielding against unwanted electromagnetic interference.
-
Scientific Instrumentation: Precise control over magnetic fields is crucial in many scientific instruments, such as mass spectrometers and particle accelerators.
-
Circuit Design: Understanding the interaction of current-carrying wires is essential for designing efficient and reliable electronic circuits, ensuring minimal interference between different components.
Advanced Considerations: Beyond the Basics
The analysis presented above makes certain simplifying assumptions, such as infinitely long wires. In reality, wires have finite lengths, and this will affect the magnetic field distribution. Moreover, we haven't considered the effects of the magnetic field on the wires themselves. In reality, the wires will experience a force, which might lead to changes in their physical position. Further, the model could become even more complex if the wires were not perfectly perpendicular or if they were placed in a material that is not free space.
Conclusion
Analyzing the magnetic fields and forces generated by four perpendicular, current-carrying wires provides a rich learning experience in electromagnetism. By applying fundamental principles like Ampere's Law and the right-hand rule, we can understand how individual magnetic fields combine to create a net field and how the resulting forces between wires influence their behavior. Exploring variations in current directions and magnitudes adds layers of complexity and highlights the importance of vector analysis in solving such problems. The principles derived from this simple yet insightful model have broad applications in various fields, emphasizing the significance of mastering the basics of electromagnetism. Further exploration into the complexities of finite wire lengths and material effects would make this model even more comprehensive and applicable to real-world scenarios.
Latest Posts
Latest Posts
-
What Is The Molar Mass Of Cucl2
Mar 29, 2025
-
Density A Physical Or Chemical Property
Mar 29, 2025
-
Explain How Mining Positively And Negatively Impacts Local Communities
Mar 29, 2025
-
Is Water A Reactant Or Product
Mar 29, 2025
-
How Many Thousands Make A Lakh
Mar 29, 2025
Related Post
Thank you for visiting our website which covers about In The Figure Four Long Straight Wires Are Perpendicular . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
