In The Figure A Solid Cylinder Of Radius
News Leon
Mar 26, 2025 · 6 min read
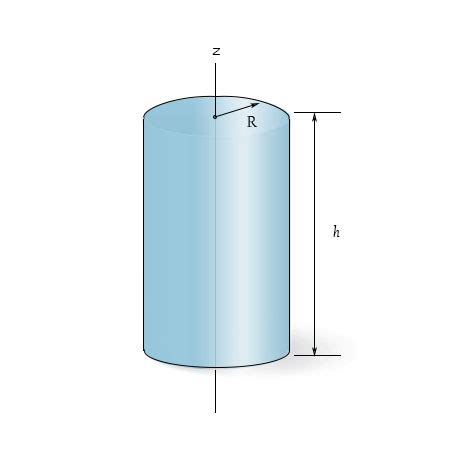
Table of Contents
Delving Deep into the Mechanics of a Solid Cylinder: Analysis and Applications
The humble solid cylinder, a three-dimensional shape characterized by its uniform circular cross-section and constant length, might seem deceptively simple. However, a deeper examination reveals a wealth of fascinating mechanical properties and diverse applications across various engineering disciplines. This article will explore the fundamental characteristics of a solid cylinder, analyze its behavior under different loading conditions, and delve into its significance in various real-world scenarios. We'll cover topics ranging from stress and strain distribution to buckling and torsional behavior, providing a comprehensive overview for both students and professionals.
Understanding the Geometry and Material Properties
Before we delve into the mechanical aspects, it's crucial to establish a clear understanding of the cylinder's geometry and the material properties that significantly influence its behavior.
-
Radius (r): The radius defines the circular cross-section's distance from the center to its edge. It's a fundamental parameter in calculating the cylinder's area, volume, and various mechanical properties.
-
Length (l): The length dictates the overall size of the cylinder. Along with the radius, it determines the cylinder's volume and plays a critical role in buckling and bending calculations.
-
Material Properties: The material from which the cylinder is constructed significantly impacts its response to external forces. Key properties include:
- Young's Modulus (E): This measures the material's stiffness or resistance to elastic deformation under tensile or compressive stress. A higher Young's modulus indicates a stiffer material.
- Poisson's Ratio (ν): This ratio describes the material's tendency to deform in one direction when stressed in another. For example, a material under tensile stress will typically contract laterally.
- Shear Modulus (G): This measures the material's resistance to shear deformation, which is a change in shape without a change in volume.
- Yield Strength (σ<sub>y</sub>): This is the stress at which the material begins to deform plastically (permanently).
- Ultimate Tensile Strength (σ<sub>u</sub>): This represents the maximum stress the material can withstand before failure.
Stress and Strain Analysis under Axial Loading
When a solid cylinder is subjected to an axial load (force applied along its longitudinal axis), it experiences both stress and strain.
-
Axial Stress (σ): This is the force (F) per unit area (A) acting on the cross-section of the cylinder. The formula is: σ = F/A = F/(πr²)
-
Axial Strain (ε): This is the change in length (Δl) divided by the original length (l). It represents the relative deformation of the cylinder under the applied load. ε = Δl/l
-
Hooke's Law: For elastic materials within their elastic limit, stress and strain are proportionally related through Hooke's Law: σ = Eε. This equation allows us to predict the cylinder's deformation under an applied load.
Stress and Strain under Torsional Loading
Applying a twisting moment (torque) to a solid cylinder induces torsional stress and strain.
-
Torsional Shear Stress (τ): This is the shear stress acting on the cross-section of the cylinder. The maximum shear stress occurs at the outer radius and is given by: τ<sub>max</sub> = (Tr)/J, where T is the applied torque and J is the polar moment of inertia (J = πr⁴/2 for a solid cylinder).
-
Torsional Shear Strain (γ): This represents the angular deformation of the cylinder due to the applied torque. It's related to the shear stress through the shear modulus: τ = Gγ.
-
Angle of Twist (θ): This is the total angular deformation of the cylinder's end under the applied torque. It's calculated using the formula: θ = (Tl)/(GJ).
Bending and Buckling Behavior
Cylinders are often subjected to bending moments, leading to bending stresses and potential buckling.
-
Bending Stress (σ<sub>b</sub>): This stress arises from the bending moment (M) and is dependent on the distance from the neutral axis (y) of the cylinder's cross-section. The bending stress is calculated using the flexure formula: σ<sub>b</sub> = My/I, where I is the area moment of inertia (I = πr⁴/4 for a solid cylinder).
-
Buckling: When a slender cylinder is subjected to compressive axial load, it may buckle, exhibiting a sudden lateral deformation. Euler's formula provides a critical load (P<sub>cr</sub>) at which buckling may occur: P<sub>cr</sub> = (π²EI)/(kl²), where k is the effective length factor, dependent on the end conditions of the cylinder.
Applications of Solid Cylinders
The versatility of solid cylinders makes them indispensable in numerous engineering applications:
-
Structural Components: Cylindrical columns, shafts, and rods are commonly used in structures due to their high strength-to-weight ratio and ability to withstand axial, torsional, and bending loads. Examples include pillars in buildings, shafts in machinery, and supporting elements in bridges.
-
Pressure Vessels: Cylinders are extensively used to contain fluids under pressure, such as pipes, tanks, and boilers. The cylinder's ability to withstand hoop stresses (circumferential stresses) is crucial in these applications.
-
Rolling Elements: Cylindrical rollers are used in bearings and other mechanisms to reduce friction and facilitate smooth motion.
-
Mechanical Parts: Solid cylinders serve as fundamental components in various mechanical devices, including gears, pistons, and shafts.
-
Aerospace Engineering: Cylindrical components are frequently employed in aircraft and spacecraft structures, due to their lightweight yet strong characteristics.
Advanced Considerations
This overview has provided a fundamental understanding of solid cylinder mechanics. However, many factors can complicate the analysis in real-world scenarios. These include:
-
Residual Stresses: Manufacturing processes can induce internal stresses within the cylinder, affecting its overall behavior.
-
Material Non-linearity: Beyond the elastic limit, the material's behavior becomes non-linear, making stress-strain relationships more complex.
-
Fatigue: Repeated loading can lead to fatigue failure, even if the stresses are below the yield strength.
-
Creep: At high temperatures, materials can deform slowly over time under sustained stress, a phenomenon known as creep.
-
Impact Loading: Sudden impact loads can cause significantly higher stresses than static loads.
Conclusion
The analysis of a solid cylinder’s mechanical behavior, although seemingly straightforward at first glance, reveals a complex interplay between geometry, material properties, and loading conditions. Understanding these interactions is crucial for engineers designing structures and machines that utilize this ubiquitous shape. From the simple yet elegant design of a cylindrical column supporting a building to the complex stress analysis of a pressure vessel containing high-pressure fluids, the solid cylinder remains a fundamental element in numerous engineering applications. Further exploration into advanced concepts like finite element analysis (FEA) is necessary for detailed simulations and accurate predictions of behavior under complex loading conditions and non-linear material responses. This detailed analysis highlights the importance of considering these factors for safe and efficient design. The principles discussed here offer a robust foundation for tackling more sophisticated problems related to cylindrical components and systems.
Latest Posts
Latest Posts
-
Is Water A Reactant Or Product
Mar 29, 2025
-
How Many Thousands Make A Lakh
Mar 29, 2025
-
A Slumber Did My Spirit Seal
Mar 29, 2025
-
Letters With A Line Of Symmetry
Mar 29, 2025
-
Marginal Product And Average Product Graph
Mar 29, 2025
Related Post
Thank you for visiting our website which covers about In The Figure A Solid Cylinder Of Radius . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
