In The Figure A Plastic Rod Having A Uniformly
News Leon
Mar 28, 2025 · 6 min read
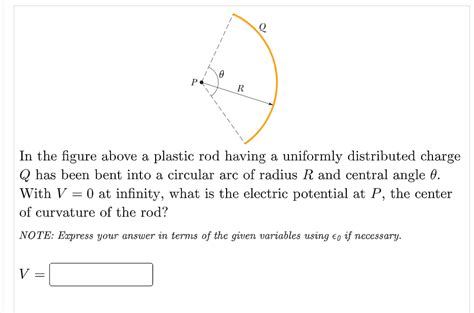
Table of Contents
Analyzing a Uniformly Charged Plastic Rod: Electrostatic Fields and Potential
This article delves into the physics of a uniformly charged plastic rod, exploring the electrostatic field and potential it generates. We'll examine the problem using both the principle of superposition and Gauss's law, comparing the approaches and highlighting their strengths and limitations. We'll also discuss practical considerations and applications of this fundamental electrostatic problem.
Understanding the Problem: A Uniformly Charged Rod
Imagine a thin, straight plastic rod of length 'L' carrying a uniformly distributed charge 'Q'. This means that the charge is evenly spread along the rod's length, resulting in a linear charge density, λ (lambda), defined as:
λ = Q / L
Our goal is to determine the electric field E and electric potential V at various points in space surrounding this charged rod. This is a classic problem in electrostatics that illustrates fundamental concepts and problem-solving techniques.
Method 1: Superposition Principle
The superposition principle states that the total electric field at any point is the vector sum of the electric fields produced by each individual charge element. We can consider the rod to be composed of infinitesimal charge elements, dq, each producing a small electric field, dE.
1. Defining the Charge Element:
Let's consider a small segment of the rod of length dx located at a distance x from one end. The charge dq on this segment is:
dq = λdx = (Q/L)dx
2. Calculating the Electric Field due to a Single Element:
The electric field dE produced by this charge element dq at a point P located at a distance r from the element, making an angle θ with the rod, can be calculated using Coulomb's Law:
dE = k * dq / r²
where k is Coulomb's constant (approximately 8.98755 × 10⁹ N⋅m²/C²). We need to resolve this electric field into its components, usually x and y components, depending on the coordinate system chosen.
3. Integrating to find the Total Electric Field:
To find the total electric field E at point P, we need to integrate the contributions from all the charge elements along the rod:
E = ∫ dE
This integral is often quite complex and depends heavily on the chosen coordinate system and the position of point P relative to the rod. The integration process involves expressing r and the angles in terms of x and the coordinates of point P. The solution will typically involve trigonometric functions and may require the use of integral tables or software tools for symbolic integration.
4. Special Case: Point on the Perpendicular Bisector:
A simplification occurs if we consider a point P located on the perpendicular bisector of the rod, a distance 'y' from the center. Due to symmetry, the x-components of the electric field cancel out, and the total electric field points directly away from the rod (or towards it if the charge is negative). The integration becomes significantly simpler, yielding:
E = (2kλ / y) * [L / (4y² + L²)^½]
This equation provides the magnitude of the electric field at a point on the perpendicular bisector.
Method 2: Gauss's Law
Gauss's law offers an alternative approach, especially advantageous for situations with high symmetry. It states that the flux of the electric field through a closed surface is proportional to the enclosed charge:
∮ E • dA = Q_enclosed / ε₀
where ε₀ is the permittivity of free space (approximately 8.854 × 10⁻¹² C²/N⋅m²).
1. Choosing a Gaussian Surface:
For a uniformly charged rod, a cylindrical Gaussian surface is a suitable choice. The cylinder should be coaxial with the rod, with its axis coinciding with the rod. The cylinder's radius should be 'r', and its length should be 'l' (less than or equal to L).
2. Applying Gauss's Law:
The electric field will be perpendicular to the cylindrical surface, and its magnitude will be constant at a given radius. Thus, the flux through the curved surface area of the cylinder is:
∮ E • dA = E * 2πrl
The flux through the end caps is zero, as the electric field is parallel to the surface there. The enclosed charge is:
Q_enclosed = λl
Therefore, Gauss's law becomes:
E * 2πrl = λl / ε₀
3. Solving for the Electric Field:
Solving for E gives:
E = λ / (2πε₀r)
This equation provides the magnitude of the electric field at a distance 'r' from the rod's axis. Note that this equation assumes an infinitely long rod. For a finite rod, the result obtained from Gauss's law is approximate but provides a good approximation at distances significantly smaller than the length of the rod.
Comparing the Two Methods
Both the superposition principle and Gauss's law lead to expressions for the electric field produced by a uniformly charged rod. The superposition method is more general, able to handle non-uniform charge distributions and more complex geometries. However, the integration can become extremely challenging. Gauss's law, while more elegant and often simpler, requires a high degree of symmetry to be effective. Its usefulness hinges on carefully selecting an appropriate Gaussian surface. In many cases, Gauss's Law yields an approximate result whereas superposition method yields an exact solution.
Electric Potential
The electric potential V at a point in space is the work done per unit charge to bring a test charge from infinity to that point. It's a scalar quantity, making calculations often simpler than electric field calculations. The potential due to the uniformly charged rod can be calculated by integrating the potential contribution from each infinitesimal charge element:
V = ∫ k dq / r
Similar to the electric field calculation, this integral also depends on the location of the point and the geometry. The solution can be quite complex, especially for arbitrary points in space. However, for specific points, the integration can be simplified. For example, at a distance r from the center of the rod on its perpendicular bisector, the potential is approximately given by:
V ≈ (kQ/L) * ln[(L/2 + √(L²/4 + r²))/r]
Practical Applications
Understanding the electric field and potential of a uniformly charged rod has numerous practical applications, including:
-
Modeling of charged wires and cables: Many electrical systems involve charged wires or cables. The model of a uniformly charged rod provides a good approximation for calculating the electric fields and forces associated with these systems.
-
Design of electrostatic devices: This understanding is crucial for the design of electrostatic devices such as electrostatic precipitators used in air pollution control.
-
Analysis of charged particle beams: The principles learned from solving this problem inform the understanding and control of charged particle beams in accelerators and other scientific instruments.
-
Understanding lightning rods: The electric field created by a charged rod is relevant to understanding the behavior of lightning rods which are designed to attract lightning discharges away from protected structures.
-
Electrostatic painting: In electrostatic painting, the charged paint particles are attracted to the grounded object, leading to more efficient and uniform coating. The principles involved here can be modelled using the charged rod approach.
Conclusion
The analysis of a uniformly charged plastic rod is a foundational problem in electrostatics. It provides an excellent platform for understanding and applying the superposition principle and Gauss's law. While seemingly simple, this problem highlights the challenges and nuances of calculating electric fields and potentials, especially when dealing with continuous charge distributions. The knowledge gained from solving this problem has numerous applications in various fields of science and engineering. Further exploration can include considering non-uniform charge distributions, curved rods, and the effects of nearby conductors. The insights from this basic model form a critical stepping stone towards tackling more complex electrostatic scenarios.
Latest Posts
Latest Posts
-
What Is Not True Regarding Antibiotics
Mar 31, 2025
-
Balanced Equation For Copper And Nitric Acid
Mar 31, 2025
-
Passwords Passphrases And Pins Are Examples Of Which Security Term
Mar 31, 2025
-
Both Glucose And Fructose Are
Mar 31, 2025
-
A Perfectly Elastic Demand Curve Implies That The Firm
Mar 31, 2025
Related Post
Thank you for visiting our website which covers about In The Figure A Plastic Rod Having A Uniformly . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
