If A Transversal Intersects Two Parallel Lines Then
News Leon
Apr 01, 2025 · 6 min read
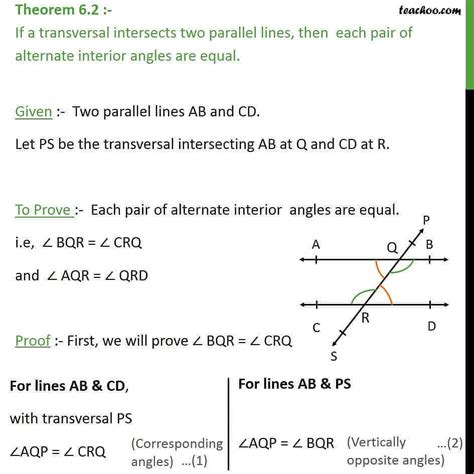
Table of Contents
If a Transversal Intersects Two Parallel Lines, Then... A Deep Dive into Geometry
Geometry, the study of shapes, sizes, relative positions of figures, and the properties of space, offers a rich tapestry of theorems and postulates. One of the foundational concepts, particularly relevant to understanding parallel lines, is the relationship that arises when a transversal intersects them. This exploration will delve into the properties, theorems, and applications stemming from this fundamental geometric principle.
Understanding Parallel Lines and Transversals
Before we dissect the consequences of a transversal intersecting parallel lines, let's define the key terms:
Parallel Lines: Two lines are parallel if they lie in the same plane and never intersect, no matter how far they are extended. We often denote parallel lines using the symbol ∥. For instance, line l ∥ line m indicates that line l is parallel to line m.
Transversal: A transversal is a line that intersects two or more other lines at distinct points. It's crucial to understand that the transversal must intersect the other lines; it doesn't simply run alongside them.
The Core Theorems: Corresponding, Alternate Interior, and Alternate Exterior Angles
When a transversal intersects two parallel lines, several pairs of angles are created. These angles exhibit specific relationships, governed by three primary theorems:
1. Corresponding Angles Theorem
Corresponding angles are pairs of angles that are in the same relative position at an intersection when a line intersects two other lines. If a transversal intersects two parallel lines, then corresponding angles are congruent (equal in measure).
Visual Representation:
Imagine line l and line m being parallel (l ∥ m), intersected by transversal line t. Several pairs of corresponding angles exist:
- Angle 1 and Angle 5
- Angle 2 and Angle 6
- Angle 3 and Angle 7
- Angle 4 and Angle 8
If ∠1 = ∠5, then we know, by the Corresponding Angles Theorem, that lines l and m are parallel. This theorem is crucial for proving lines parallel and for solving various geometric problems.
2. Alternate Interior Angles Theorem
Alternate interior angles are pairs of angles that lie on opposite sides of the transversal and between the two parallel lines. If a transversal intersects two parallel lines, then alternate interior angles are congruent.
Visual Representation:
Referring to the same diagram, the alternate interior angles are:
- Angle 3 and Angle 6
- Angle 4 and Angle 5
If ∠3 = ∠6 (or ∠4 = ∠5), it provides further confirmation of the parallel relationship between lines l and m.
3. Alternate Exterior Angles Theorem
Alternate exterior angles are pairs of angles that lie on opposite sides of the transversal and outside the two parallel lines. If a transversal intersects two parallel lines, then alternate exterior angles are congruent.
Visual Representation:
In our diagram, the alternate exterior angles are:
- Angle 1 and Angle 8
- Angle 2 and Angle 7
The congruence of these angles (∠1 = ∠8 or ∠2 = ∠7) reinforces the parallelism between lines l and m.
Consecutive Interior Angles Theorem: A Different Relationship
While the previous theorems focused on congruent angles, the Consecutive Interior Angles Theorem highlights a different relationship:
Consecutive interior angles are pairs of angles that lie on the same side of the transversal and between the two parallel lines. If a transversal intersects two parallel lines, then consecutive interior angles are supplementary (their sum is 180°).
Visual Representation:
The consecutive interior angles in our example are:
- Angle 3 and Angle 5
- Angle 4 and Angle 6
Therefore, ∠3 + ∠5 = 180° and ∠4 + ∠6 = 180°. This theorem provides another way to verify the parallelism of lines or to solve for unknown angles.
Proofs and Deductive Reasoning: The Foundation of Geometric Understanding
The theorems presented above aren't simply statements; they are rigorously proven using deductive reasoning based on axioms and postulates of Euclidean geometry. Understanding these proofs solidifies the understanding of these relationships. A common approach involves using the properties of parallel lines and the definition of angles formed by intersecting lines. For example, proving the Corresponding Angles Theorem often involves constructing auxiliary lines parallel to the given parallel lines and demonstrating angle congruence based on vertical angles and linear pairs.
Applications and Real-World Examples
The concepts discussed aren't confined to theoretical geometry; they have numerous practical applications:
-
Construction and Engineering: Parallel lines and transversals are fundamental in construction, ensuring structural integrity and accurate measurements. Understanding angle relationships is critical in building frameworks, laying foundations, and creating level surfaces.
-
Architecture and Design: Architects and designers utilize these principles to create visually appealing and structurally sound buildings. Parallel lines and angles influence the design of windows, doors, and overall building proportions.
-
Cartography and Navigation: Mapping and navigation rely heavily on parallel lines and transversals. Latitude and longitude lines, which are parallel and intersect at right angles, form the basis of many coordinate systems.
-
Computer Graphics and Computer-Aided Design (CAD): The principles governing parallel lines and transversals are crucial in computer graphics and CAD software. These principles enable the creation of precise drawings, simulations, and 3D models. Understanding how lines intersect and the resulting angles allows for accurate representation of objects and scenes.
Solving Problems: Putting Theory into Practice
Let's consider a practical example to solidify our understanding:
Problem: Two parallel lines are intersected by a transversal. One of the alternate interior angles measures 65°. Find the measure of the other alternate interior angle and the corresponding angle to the given angle.
Solution:
-
Alternate Interior Angle: Since alternate interior angles are congruent when a transversal intersects parallel lines, the other alternate interior angle also measures 65°.
-
Corresponding Angle: Corresponding angles are also congruent. Therefore, the corresponding angle to the given 65° angle also measures 65°.
This simple example illustrates how the theorems provide a straightforward method for solving problems involving parallel lines and transversals.
Advanced Applications and Extensions
Beyond the basic theorems, the intersection of a transversal with parallel lines plays a critical role in:
-
Projective Geometry: This branch of geometry deals with properties of figures that remain invariant under projection, often involving parallel lines and their intersections at infinity.
-
Trigonometry: The concepts of parallel lines and transversals are used to derive trigonometric identities and solve trigonometric equations.
-
Vector Geometry: Vector methods can be used to prove the theorems related to parallel lines and transversals, offering a different perspective on these geometrical relationships.
Conclusion: A Cornerstone of Geometry
The intersection of a transversal with two parallel lines is a fundamental concept in geometry. The resulting relationships between angles – corresponding, alternate interior, alternate exterior, and consecutive interior – are essential for proving theorems, solving geometric problems, and understanding the structure of space. The application of these principles extends far beyond the classroom, playing a significant role in numerous fields, including engineering, architecture, and computer graphics. A solid grasp of these concepts is crucial for anyone pursuing further studies in mathematics or fields that rely on geometric principles. The ability to visualize and analyze these relationships is key to problem-solving and innovation in various technical and creative domains.
Latest Posts
Latest Posts
-
What Number Is 45 Of 60
Apr 02, 2025
-
Is Oxygen A Solid Liquid Or A Gas
Apr 02, 2025
-
Which Of The Following Is A Source Of Income
Apr 02, 2025
-
What Happens When Gas Is Heated
Apr 02, 2025
-
Cobalt Ii Hydrogen Carbonate Chemical Formula
Apr 02, 2025
Related Post
Thank you for visiting our website which covers about If A Transversal Intersects Two Parallel Lines Then . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
