How Do You Factor 2x 2 7x 3
News Leon
Mar 29, 2025 · 5 min read
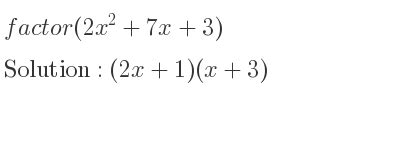
Table of Contents
How Do You Factor 2x² + 7x + 3? A Comprehensive Guide to Quadratic Factoring
Factoring quadratic expressions is a fundamental skill in algebra. Understanding how to factor these expressions is crucial for solving quadratic equations, simplifying algebraic expressions, and tackling more advanced mathematical concepts. This comprehensive guide will walk you through the process of factoring the quadratic expression 2x² + 7x + 3, explaining the methods involved and providing examples to solidify your understanding. We'll explore various techniques, ensuring you grasp the underlying principles and can confidently apply them to other quadratic expressions.
Understanding Quadratic Expressions
Before diving into the factoring process, let's establish a clear understanding of what a quadratic expression is. A quadratic expression is a polynomial of degree two, meaning the highest power of the variable (usually 'x') is 2. It generally takes the form:
ax² + bx + c
where 'a', 'b', and 'c' are constants (numbers), and 'a' is not equal to zero. In our case, we have the quadratic expression:
2x² + 7x + 3
Here, a = 2, b = 7, and c = 3. Our goal is to express this quadratic as a product of two simpler expressions, typically two binomials.
Method 1: AC Method (Splitting the Middle Term)
This is a common method for factoring quadratic expressions, especially when the coefficient of x² (a) is not 1. Here's how it works:
-
Find the product 'ac': Multiply the coefficient of x² (a) by the constant term (c). In our example: ac = 2 * 3 = 6
-
Find two numbers that add up to 'b' and multiply to 'ac': We need two numbers that add up to 7 (the coefficient of x, which is b) and multiply to 6. These numbers are 1 and 6 (1 + 6 = 7 and 1 * 6 = 6).
-
Rewrite the middle term: Split the middle term (7x) using the two numbers we found:
2x² + 1x + 6x + 3
-
Factor by grouping: Group the terms in pairs and factor out the greatest common factor (GCF) from each pair:
x(2x + 1) + 3(2x + 1)
-
Factor out the common binomial: Notice that (2x + 1) is a common factor in both terms. Factor it out:
(2x + 1)(x + 3)
Therefore, the factored form of 2x² + 7x + 3 is (2x + 1)(x + 3).
Method 2: Trial and Error
This method involves systematically trying different combinations of binomial factors until you find the correct one. It's particularly useful when the coefficients are small and relatively easy to work with.
-
Set up the binomial factors: Since the first term is 2x², we know the first terms of the binomials must be 2x and x:
(2x )(x )
-
Consider the factors of the constant term: The constant term is 3, and its factors are 1 and 3. We need to place these factors in the second positions of the binomials such that when we expand the expression using the FOIL method (First, Outer, Inner, Last), we get the original quadratic expression.
-
Test different combinations: Let's try placing 1 and 3 in different positions:
(2x + 1)(x + 3) or (2x + 3)(x + 1)
-
Expand and check: Let's expand the first combination using FOIL:
(2x + 1)(x + 3) = 2x² + 6x + x + 3 = 2x² + 7x + 3
This matches our original quadratic expression, so this is the correct factorization.
Method 3: Quadratic Formula (Indirect Factoring)
While not a direct factoring method, the quadratic formula can help find the roots of the quadratic equation 2x² + 7x + 3 = 0. These roots can then be used to determine the factors. The quadratic formula is:
x = [-b ± √(b² - 4ac)] / 2a
For our quadratic, a = 2, b = 7, and c = 3. Plugging these values into the formula:
x = [-7 ± √(7² - 4 * 2 * 3)] / (2 * 2) x = [-7 ± √(49 - 24)] / 4 x = [-7 ± √25] / 4 x = [-7 ± 5] / 4
This gives us two solutions:
x₁ = (-7 + 5) / 4 = -2/4 = -1/2 x₂ = (-7 - 5) / 4 = -12/4 = -3
The roots are -1/2 and -3. We can use these roots to find the factors:
- x = -1/2 implies a factor of (2x + 1) [since setting this equal to zero gives x = -1/2]
- x = -3 implies a factor of (x + 3)
Thus, we again arrive at the factored form: (2x + 1)(x + 3)
Verification and Checking Your Answer
It's crucial to always check your factored answer by expanding it using the FOIL method (First, Outer, Inner, Last):
(2x + 1)(x + 3) = 2x² + 6x + x + 3 = 2x² + 7x + 3
This confirms that our factorization is correct.
Why Factoring is Important
Factoring quadratic expressions is a fundamental algebraic skill with various applications:
-
Solving Quadratic Equations: Factoring allows us to easily solve quadratic equations by setting each factor equal to zero and solving for x. This provides the roots or solutions to the equation.
-
Simplifying Algebraic Expressions: Factoring can simplify complex algebraic expressions, making them easier to manipulate and analyze.
-
Graphing Quadratic Functions: The factored form of a quadratic expression reveals the x-intercepts (roots) of the corresponding quadratic function, which are crucial for accurately graphing the function.
-
Advanced Mathematical Concepts: Factoring forms the basis for many advanced mathematical concepts, including calculus and linear algebra.
Practice Problems
To solidify your understanding, try factoring the following quadratic expressions using the methods discussed:
- x² + 5x + 6
- 3x² + 8x + 4
- 4x² - 4x - 3
- x² - 7x + 12
- 2x² - 5x - 3
Remember to always check your answers by expanding the factored form.
Conclusion
Factoring quadratic expressions, such as 2x² + 7x + 3, is a key algebraic skill. The AC method, trial and error, and even the indirect use of the quadratic formula are all valuable techniques to master. Understanding these methods and practicing regularly will build your confidence and proficiency in tackling more complex algebraic problems. Remember to always verify your factored answer by expanding it to ensure accuracy. With consistent practice, you'll become adept at factoring quadratic expressions and unlocking their many applications in mathematics.
Latest Posts
Latest Posts
-
Sulphuric Acid Sodium Hydroxide Balanced Equation
Apr 01, 2025
-
Which Factors Would Increase The Rate Of A Chemical Reaction
Apr 01, 2025
-
How Many Days Are In 2016
Apr 01, 2025
-
Naoh Is Strong Or Weak Base
Apr 01, 2025
-
What Is The Decimal For 11 12
Apr 01, 2025
Related Post
Thank you for visiting our website which covers about How Do You Factor 2x 2 7x 3 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
