Find The Values Of X And Y In Parallelogram Pqrs
News Leon
Apr 03, 2025 · 5 min read
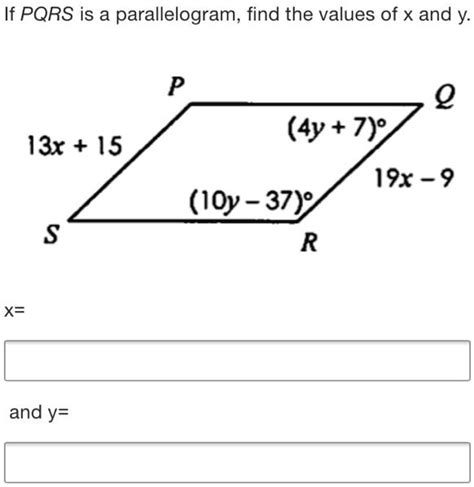
Table of Contents
Finding the Values of x and y in Parallelogram PQRS: A Comprehensive Guide
Finding the values of x and y in a parallelogram problem requires a solid understanding of parallelogram properties. This guide will walk you through various scenarios, providing step-by-step solutions and explaining the underlying geometric principles. We'll cover different approaches, including using algebraic equations and applying the properties of parallel lines and transversals. By the end, you'll be confident in tackling any parallelogram problem involving finding x and y.
Understanding Parallelogram Properties
Before diving into specific problems, let's review the key properties of parallelograms that are crucial for solving these types of questions. Remember, a parallelogram is a quadrilateral with opposite sides parallel and equal in length. This fundamental property leads to several other important characteristics:
- Opposite sides are parallel: This is the defining characteristic. Sides PQ and RS are parallel, as are sides PS and QR.
- Opposite sides are congruent: PQ = RS and PS = QR.
- Opposite angles are congruent: ∠P = ∠R and ∠Q = ∠S.
- Consecutive angles are supplementary: ∠P + ∠Q = 180°, ∠Q + ∠R = 180°, ∠R + ∠S = 180°, and ∠S + ∠P = 180°.
- Diagonals bisect each other: The diagonals PR and QS intersect at a point, let's call it M, and PM = MR and QM = MS.
Understanding these properties is essential for setting up equations to solve for x and y.
Solving for x and y: Different Scenarios
Parallelogram problems involving x and y can take many forms. Let's explore various scenarios with detailed examples:
Scenario 1: Using Opposite Sides
Problem: In parallelogram PQRS, PQ = 2x + 5, RS = 3x - 2, PS = y + 7, and QR = 2y - 3. Find the values of x and y.
Solution:
Since opposite sides of a parallelogram are congruent, we can set up two equations:
- Equation 1 (Opposite Sides): 2x + 5 = 3x - 2
- Equation 2 (Opposite Sides): y + 7 = 2y - 3
Solving Equation 1:
- Subtract 2x from both sides: 5 = x - 2
- Add 2 to both sides: x = 7
Solving Equation 2:
- Subtract y from both sides: 7 = y - 3
- Add 3 to both sides: y = 10
Therefore, x = 7 and y = 10.
Scenario 2: Using Opposite Angles
Problem: In parallelogram PQRS, ∠P = 3x + 10 and ∠R = 4x - 20. Find the value of x and the measure of ∠P and ∠R.
Solution:
Opposite angles in a parallelogram are congruent, so:
- Equation 1 (Opposite Angles): 3x + 10 = 4x - 20
Solving Equation 1:
- Subtract 3x from both sides: 10 = x - 20
- Add 20 to both sides: x = 30
Now substitute x = 30 into the expressions for ∠P and ∠R:
- ∠P = 3(30) + 10 = 100°
- ∠R = 4(30) - 20 = 100°
Therefore, x = 30, ∠P = 100°, and ∠R = 100°.
Scenario 3: Using Consecutive Angles
Problem: In parallelogram PQRS, ∠P = 2x + y and ∠Q = 3x - 2y. Given that consecutive angles are supplementary and ∠P = 110°, find x and y.
Solution:
We know that consecutive angles in a parallelogram are supplementary, meaning their sum is 180°. Thus:
- Equation 1 (Supplementary Angles): (2x + y) + (3x - 2y) = 180°
- Equation 2 (Given Angle): 2x + y = 110°
Simplify Equation 1:
- 5x - y = 180°
Now we have a system of two linear equations:
- 2x + y = 110°
- 5x - y = 180°
We can solve this system using either substitution or elimination. Let's use elimination:
Add the two equations:
- 7x = 290°
- x = 290°/7 ≈ 41.43°
Substitute x back into Equation 2:
- 2(41.43) + y = 110°
- 82.86 + y = 110°
- y = 110° - 82.86° ≈ 27.14°
Therefore, x ≈ 41.43° and y ≈ 27.14°. Note that these values are approximate due to the nature of the solution.
Scenario 4: Using Diagonals
Problem: The diagonals of parallelogram PQRS intersect at point M. PM = 3x + 2 and MR = 5x - 10. Find the value of x and the length of PR.
Solution:
The diagonals of a parallelogram bisect each other. Therefore, PM = MR. This gives us:
- Equation 1 (Diagonal Bisector): 3x + 2 = 5x - 10
Solving Equation 1:
- Subtract 3x from both sides: 2 = 2x - 10
- Add 10 to both sides: 12 = 2x
- Divide by 2: x = 6
Now find the length of PR:
- PR = PM + MR = (3x + 2) + (5x - 10) = 8x - 8 = 8(6) - 8 = 40
Therefore, x = 6 and PR = 40.
Advanced Problems and Strategies
More complex problems might involve combining several parallelogram properties or incorporating other geometric concepts like similar triangles or trigonometry. Let's consider one such advanced example:
Scenario 5: Combining Properties
Problem: In parallelogram PQRS, ∠P = 120°. The bisectors of ∠P and ∠Q intersect at point T. Find ∠PTQ.
Solution:
This problem requires understanding angle bisectors and their relationship to parallelogram angles.
-
Consecutive angles are supplementary: Since ∠P = 120°, ∠Q = 180° - 120° = 60°.
-
Angle bisectors: The bisectors of ∠P and ∠Q divide these angles in half. Therefore, ∠TPA = ∠TPQ = 60°, and ∠TQA = ∠TQP = 30°.
-
Sum of angles in a triangle: Consider triangle PTQ. The sum of angles in a triangle is 180°. We have:
- ∠PTQ + ∠TQP + ∠TPQ = 180°
- ∠PTQ + 30° + 60° = 180°
- ∠PTQ = 180° - 90° = 90°
Therefore, ∠PTQ = 90°.
Conclusion
Solving for x and y in parallelogram problems requires a thorough understanding of parallelogram properties and the ability to set up and solve algebraic equations. By mastering these techniques and practicing with various scenarios, you'll develop the skills needed to tackle increasingly complex geometry problems. Remember to always clearly define your variables, show your work step-by-step, and double-check your solutions to ensure accuracy. This comprehensive guide provides a solid foundation for your success in solving parallelogram problems. Keep practicing, and you'll become proficient in handling these types of questions with confidence.
Latest Posts
Latest Posts
-
What Binds To The Exposed Cross Bridges On Actin
Apr 04, 2025
-
All Squares Are Rectangles And Rhombuses
Apr 04, 2025
-
What Is The Role Of Toothpaste In Preventing Cavities
Apr 04, 2025
-
Is A Grasshopper A Producer Consumer Or Decomposer
Apr 04, 2025
-
Ac And Bd Bisect Each Other
Apr 04, 2025
Related Post
Thank you for visiting our website which covers about Find The Values Of X And Y In Parallelogram Pqrs . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
