Calculate Energy Stored In A Spring
News Leon
Apr 02, 2025 · 6 min read
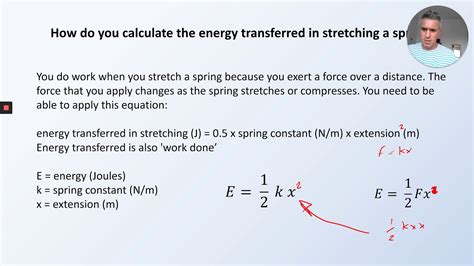
Table of Contents
Calculating the Energy Stored in a Spring: A Comprehensive Guide
Understanding how to calculate the energy stored in a spring is crucial in various fields, from engineering and physics to designing everyday objects. Springs are ubiquitous – they're in your car, your pen, and even your mattress! This comprehensive guide will walk you through the principles behind spring potential energy, explore different types of springs, and delve into the formulas and calculations needed to accurately determine the energy stored within them.
Understanding Spring Potential Energy
Springs are remarkable devices that store mechanical energy in the form of potential energy. This potential energy is a consequence of the work done in stretching or compressing the spring from its equilibrium position. When a force is applied to stretch or compress a spring, it deforms. This deformation results in the storage of energy within the spring's internal structure. When the force is removed, the spring returns to its original shape, releasing the stored energy.
This stored energy is directly proportional to the amount of deformation. The greater the stretch or compression, the greater the energy stored. This relationship forms the basis for calculating the energy stored within a spring.
Hooke's Law: The Foundation
The foundation for understanding spring energy lies in Hooke's Law. This law states that the force required to stretch or compress a spring is directly proportional to the distance the spring is stretched or compressed from its equilibrium position. Mathematically, this is represented as:
F = -kx
Where:
- F represents the force applied to the spring (in Newtons)
- k represents the spring constant (in Newtons per meter, N/m) – a measure of the spring's stiffness. A higher spring constant indicates a stiffer spring.
- x represents the displacement of the spring from its equilibrium position (in meters). The negative sign indicates that the force exerted by the spring is in the opposite direction to the displacement.
This equation is crucial because it allows us to relate the force applied to the spring's deformation.
Calculating Spring Potential Energy: The Formula
The energy stored in a spring, also known as elastic potential energy (U), is calculated using the following formula, derived from Hooke's Law and the principles of work and energy:
U = (1/2)kx²
Where:
- U represents the elastic potential energy (in Joules)
- k is the spring constant (in N/m)
- x is the displacement from the equilibrium position (in meters)
This formula shows that the energy stored is directly proportional to the square of the displacement. This means that doubling the displacement quadruples the energy stored.
Example Calculation:
Let's say we have a spring with a spring constant (k) of 100 N/m. We stretch the spring 0.1 meters from its equilibrium position. The energy stored in the spring can be calculated as follows:
U = (1/2) * 100 N/m * (0.1 m)² = 0.5 Joules
Therefore, the spring stores 0.5 Joules of elastic potential energy.
Different Types of Springs and Their Considerations
While the fundamental principle of calculating potential energy remains the same, various spring types exist, each with its nuances:
1. Coil Springs (Helical Springs):
These are the most common type, consisting of a coiled wire. The formula U = (1/2)kx² applies directly to ideal coil springs. However, in real-world scenarios, factors such as friction and non-linearity (where Hooke's Law doesn't perfectly hold at larger displacements) can slightly affect the accuracy of the calculation.
2. Leaf Springs:
Used in vehicles and other applications, these are essentially flat, elongated springs that flex under load. Calculating the potential energy for leaf springs is more complex and often requires more advanced mathematical techniques, considering the spring's geometry and material properties.
3. Torsion Springs:
These springs store energy by twisting. The formula for potential energy in a torsion spring is slightly different:
U = (1/2)kθ²
Where:
- U is the potential energy
- k is the torsion spring constant (in N⋅m/rad)
- θ is the angle of twist (in radians)
4. Rubber Springs:
These utilize the elasticity of rubber to store energy. Calculating their potential energy is more complicated than for metal springs because rubber exhibits non-linear elastic behavior. Specialized models and experimental data are often needed for accurate calculation.
Beyond the Basic Formula: Considering Non-Idealities
The formula U = (1/2)kx² is based on the assumption of an ideal spring. Real-world springs deviate from this ideal behavior in several ways:
-
Non-linearity: At larger displacements, many springs exhibit non-linear behavior, meaning Hooke's Law doesn't precisely hold. This requires more complex mathematical models for accurate energy calculation.
-
Friction: Internal friction within the spring dissipates some energy during compression and extension, meaning the actual energy stored might be slightly less than predicted by the formula.
-
Material Properties: The material properties of the spring (such as its Young's modulus) affect its stiffness and energy storage capacity. Variations in material properties can lead to deviations from the ideal calculation.
-
Temperature: Temperature changes can affect the spring's stiffness and thus its energy storage capability.
Practical Applications and Real-World Examples
Understanding how to calculate the energy stored in a spring has numerous practical applications across various disciplines:
-
Mechanical Engineering: Designing suspension systems for vehicles, designing springs for machines, and calculating the energy required for various mechanical processes.
-
Civil Engineering: Designing structures that utilize springs for vibration damping and energy absorption.
-
Aerospace Engineering: Designing spring mechanisms for spacecraft deployment and other applications where precise energy storage is critical.
-
Physics Experiments: Springs are frequently used in experiments to demonstrate concepts like energy conservation, simple harmonic motion, and oscillations.
Examples:
-
Automotive Suspension: The suspension system of a car uses springs to absorb shocks and vibrations from the road. Calculating the energy stored in these springs is crucial for designing a comfortable and safe ride.
-
Clock Mechanisms: Many clocks use springs to store energy, which is then gradually released to power the clock's movement. Precise calculation of the spring's energy is essential for accurate timekeeping.
-
Toy Design: Many toys utilize springs for their mechanisms. The energy stored in these springs dictates the toy's performance and functionality.
Advanced Techniques for Complex Spring Systems
For complex spring systems, where multiple springs interact or where non-linear behavior dominates, more advanced techniques are needed:
-
Finite Element Analysis (FEA): FEA is a powerful computational technique used to model the behavior of complex systems, including springs. It allows for precise calculation of energy storage, considering factors such as geometry, material properties, and boundary conditions.
-
Experimental Measurements: In some cases, direct experimental measurement of energy storage may be necessary. This involves measuring the force applied to the spring and the resulting displacement, then using this data to calculate the energy stored.
Conclusion
Calculating the energy stored in a spring is a fundamental concept with wide-ranging applications. While the basic formula U = (1/2)kx² provides a good approximation for ideal springs, real-world scenarios often require consideration of non-idealities. Understanding these complexities, along with advanced techniques like FEA, allows for accurate calculation and design of spring systems in various engineering applications. By mastering this knowledge, you can contribute to innovative solutions across multiple fields.
Latest Posts
Latest Posts
-
1 A 2 X 2 Integral Formula
Apr 03, 2025
-
The Two Most Abundant Components Of Dry Air Are
Apr 03, 2025
-
Work Done By A Gas In Isothermal Expansion
Apr 03, 2025
-
Conversion Of L Atm To J
Apr 03, 2025
-
Coal Is An Example Of Which Type Of Sedimentary Rock
Apr 03, 2025
Related Post
Thank you for visiting our website which covers about Calculate Energy Stored In A Spring . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
