An Infinite Line Of Charge Produces A Field Of Magnitude
News Leon
Mar 30, 2025 · 6 min read
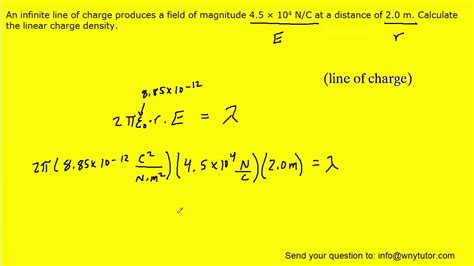
Table of Contents
An Infinite Line of Charge: Understanding its Electric Field
The concept of an infinite line of charge, while a theoretical construct, serves as a powerful tool in electrostatics for understanding the behavior of electric fields. It provides a simplified model for analyzing the fields generated by long, thin objects like wires carrying a charge. This article delves into the derivation and implications of the electric field produced by an infinite line of charge, exploring various aspects such as Gauss's Law, symmetry considerations, and practical applications.
Understanding the Problem: An Infinite Line of Charge
Imagine a line of charge extending infinitely in both directions. This line carries a uniform linear charge density, denoted by λ (lambda), measured in Coulombs per meter (C/m). This means that for any given length of the line, the charge is evenly distributed. Our goal is to determine the magnitude of the electric field at a radial distance r from this infinite line of charge.
Why an Infinite Line?
While a truly infinite line of charge doesn't exist in reality, the model is useful for several reasons:
- Simplification: Dealing with an infinitely long line simplifies the calculations significantly compared to a finite line of charge, where the contributions from the ends must be considered.
- Approximation: For long, thin charged objects, the infinite line model provides a good approximation of the electric field at points far from the ends. The error introduced by this approximation diminishes as the distance from the ends increases relative to the length of the object.
- Analytical Solution: The infinite line of charge allows for a relatively straightforward analytical solution using Gauss's Law, enabling a deeper understanding of the principles involved.
Applying Gauss's Law: The Key to the Solution
Gauss's Law is a fundamental theorem in electromagnetism that relates the flux of the electric field through a closed surface to the enclosed charge. Mathematically, it's expressed as:
∮ E ⋅ dA = Q<sub>enc</sub> / ε₀
Where:
- E is the electric field vector.
- dA is a vector representing an infinitesimal area element of the closed surface, directed outwards.
- Q<sub>enc</sub> is the total charge enclosed within the surface.
- ε₀ is the permittivity of free space (a fundamental constant).
The key to solving our problem lies in choosing an appropriate Gaussian surface—a strategically chosen closed surface that simplifies the integral in Gauss's Law.
Choosing the Gaussian Surface: Cylindrical Symmetry
Given the cylindrical symmetry of the infinite line of charge, the ideal Gaussian surface is a cylinder of radius r and length l, coaxial with the line of charge. This choice simplifies the problem due to the following reasons:
- Symmetry: The electric field is radially outward (or inward, depending on the sign of λ) and has the same magnitude at every point on the curved surface of the cylinder. This means that E and dA are parallel on the curved surface.
- Zero Flux: The electric field lines are parallel to the flat ends of the cylinder. Therefore, the electric flux through these ends is zero (E ⋅ dA = 0).
This strategic choice drastically reduces the complexity of the integral in Gauss's Law.
Derivation of the Electric Field Magnitude
Let's break down the application of Gauss's Law:
-
The Enclosed Charge: The charge enclosed within our cylindrical Gaussian surface is simply the linear charge density λ multiplied by the length of the cylinder, l: Q<sub>enc</sub> = λ*l.
-
The Flux through the Curved Surface: The flux through the curved surface is given by:
∮<sub>curved surface</sub> E ⋅ dA = E * (2πrl)
The electric field E is constant in magnitude across the curved surface due to the symmetry. The area of the curved surface is 2πrl.
- Applying Gauss's Law: Substituting the enclosed charge and the flux into Gauss's Law, we get:
E * (2πrl) = λl / ε₀
- Solving for E: Solving for the magnitude of the electric field, E, we obtain:
E = λ / (2πε₀r)
This equation reveals that the magnitude of the electric field produced by an infinite line of charge is inversely proportional to the radial distance r from the line. It also demonstrates that the electric field is independent of the length of the Gaussian cylinder, l—a direct consequence of the infinite extent of the line charge.
Understanding the Implications of the Result
The derived equation, E = λ / (2πε₀r), offers several crucial insights:
- Inverse Relationship: The electric field strength decreases as the distance from the line increases. This is a characteristic feature of linear charge distributions.
- Independence of Length: The field strength is independent of the length of the Gaussian surface, consistent with the infinite nature of the line charge.
- Radial Symmetry: The electric field is radially directed, pointing away from (or towards, depending on the sign of λ) the line of charge.
Limitations and Extensions of the Model
While the infinite line of charge model is a valuable tool, it's crucial to understand its limitations:
- Theoretical Construct: An infinitely long line of charge is a theoretical idealization. Real-world charge distributions are always finite.
- Approximation for Long Wires: The model provides a good approximation for long, thin wires only at distances much smaller than the wire's length. Near the ends of a finite wire, the electric field deviates significantly from the inverse-distance relationship.
Despite these limitations, this model provides a strong foundation for understanding electric fields and lays the groundwork for handling more complex scenarios involving charge distributions. Further extensions include:
- Finite Line of Charge: Calculating the electric field of a finite line of charge requires integration techniques due to the lack of symmetry along the length.
- Non-uniform Charge Density: If the linear charge density is not uniform, the calculation becomes significantly more complex, necessitating integration techniques to account for the varying charge contributions.
Practical Applications and Further Exploration
The infinite line of charge model finds applications in several areas:
- Coaxial Cables: The model is useful for understanding the electric fields within coaxial cables, which consist of concentric cylindrical conductors.
- High Voltage Transmission Lines: The model provides a reasonable approximation for the electric fields around long, high-voltage transmission lines, allowing for safety assessments and design considerations.
- Electrostatic Precipitators: The principles governing the electric field around an infinite line of charge are crucial in understanding the operation of electrostatic precipitators, which use electric fields to remove particles from gases.
Further explorations into this topic might include:
- Numerical simulations: Using software like MATLAB or Python to simulate the electric field of a finite line of charge and compare it with the analytical results for an infinite line.
- Experimental verification: Designing an experiment to measure the electric field around a long, charged wire and comparing the results with the theoretical predictions.
This comprehensive exploration of the electric field produced by an infinite line of charge provides a solid foundation for understanding electrostatic principles and their applications in various real-world scenarios. The model's simplicity, despite its theoretical nature, makes it an invaluable tool for learning and applying the concepts of electromagnetism. By understanding its limitations and extensions, one can effectively utilize this model for a range of practical applications and deeper theoretical investigations.
Latest Posts
Latest Posts
-
Greatest Amount Of Digestion Takes Place In The
Apr 01, 2025
-
All Real Numbers Are Rational Numbers True Or False
Apr 01, 2025
-
Which Of The Following Is A Function That Money Serves
Apr 01, 2025
-
The Most Abundant Compound In Most Living Things Is
Apr 01, 2025
-
How Can We Change The Polarity Of An Electromagnet
Apr 01, 2025
Related Post
Thank you for visiting our website which covers about An Infinite Line Of Charge Produces A Field Of Magnitude . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
