All Real Numbers Are Rational Numbers True Or False
News Leon
Apr 01, 2025 · 6 min read
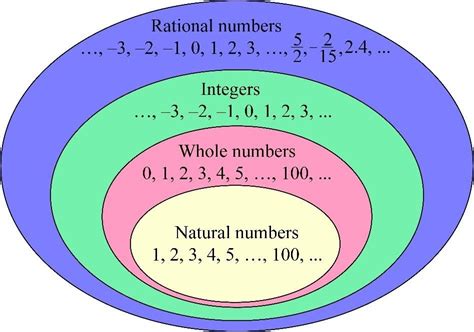
Table of Contents
All Real Numbers are Rational Numbers: True or False? A Deep Dive into Number Systems
The statement "All real numbers are rational numbers" is unequivocally false. This seemingly simple statement opens the door to a fascinating exploration of number systems, their properties, and the crucial distinctions between rational and irrational numbers. Understanding this difference is fundamental to various branches of mathematics, from basic arithmetic to advanced calculus. This article will delve into the precise definitions of rational and real numbers, illustrate the existence of irrational numbers, and explore why the inclusion of irrational numbers is critical to the completeness of the real number system.
Understanding Rational Numbers
A rational number is any number that can be expressed as the quotient or fraction p/q of two integers, where p is the numerator and q is the non-zero denominator. In simpler terms, it's a number that can be written as a fraction. This includes:
- Integers: Whole numbers, both positive and negative (e.g., -3, 0, 5). These can be expressed as fractions with a denominator of 1 (e.g., -3/1, 0/1, 5/1).
- Terminating Decimals: Decimals that end after a finite number of digits (e.g., 0.75, 2.5, -3.125). These can always be converted into fractions (e.g., 3/4, 5/2, -25/8).
- Repeating Decimals: Decimals that have a sequence of digits that repeats infinitely (e.g., 0.333..., 0.142857142857..., -1.234234...). These, too, can be represented as fractions using techniques involving geometric series.
Examples of Rational Numbers:
- 1/2
- -3/4
- 7
- 0
- 0.666... (repeating decimal, equivalent to 2/3)
- -2.75 (terminating decimal, equivalent to -11/4)
Understanding Real Numbers
Real numbers encompass all numbers that can be plotted on a number line. This vast set includes both rational and irrational numbers. The real numbers are considered complete, meaning there are no "gaps" on the number line. Every point on the number line corresponds to a real number, and every real number corresponds to a point on the number line. This completeness is a crucial property in calculus and analysis.
The Crux of the Matter: Irrational Numbers
The existence of irrational numbers directly refutes the statement that all real numbers are rational. An irrational number is a real number that cannot be expressed as a fraction p/q, where p and q are integers, and q is not zero. Their decimal representations are non-terminating and non-repeating, meaning they go on forever without ever settling into a repeating pattern.
Famous Examples of Irrational Numbers:
- π (Pi): The ratio of a circle's circumference to its diameter, approximately 3.1415926535... Its digits continue infinitely without repeating.
- e (Euler's number): The base of the natural logarithm, approximately 2.7182818284... Like π, its decimal representation is infinite and non-repeating.
- √2 (Square root of 2): This number, approximately 1.41421356..., cannot be expressed as a simple fraction. Its irrationality can be proven using proof by contradiction.
Why are irrational numbers crucial?
The inclusion of irrational numbers is essential for the completeness and consistency of the real number system. Without them, there would be "holes" on the number line. For example, the diagonal of a unit square (a square with sides of length 1) has a length of √2. If we only had rational numbers, we couldn't accurately represent this length on the number line.
Proof of the Existence of Irrational Numbers: The Case of √2
One of the most elegant and fundamental proofs in mathematics demonstrates that the square root of 2 (√2) is irrational. This proof relies on the technique of proof by contradiction:
-
Assumption: Assume, for the sake of contradiction, that √2 is rational. This means it can be expressed as a fraction p/q, where p and q are integers, q ≠ 0, and the fraction is in its simplest form (meaning p and q share no common factors other than 1).
-
Squaring Both Sides: Squaring both sides of the equation √2 = p/q, we get 2 = p²/q².
-
Rearranging: This can be rearranged to 2q² = p². This equation tells us that p² is an even number (since it's equal to 2 times another integer).
-
Implication for p: If p² is even, then p must also be even. This is because the square of an odd number is always odd. Since p is even, we can express it as p = 2k, where k is another integer.
-
Substitution: Substituting p = 2k into the equation 2q² = p², we get 2q² = (2k)² = 4k².
-
Simplifying: Dividing both sides by 2, we get q² = 2k². This shows that q² is also an even number.
-
Implication for q: Following the same logic as before, if q² is even, then q must also be even.
-
Contradiction: We've now shown that both p and q are even numbers. This contradicts our initial assumption that the fraction p/q is in its simplest form (because they share a common factor of 2).
-
Conclusion: Since our initial assumption leads to a contradiction, the assumption must be false. Therefore, √2 cannot be expressed as a fraction p/q, and it must be irrational.
This proof highlights the inherent difference between rational and irrational numbers and underscores why the set of real numbers must include both.
Implications and Applications
The distinction between rational and irrational numbers has profound implications across numerous fields:
- Geometry: The discovery of irrational numbers challenged the Pythagorean worldview, which relied on the belief that all lengths could be expressed as rational ratios.
- Calculus: The concept of limits and continuity relies heavily on the completeness of the real number system, including both rational and irrational numbers.
- Computer Science: Representing real numbers in computers requires approximations because most irrational numbers have infinite decimal expansions. Understanding the limitations of these approximations is crucial for numerical analysis and scientific computing.
- Physics and Engineering: Many physical quantities, such as the speed of light or gravitational constants, are often approximated by rational numbers, but their true values might be irrational.
Conclusion
The statement "All real numbers are rational numbers" is definitively false. The real number system's completeness demands the inclusion of irrational numbers. These numbers, which cannot be expressed as fractions of integers, fill the gaps in the number line and are fundamental to many mathematical concepts and applications. Understanding the differences and properties of rational and irrational numbers is crucial for a solid foundation in mathematics and its numerous applications across diverse fields. The elegant proof of the irrationality of √2 serves as a powerful example of mathematical reasoning and the fundamental importance of irrational numbers in the broader mathematical landscape. The richness and complexity of the real number system, which elegantly combines both rational and irrational numbers, is a testament to the profound beauty and utility of mathematics.
Latest Posts
Latest Posts
-
What Is The Absolute Value Of 16
Apr 02, 2025
-
Which Type Of Energy Is Stored Energy
Apr 02, 2025
-
Electric Field Inside A Hollow Sphere
Apr 02, 2025
-
Where Does Light Independent Reaction Take Place
Apr 02, 2025
-
X Minus Y Ka Whole Square
Apr 02, 2025
Related Post
Thank you for visiting our website which covers about All Real Numbers Are Rational Numbers True Or False . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
