X 4 29x 2 100 0
News Leon
Mar 28, 2025 · 5 min read
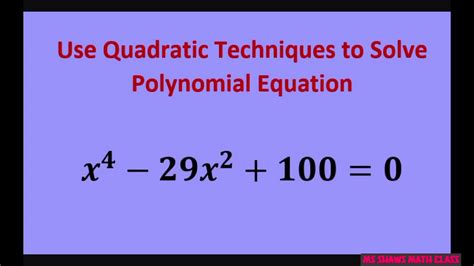
Table of Contents
Decoding the Enigma: Exploring the Mathematical Expression "x⁴ + 29x² + 100 = 0"
The seemingly simple quartic equation, x⁴ + 29x² + 100 = 0, presents a fascinating challenge for mathematicians and students alike. While it might appear intimidating at first glance, its solution reveals valuable insights into polynomial manipulation, factorization techniques, and the nature of quadratic and quartic equations. This comprehensive guide will delve into various methods to solve this equation, exploring the underlying concepts and offering a detailed step-by-step approach.
Understanding Quartic Equations
Before tackling the specific problem, let's establish a foundational understanding of quartic equations. A quartic equation is a polynomial equation of degree four, meaning the highest power of the variable (in this case, 'x') is four. The general form is:
ax⁴ + bx³ + cx² + dx + e = 0
where a, b, c, d, and e are constants, and 'a' is non-zero. Solving quartic equations can be significantly more complex than solving quadratic equations (degree two) or cubic equations (degree three), often requiring advanced techniques and sometimes resulting in complex solutions.
Our specific equation, x⁴ + 29x² + 100 = 0, is a special case of a quartic equation. Notice that the coefficients of the x³ and x terms are both zero. This simplified form significantly streamlines the solution process.
Method 1: Solving by Factoring (Quadratic in disguise)
The key to solving x⁴ + 29x² + 100 = 0 efficiently lies in recognizing its structure. Observe that the equation is actually a quadratic equation in disguise. Let's substitute 'y' for x²:
y = x²
Substituting this into our original equation yields:
y² + 29y + 100 = 0
This is a standard quadratic equation, which we can solve using various techniques, such as factoring, the quadratic formula, or completing the square. Factoring is often the quickest method if the factors are easily discernible. In this case, we look for two numbers that add up to 29 and multiply to 100. These numbers are 4 and 25. Therefore, we can factor the quadratic equation as:
(y + 4)(y + 25) = 0
This gives us two possible solutions for 'y':
- y = -4
- y = -25
Now, we need to substitute back x² for 'y' to find the values of 'x':
- x² = -4
- x² = -25
These equations reveal that the solutions for 'x' will involve imaginary numbers since we're taking the square root of negative numbers.
- x = ±√(-4) = ±2i (where 'i' is the imaginary unit, √-1)
- x = ±√(-25) = ±5i
Therefore, the complete solution set for the quartic equation x⁴ + 29x² + 100 = 0 is {2i, -2i, 5i, -5i}.
Method 2: Using the Quadratic Formula
If factoring isn't immediately apparent, the quadratic formula provides a reliable method for solving quadratic equations. The quadratic formula is:
x = (-b ± √(b² - 4ac)) / 2a
For our quadratic equation y² + 29y + 100 = 0, a = 1, b = 29, and c = 100. Plugging these values into the formula, we get:
y = (-29 ± √(29² - 4 * 1 * 100)) / 2 * 1
y = (-29 ± √(841 - 400)) / 2
y = (-29 ± √441) / 2
y = (-29 ± 21) / 2
This gives us the same solutions for 'y' as before: y = -4 and y = -25. Following the steps outlined in Method 1, we arrive at the same solution set for 'x': {2i, -2i, 5i, -5i}.
Exploring Complex Numbers
The solutions to our quartic equation involve complex numbers, specifically imaginary numbers. Complex numbers are numbers of the form a + bi, where 'a' and 'b' are real numbers, and 'i' is the imaginary unit (√-1). Complex numbers are essential in various fields, including electrical engineering, quantum mechanics, and signal processing.
The presence of complex solutions highlights an important property of quartic equations: they can have both real and complex roots. In this particular case, all roots are purely imaginary (i.e., the real part is zero).
Significance and Applications
While this specific equation might seem abstract, understanding the techniques used to solve it has far-reaching implications. The ability to manipulate and solve polynomial equations is fundamental to many branches of mathematics and science. Applications include:
- Engineering: Solving equations similar to this arises in various engineering problems, such as analyzing electrical circuits or modeling mechanical systems.
- Physics: Many physical phenomena can be described using polynomial equations, and solving them is crucial for understanding and predicting these phenomena.
- Computer Science: Algorithms and numerical methods used in computer science rely heavily on the ability to solve polynomial equations efficiently.
- Economics and Finance: Models in economics and finance often involve polynomial equations, particularly in areas like optimization and risk management.
Further Exploration: Higher-Degree Polynomials
The techniques used to solve our quartic equation provide a foundation for understanding how to approach even higher-degree polynomial equations. While closed-form solutions exist for cubic and quartic equations, finding solutions for polynomials of degree five or higher typically requires numerical methods (approximation techniques). These numerical methods use iterative processes to find increasingly accurate approximations of the roots.
Conclusion: Mastering the Art of Polynomial Solution
The equation x⁴ + 29x² + 100 = 0, while seemingly simple, offers a valuable opportunity to explore and reinforce key concepts in algebra. By employing factoring or the quadratic formula, we successfully solved this quartic equation, revealing the elegant yet complex nature of its solutions. Understanding such methods is crucial for tackling more challenging mathematical problems in various disciplines, highlighting the fundamental importance of polynomial equations in mathematics and its applications across numerous fields. The journey of solving this seemingly simple equation unveils a wealth of mathematical depth and utility. Remember, consistent practice and a thorough understanding of fundamental principles are key to mastering these techniques.
Latest Posts
Latest Posts
-
What Is The Net Gain Of Atp In Glycolysis
Mar 31, 2025
-
What Is The Opposite Word Of Beautiful
Mar 31, 2025
-
Which Of The Following Is Not A Planet
Mar 31, 2025
-
How To Find Valence Electrons Of Transition Elements
Mar 31, 2025
-
An Account Is Said To Have A Debit Balance If
Mar 31, 2025
Related Post
Thank you for visiting our website which covers about X 4 29x 2 100 0 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
