What Is Equivalent Fraction Of 4/5
News Leon
Apr 02, 2025 · 5 min read
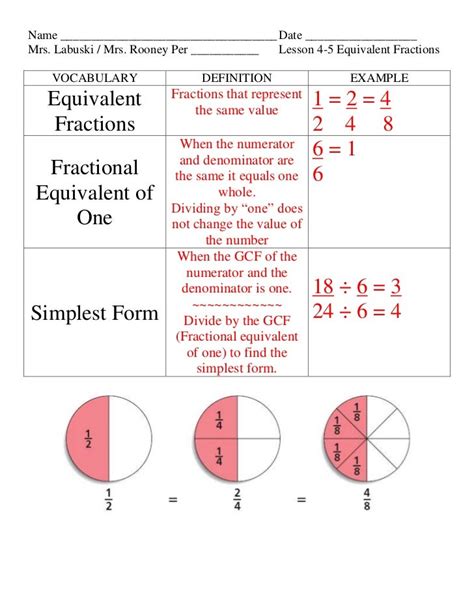
Table of Contents
- What Is Equivalent Fraction Of 4/5
- Table of Contents
- What is the Equivalent Fraction of 4/5? A Comprehensive Guide
- Understanding Equivalent Fractions
- Finding Equivalent Fractions of 4/5
- Multiplying by 2:
- Multiplying by 3:
- Multiplying by 4:
- Multiplying by any whole number 'n':
- Simplifying Fractions and Finding Equivalent Fractions
- Visualizing Equivalent Fractions
- Practical Applications of Equivalent Fractions
- Cooking and Baking:
- Construction and Engineering:
- Finance and Budgeting:
- Data Analysis and Statistics:
- Sewing and Tailoring:
- Beyond Basic Equivalent Fractions: Decimals and Percentages
- Addressing Common Misconceptions
- Conclusion: The Power of Equivalent Fractions
- Latest Posts
- Latest Posts
- Related Post
What is the Equivalent Fraction of 4/5? A Comprehensive Guide
Understanding equivalent fractions is fundamental to mastering mathematics, especially in areas like algebra, geometry, and calculus. This comprehensive guide delves into the concept of equivalent fractions, focusing specifically on finding equivalent fractions for 4/5. We'll explore various methods, provide numerous examples, and discuss the practical applications of this crucial mathematical concept.
Understanding Equivalent Fractions
Equivalent fractions represent the same proportion or value, even though they look different. Think of it like slicing a pizza: a pizza cut into 8 slices with 4 slices taken is the same as a pizza cut into 4 slices with 2 slices taken. Both represent half the pizza. This is the essence of equivalent fractions. They have different numerators and denominators, but their ratios remain identical.
Key Concept: To obtain an equivalent fraction, you multiply or divide both the numerator (top number) and the denominator (bottom number) by the same non-zero number. This maintains the ratio and, therefore, the value of the fraction.
Finding Equivalent Fractions of 4/5
The fraction 4/5 represents four parts out of a total of five equal parts. To find an equivalent fraction, we need to multiply both the numerator and denominator by the same number. Let's explore some examples:
Multiplying by 2:
- Numerator: 4 x 2 = 8
- Denominator: 5 x 2 = 10
- Equivalent Fraction: 8/10
Therefore, 8/10 is an equivalent fraction of 4/5. Both represent the same proportion – 80% or 0.8 in decimal form.
Multiplying by 3:
- Numerator: 4 x 3 = 12
- Denominator: 5 x 3 = 15
- Equivalent Fraction: 12/15
This demonstrates another equivalent fraction: 12/15. Again, this represents the same value as 4/5.
Multiplying by 4:
- Numerator: 4 x 4 = 16
- Denominator: 5 x 4 = 20
- Equivalent Fraction: 16/20
We can continue this process indefinitely, generating an infinite number of equivalent fractions for 4/5. Each resulting fraction will maintain the same proportional value.
Multiplying by any whole number 'n':
- Numerator: 4 x n = 4n
- Denominator: 5 x n = 5n
- Equivalent Fraction: 4n/5n
This general formula showcases how any whole number, 'n', can be used to create an equivalent fraction for 4/5.
Simplifying Fractions and Finding Equivalent Fractions
While we can create countless equivalent fractions by multiplying, we can also find equivalent fractions by simplifying. Simplifying means reducing a fraction to its lowest terms, where the numerator and denominator share no common factors other than 1. This is particularly useful when dealing with larger numbers.
Let's consider a hypothetical equivalent fraction of 4/5, such as 20/25. To simplify, we find the greatest common divisor (GCD) of the numerator (20) and the denominator (25). The GCD of 20 and 25 is 5.
- Divide Numerator: 20 ÷ 5 = 4
- Divide Denominator: 25 ÷ 5 = 5
- Simplified Fraction: 4/5
This demonstrates that 20/25 simplifies to 4/5, confirming it as an equivalent fraction. This simplification process helps in comparing and working with fractions more efficiently.
Visualizing Equivalent Fractions
Visual representations can greatly aid understanding. Imagine a rectangle divided into 5 equal parts, with 4 shaded. This visually represents 4/5. Now, imagine the same rectangle, but divided into 10 equal parts, with 8 shaded. This represents 8/10. Both visually depict the same proportion of the rectangle being shaded, highlighting the equivalence. You can apply this visual approach to other equivalent fractions of 4/5 like 12/15, 16/20, and so on. Each representation will show the same proportional area shaded, reinforcing the concept of equivalence.
Practical Applications of Equivalent Fractions
Equivalent fractions are not merely an abstract mathematical concept; they have widespread practical applications in many fields.
Cooking and Baking:
Recipes often require adjusting ingredient quantities. If a recipe calls for 4/5 of a cup of flour, and you want to double the recipe, you'd need 8/10 of a cup (an equivalent fraction). Scaling recipes up or down frequently involves working with equivalent fractions.
Construction and Engineering:
Blueprints and scale models rely heavily on proportions. Converting between different scale measurements often requires finding equivalent fractions to maintain accuracy and consistency.
Finance and Budgeting:
Understanding proportions of income and expenses utilizes equivalent fractions for budgeting, comparing financial ratios, and analyzing investment returns.
Data Analysis and Statistics:
Presenting data in various forms often necessitates converting between fractions. For example, expressing a survey result as both a fraction (4/5) and a percentage (80%) involves using equivalent fractions.
Sewing and Tailoring:
Pattern adjustments and fabric calculations rely on precise measurements and ratios. Using equivalent fractions ensures accurate sizing and proportions.
Beyond Basic Equivalent Fractions: Decimals and Percentages
Equivalent fractions also connect to decimals and percentages, providing additional ways to represent the same value.
- Decimal: 4/5 is equivalent to 0.8 (divide the numerator by the denominator).
- Percentage: 0.8 is equivalent to 80% (multiply the decimal by 100).
Understanding this interconnectedness allows for flexibility in representing and manipulating proportions in various contexts.
Addressing Common Misconceptions
A common misconception is that only multiplying the numerator and denominator by the same number creates equivalent fractions. While this is the primary method, remember that simplifying a fraction also yields an equivalent fraction. The key is that the ratio between the numerator and denominator remains unchanged.
Conclusion: The Power of Equivalent Fractions
Understanding equivalent fractions is a cornerstone of mathematical literacy. This guide has explored various methods for finding equivalent fractions for 4/5, highlighting their practical applications across diverse fields. Mastering this concept opens doors to a deeper understanding of mathematical concepts and enhances problem-solving abilities in everyday situations and professional settings. The ability to manipulate and simplify fractions is invaluable in numerous practical contexts, making it a crucial skill to develop. By understanding the interconnectedness of fractions, decimals, and percentages, you equip yourself with a powerful toolkit for tackling a wide range of mathematical challenges. Remember that practice is key – the more you work with equivalent fractions, the more intuitive and comfortable you will become with the concept.
Latest Posts
Latest Posts
-
Correctly Match The Following Joint Symphysis
Apr 06, 2025
-
The Solid Part Of The Earth
Apr 06, 2025
-
How Many Asymmetric Carbons Are Present In The Compound Below
Apr 06, 2025
-
K2 Cr2 O7 H2 S O4
Apr 06, 2025
-
Why The Electric Field Inside A Conductor Is Zero
Apr 06, 2025
Related Post
Thank you for visiting our website which covers about What Is Equivalent Fraction Of 4/5 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
