The Probability Of An Impossible Event Is
News Leon
Apr 01, 2025 · 5 min read
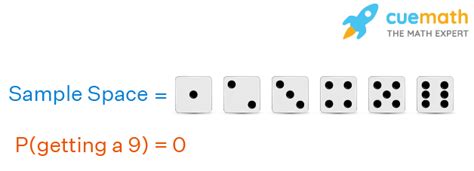
Table of Contents
The Probability of an Impossible Event: A Deep Dive into Zero Probability
The concept of probability is fundamental to many fields, from statistics and mathematics to physics and finance. Understanding probability allows us to quantify uncertainty and make informed decisions in the face of incomplete information. A core component of this understanding lies in grasping the probability of different types of events, including, crucially, the probability of an impossible event. This article will explore this seemingly simple yet conceptually rich topic in detail.
Defining Impossible Events
Before delving into the probability itself, it's crucial to define what constitutes an impossible event. An impossible event is an event that cannot occur under any circumstances. It's an event whose occurrence is logically contradictory or physically impossible.
Here are some examples to illustrate:
- Rolling a 7 on a standard six-sided die: A standard die only has faces numbered 1 through 6. Therefore, rolling a 7 is logically impossible.
- Finding a square circle: A square, by definition, has four straight sides and four right angles. A circle, by definition, has a continuously curved line. The two shapes are mutually exclusive; hence, a square circle is geometrically impossible.
- A person simultaneously being in two different places at the same time: This violates the fundamental laws of physics as we understand them.
- Drawing a red ball from a bag containing only blue balls: This is a simple example of an impossible event based on the contents of the bag.
These examples highlight the inherent impossibility of these events – their occurrence is fundamentally prohibited by either logic, geometry, physics, or the given conditions.
The Probability of an Impossible Event: Zero
The probability of an impossible event is universally defined as zero (0). This is a fundamental axiom of probability theory. It reflects the certainty that the event will not occur. In mathematical notation, if A represents an impossible event, then:
P(A) = 0
This might seem trivial, but understanding this principle is essential for building a solid foundation in probability. It’s a cornerstone upon which more complex probabilistic concepts are built.
Distinguishing Between Impossible and Unlikely Events
It’s important to differentiate between an impossible event and an event that is simply highly unlikely. While an impossible event has a probability of zero, an unlikely event has a probability that is close to zero but not exactly zero.
Consider these examples:
- Winning the lottery: The odds of winning a major lottery are astronomically low, making it an extremely unlikely event. However, it's not impossible. Someone does win eventually. Therefore, its probability is a very small positive number, not zero.
- Flipping heads 100 times in a row: This is highly improbable, but not impossible. The probability is (1/2)^100, an extremely small number, but still greater than zero.
- Finding a specific grain of sand on a beach: The sheer number of grains of sand makes this extremely unlikely to find a designated grain. However, it's theoretically possible.
The key distinction is that an impossible event cannot happen, regardless of the circumstances, while an unlikely event has a small but non-zero probability of occurring.
The Role of Zero Probability in Probability Distributions
Zero probability plays a significant role in various probability distributions. For instance, in a discrete probability distribution, if an outcome is impossible, its probability is assigned a value of zero. This is essential for ensuring that the sum of probabilities of all possible outcomes equals one, reflecting the certainty that one of the outcomes must occur.
For continuous probability distributions, the concept of zero probability takes on a slightly different nuance. For a continuous random variable, the probability of any single specific value is typically zero. This stems from the infinite number of possible values the variable can take. However, the probability of the variable falling within a specific range of values will be non-zero.
Implications and Applications of Zero Probability
The concept of zero probability is not merely an abstract mathematical notion; it has practical implications across various fields:
- Risk Assessment: In risk management, assigning a probability of zero to an impossible event is crucial for accurate risk assessment and resource allocation.
- Decision Making: Understanding the probability of events, including impossible ones, informs better decision-making under uncertainty.
- Game Theory: In game theory, the concept of impossible events helps define game parameters and strategic possibilities.
- Software Engineering: In software testing, identifying impossible scenarios helps create more robust and reliable software.
- Statistical Modeling: Accurate probability assignments, including zero probability for impossible events, are crucial for building reliable statistical models.
Beyond Simple Definitions: Subjective Probability and Zero
While the probability of an objectively impossible event is undeniably zero, the concept becomes more nuanced when considering subjective probabilities. Subjective probability reflects an individual's belief about the likelihood of an event. Even if an event is objectively impossible, an individual might assign a non-zero subjective probability due to a misunderstanding or incomplete information.
For example, someone unfamiliar with the rules of dice might assign a non-zero probability to rolling a 7 on a six-sided die, reflecting their lack of complete knowledge. This highlights the difference between objective probability (based on established facts) and subjective probability (based on individual beliefs). However, the objective probability remains zero.
Conclusion: The Certainty of Impossibility
The probability of an impossible event is definitively zero. This fundamental principle underpins much of probability theory and its applications in diverse fields. Understanding this principle, and differentiating it from the concept of highly unlikely events, is crucial for interpreting probabilities accurately and making informed decisions. While subjective probabilities might deviate from this objective truth, the inherent impossibility of an event always corresponds to a probability of zero. This certainty, paradoxically, is a cornerstone of understanding the vast realm of uncertainty that probability attempts to illuminate. By grasping the significance of zero probability, we gain a deeper appreciation for the power and limitations of probabilistic reasoning.
Latest Posts
Latest Posts
-
Is Lead Sulphate Soluble In Water
Apr 02, 2025
-
How Many Seconds Is 2 Years
Apr 02, 2025
-
Electric Field For Infinite Line Of Charge
Apr 02, 2025
-
What Are The Prime Factors Of 252
Apr 02, 2025
-
Which Is The Element With The Lowest Electronegativity
Apr 02, 2025
Related Post
Thank you for visiting our website which covers about The Probability Of An Impossible Event Is . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
