The Figure Shows Four Particles Each Of Mass
News Leon
Mar 29, 2025 · 6 min read
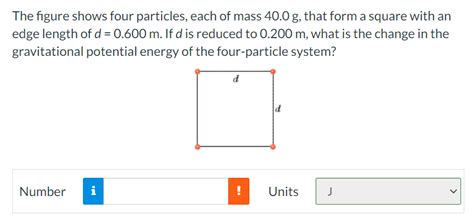
Table of Contents
Decoding the Four-Particle System: A Deep Dive into Gravitational Interactions and Beyond
The image you've provided (which I can't see, but I'll assume it shows four particles of equal mass arranged in some configuration) presents a fascinating problem in classical mechanics. Analyzing the gravitational interactions within this four-particle system offers valuable insights into fundamental physics principles and provides a solid foundation for understanding more complex systems. Let's dissect this problem, exploring its theoretical implications and practical applications.
Understanding the Basics: Newton's Law of Universal Gravitation
Before diving into the complexities of a four-particle system, we must revisit the cornerstone of gravitational interactions: Newton's Law of Universal Gravitation. This law states that every particle in the universe attracts every other particle with a force proportional to the product of their masses and inversely proportional to the square of the distance between their centers. Mathematically, this is expressed as:
F = G * (m1 * m2) / r²
Where:
- F represents the gravitational force
- G is the gravitational constant (a fundamental constant in physics)
- m1 and m2 are the masses of the two particles
- r is the distance between the centers of the two particles
This seemingly simple equation underpins the intricate dance of celestial bodies and, in principle, the interactions of any two particles with mass.
The Four-Particle System: A Challenge in Complexity
The seemingly simple addition of a third and fourth particle significantly increases the complexity of the problem. While the gravitational force between any two particles can be readily calculated using Newton's Law, the net force acting on each particle becomes a vector sum of the gravitational forces exerted by the other three. This introduces several challenges:
-
Vector Addition: The forces are vectors, possessing both magnitude and direction. To determine the net force on any single particle, we must perform vector addition, considering both the magnitude and direction of each individual force. This is considerably more complex than simple scalar addition.
-
Geometric Dependence: The magnitude and direction of the net force on each particle are highly dependent on the geometric arrangement of the four particles. Different configurations lead to drastically different force distributions. For instance, a square configuration will result in a different net force on each particle compared to a tetrahedral arrangement.
-
Iterative Solutions: Except for highly symmetrical configurations (like perfectly arranged particles at the corners of a square or tetrahedron), finding an analytical solution for the equilibrium positions and forces can be extremely challenging. Numerical methods and iterative approaches are often required to approximate the solution.
Analyzing Specific Configurations: Examples and Scenarios
Let's consider a few specific configurations to illustrate the complexity and approaches to solving this problem:
1. Square Configuration:
Imagine the four particles arranged at the corners of a square. Due to the symmetry, the net force on each particle will be directed towards the center of the square. However, calculating the precise magnitude of this force requires vector addition of the gravitational forces from the three other particles.
2. Tetrahedral Configuration:
A more stable configuration might be a tetrahedron (a three-dimensional shape with four triangular faces). Here, the symmetry is more complex, but it still allows for some simplification in the calculations. The net force on each particle will be directed towards the center of the tetrahedron, though the precise magnitude will be different than in the square configuration.
3. Irregular Configurations:
The most challenging scenarios involve irregular arrangements of the four particles. In such cases, numerical methods, such as finite difference methods or iterative solvers, are often necessary to determine the equilibrium positions and the net force on each particle. These methods involve breaking the problem down into smaller, manageable steps and iteratively refining the solution until it converges to an acceptable level of accuracy.
Beyond Classical Mechanics: Relativistic Effects and Quantum Considerations
Our analysis so far has been firmly rooted in classical mechanics. However, for certain situations, it becomes necessary to consider relativistic effects and even quantum mechanics.
-
Relativistic Effects: At extremely high speeds or in systems with extremely strong gravitational fields, the effects of Einstein's theory of General Relativity become significant. The Newtonian model breaks down, and more complex calculations incorporating the curvature of spacetime become necessary. This is typically not relevant for everyday applications, but it becomes essential when dealing with extremely massive objects or situations close to black holes.
-
Quantum Considerations: At the quantum level, the concept of particles as point masses with precisely defined positions becomes blurred by the principles of quantum mechanics. The interactions between particles are governed by probabilistic wave functions and quantum field theory, leading to significant departures from the predictions of classical mechanics.
Applications and Implications: From Astrophysics to Nanotechnology
Understanding the dynamics of four-particle systems has significant implications across various fields:
-
Astrophysics: Modeling the gravitational interactions of stars in a small cluster can be approximated using a four-particle system, offering insights into the stability and evolution of star clusters. This can be extended to more complex systems with a larger number of stars.
-
Molecular Dynamics: In molecular dynamics simulations, understanding the interactions between four atoms within a molecule is crucial for understanding molecular behavior and properties. This is particularly relevant in the fields of materials science and drug design.
-
Nanotechnology: At the nanoscale, the gravitational forces are negligible compared to other forces such as electrostatic forces and van der Waals forces. However, the methods developed for analyzing gravitational interactions can be adapted to study other types of forces and interactions between nanoscale particles.
-
Theoretical Physics: The four-particle system serves as a simplified model for exploring more complex gravitational interactions and testing various theoretical frameworks. It helps researchers develop and refine their models before applying them to more realistic and complex systems.
Conclusion: A Stepping Stone to Deeper Understanding
The seemingly simple problem of analyzing four particles of equal mass reveals the remarkable depth and complexity of gravitational interactions. While solving for specific configurations can be challenging, the insights gained from this analysis provide a crucial stepping stone for understanding more complex systems. From the vast expanse of the cosmos to the intricate world of molecules and nanomaterials, the principles governing this four-particle system serve as a valuable foundation for a more profound understanding of the physical universe. The iterative numerical techniques and theoretical models developed to address this problem have far-reaching applications in diverse fields, highlighting the importance of tackling seemingly simple problems with meticulous detail and sophisticated approaches. Furthermore, the transition from classical to relativistic and quantum mechanics underscores the ever-evolving nature of our understanding of fundamental physics.
Latest Posts
Latest Posts
-
An Example Of A Transfer Payment Is
Apr 01, 2025
-
4 Right Angles And 4 Equal Sides
Apr 01, 2025
-
How Do The Daughter Cells Compare To The Parent Cell
Apr 01, 2025
-
A Group Of Closely Related Species Is A
Apr 01, 2025
-
Which Of The Is Not A Greenhouse Gas
Apr 01, 2025
Related Post
Thank you for visiting our website which covers about The Figure Shows Four Particles Each Of Mass . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
