Balance Equation Fes2 O2 Fe2o3 So2
News Leon
Apr 02, 2025 · 6 min read
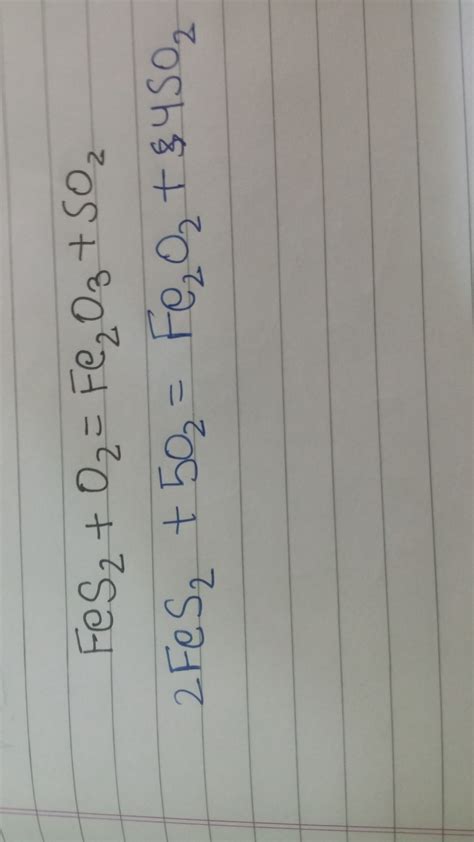
Table of Contents
Balancing the Equation: FeS₂ + O₂ → Fe₂O₃ + SO₂ – A Deep Dive into Stoichiometry
The chemical equation FeS₂ + O₂ → Fe₂O₃ + SO₂ represents the crucial process of pyrite oxidation, a reaction with significant implications in various fields, from environmental science to industrial metallurgy. Understanding and balancing this equation is fundamental to grasping the stoichiometry of the reaction and its practical applications. This article will delve into the intricacies of balancing this equation, exploring different methods and highlighting the importance of balanced chemical equations in chemical calculations and real-world scenarios.
Understanding the Reaction: Pyrite Oxidation
Before diving into the balancing process, let's understand the chemical species involved:
- FeS₂ (Iron Pyrite or Fool's Gold): This is an iron sulfide mineral, a common source of iron and sulfur. Its characteristic cubic crystalline structure and brassy yellow color often lead to misidentification as gold.
- O₂ (Oxygen): The oxidizing agent in this reaction, crucial for the oxidation of iron and sulfur.
- Fe₂O₃ (Iron(III) Oxide or Hematite): The iron-containing product, a common iron ore. Its reddish-brown color is often observed in rusty surfaces.
- SO₂ (Sulfur Dioxide): The sulfur-containing product, a pungent and toxic gas with significant environmental consequences. It's a major contributor to acid rain.
The reaction itself represents the oxidation of iron pyrite by oxygen. This is an exothermic reaction, meaning it releases heat, and often occurs naturally in the environment, particularly in the presence of water and microorganisms. This process is responsible for the formation of acid mine drainage, a major environmental concern associated with mining activities.
Balancing the Equation: Methods and Steps
Balancing chemical equations ensures that the number of atoms of each element is the same on both the reactant (left) and product (right) sides. This adheres to the Law of Conservation of Mass, stating that matter cannot be created or destroyed in a chemical reaction. Several methods can be used to balance the equation FeS₂ + O₂ → Fe₂O₃ + SO₂. Let's explore two common approaches:
Method 1: Inspection Method
This method involves systematically adjusting the coefficients (numbers in front of the chemical formulas) until the number of atoms of each element is equal on both sides. It's often a trial-and-error process, but with practice, it becomes efficient.
-
Balance Iron (Fe): There are 2 iron atoms on the product side (Fe₂O₃), so we need 2 FeS₂ on the reactant side:
2FeS₂ + O₂ → Fe₂O₃ + SO₂
-
Balance Sulfur (S): We now have 4 sulfur atoms on the reactant side (2 FeS₂ × 2 S/FeS₂ = 4 S). Therefore, we need 4 SO₂ molecules on the product side:
2FeS₂ + O₂ → Fe₂O₃ + 4SO₂
-
Balance Oxygen (O): There are 11 oxygen atoms on the product side (3 from Fe₂O₃ and 8 from 4SO₂). To balance this, we need 11/2 O₂ molecules on the reactant side:
2FeS₂ + 11/2O₂ → Fe₂O₃ + 4SO₂
-
Eliminate Fractions: To obtain whole-number coefficients, we multiply the entire equation by 2:
4FeS₂ + 11O₂ → 2Fe₂O₃ + 8SO₂
This is the balanced chemical equation.
Method 2: Algebraic Method
This more systematic method uses variables to represent the coefficients and solves a system of equations.
-
Assign Variables: Assign variables to each coefficient:
aFeS₂ + bO₂ → cFe₂O₃ + dSO₂
-
Set up Equations: Based on the number of atoms of each element, we can set up a system of equations:
- Iron (Fe): a = 2c
- Sulfur (S): 2a = d
- Oxygen (O): 2b = 3c + 2d
-
Solve the System of Equations: We can solve this system of equations using substitution or elimination. Let's use substitution. From the first equation, a = 2c. Substituting this into the second equation, we get 4c = d. Substituting these into the third equation, we have 2b = 3c + 2(4c) = 11c. Therefore, b = 11c/2.
-
Choose a Value for a Variable: Let's choose c = 2. This simplifies the calculations. Then a = 4, d = 8, and b = 11.
-
Write the Balanced Equation: Substituting these values back into the original equation, we obtain:
4FeS₂ + 11O₂ → 2Fe₂O₃ + 8SO₂
This confirms the balanced equation obtained using the inspection method.
Importance of Balanced Chemical Equations
Balanced chemical equations are essential for several reasons:
-
Stoichiometric Calculations: They allow accurate predictions of the amounts of reactants needed and products formed in a chemical reaction. This is crucial in industrial processes, where precise control of reactant quantities is essential for efficiency and safety.
-
Understanding Reaction Ratios: They reveal the molar ratios between reactants and products. In our example, 4 moles of FeS₂ react with 11 moles of O₂ to produce 2 moles of Fe₂O₃ and 8 moles of SO₂.
-
Environmental Impact Assessment: Balanced equations help assess the environmental impact of reactions. The production of SO₂ in pyrite oxidation highlights the potential for air pollution and acid rain. Understanding the stoichiometry allows for the development of mitigation strategies.
-
Industrial Applications: The balanced equation is vital in optimizing industrial processes involving pyrite oxidation. For example, in the extraction of iron from pyrite, the precise control of oxygen supply is critical for maximizing iron yield and minimizing SO₂ emissions.
-
Research and Development: Accurate stoichiometry is crucial in research settings for designing experiments and interpreting results. It ensures reproducibility and allows for the accurate comparison of data across different studies.
Real-World Applications and Environmental Considerations
The reaction of pyrite oxidation has significant real-world implications:
-
Acid Mine Drainage (AMD): The oxidation of pyrite in mines is a primary cause of AMD. The SO₂ produced reacts with water to form sulfuric acid, which contaminates surrounding waterways, impacting aquatic life and potentially harming human health.
-
Metal Extraction: Pyrite is a significant source of iron and other metals. Understanding the stoichiometry of its oxidation is vital for efficient and environmentally responsible metal extraction processes.
-
Coal Combustion: Pyrite is often present in coal. During coal combustion, pyrite oxidation contributes to SO₂ emissions, a major air pollutant. The balanced equation helps engineers design pollution control systems to minimize these emissions.
-
Geochemical Processes: Pyrite oxidation plays a significant role in various geochemical processes, such as weathering and soil formation. Understanding the stoichiometry aids in modeling these natural processes.
Conclusion
Balancing the equation FeS₂ + O₂ → Fe₂O₃ + SO₂ is a fundamental exercise in stoichiometry with profound real-world implications. Whether using the inspection method or the algebraic approach, achieving a balanced equation – 4FeS₂ + 11O₂ → 2Fe₂O₃ + 8SO₂ – is crucial for understanding the quantitative relationships within this reaction. This understanding is paramount for various applications, from minimizing environmental damage associated with pyrite oxidation to optimizing industrial processes involving this important reaction. The ability to balance chemical equations is a cornerstone skill in chemistry, offering a foundation for further exploration into the fascinating world of chemical reactions and their impact on our environment and society.
Latest Posts
Latest Posts
-
Which Of The Following Is Not Found In Blood Plasma
Apr 03, 2025
-
Compare The Pectoral And Pelvic Girdles
Apr 03, 2025
-
Find The Values Of X And Y In Parallelogram Pqrs
Apr 03, 2025
-
Select The Correct Statement About Plant Life Cycles
Apr 03, 2025
-
What Is The Molar Mass Of Phosphoric Acid
Apr 03, 2025
Related Post
Thank you for visiting our website which covers about Balance Equation Fes2 O2 Fe2o3 So2 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
