Write 2 6 In Lowest Terms.
News Leon
Apr 04, 2025 · 5 min read
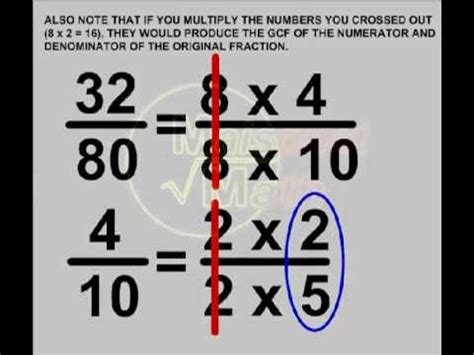
Table of Contents
Write 2/6 in Lowest Terms: A Comprehensive Guide to Fraction Simplification
Understanding fractions is a fundamental concept in mathematics, forming the building blocks for more advanced topics. Simplifying fractions, or reducing them to their lowest terms, is a crucial skill. This article will delve deep into the process of simplifying the fraction 2/6, exploring the underlying principles and extending the concept to other fractions. We'll cover various methods, practical examples, and even touch upon the real-world applications of fraction simplification.
Understanding Fractions: A Quick Recap
Before we jump into simplifying 2/6, let's quickly review the basics of fractions. A fraction represents a part of a whole. It's composed of two parts:
- Numerator: The top number represents the number of parts we have.
- Denominator: The bottom number represents the total number of equal parts the whole is divided into.
In the fraction 2/6, 2 is the numerator and 6 is the denominator. This means we have 2 parts out of a possible 6 equal parts.
Finding the Greatest Common Divisor (GCD)
The key to simplifying fractions lies in finding the Greatest Common Divisor (GCD), also known as the Greatest Common Factor (GCF), of the numerator and the denominator. The GCD is the largest number that divides both the numerator and the denominator without leaving a remainder.
Several methods can be used to find the GCD:
1. Listing Factors
This method involves listing all the factors of both the numerator and the denominator, then identifying the largest factor they have in common.
Factors of 2: 1, 2 Factors of 6: 1, 2, 3, 6
The largest common factor is 2.
2. Prime Factorization
This method breaks down both the numerator and the denominator into their prime factors. The GCD is the product of the common prime factors raised to the lowest power.
Prime factorization of 2: 2 Prime factorization of 6: 2 x 3
The common prime factor is 2. Therefore, the GCD is 2.
3. Euclidean Algorithm
This is a more efficient method for larger numbers. It involves repeatedly dividing the larger number by the smaller number and replacing the larger number with the remainder until the remainder is 0. The last non-zero remainder is the GCD.
Let's apply the Euclidean Algorithm to 2 and 6:
6 ÷ 2 = 3 with a remainder of 0.
The last non-zero remainder is 2, so the GCD is 2.
Simplifying 2/6 to Lowest Terms
Now that we've found the GCD of 2 and 6 (which is 2), we can simplify the fraction:
Divide both the numerator and the denominator by the GCD:
2 ÷ 2 = 1 6 ÷ 2 = 3
Therefore, 2/6 simplified to its lowest terms is 1/3.
Visual Representation
Imagine a pizza cut into 6 equal slices. The fraction 2/6 represents having 2 slices out of 6. If we group the slices into pairs, we have one pair of slices out of three possible pairs. This visually demonstrates that 2/6 is equivalent to 1/3.
Extending the Concept: Simplifying Other Fractions
The process of simplifying fractions using the GCD applies to all fractions. Let's look at a few more examples:
Example 1: Simplify 12/18
Factors of 12: 1, 2, 3, 4, 6, 12 Factors of 18: 1, 2, 3, 6, 9, 18
GCD = 6
12 ÷ 6 = 2 18 ÷ 6 = 3
Therefore, 12/18 simplifies to 2/3.
Example 2: Simplify 24/36
Prime factorization of 24: 2 x 2 x 2 x 3 Prime factorization of 36: 2 x 2 x 3 x 3
Common prime factors: 2 x 2 x 3 = 12
GCD = 12
24 ÷ 12 = 2 36 ÷ 12 = 3
Therefore, 24/36 simplifies to 2/3.
Example 3: Simplify 15/25
GCD of 15 and 25 is 5.
15 ÷ 5 = 3 25 ÷ 5 = 5
Therefore, 15/25 simplifies to 3/5.
Example 4: Simplify 4/16
GCD of 4 and 16 is 4.
4 ÷ 4 = 1 16 ÷ 4 = 4
Therefore, 4/16 simplifies to 1/4.
Example 5: Simplify 10/100
GCD of 10 and 100 is 10.
10 ÷ 10 = 1 100 ÷ 10 = 10
Therefore, 10/100 simplifies to 1/10.
Real-World Applications of Fraction Simplification
Simplifying fractions isn't just an academic exercise; it has practical applications in various fields:
-
Cooking and Baking: Recipes often use fractions. Simplifying fractions helps in understanding and adjusting ingredient quantities.
-
Construction and Engineering: Accurate measurements are crucial. Simplifying fractions ensures precision in calculations and blueprints.
-
Finance: Working with percentages and proportions frequently involves fraction simplification.
-
Data Analysis: Simplifying fractions helps in presenting data clearly and concisely.
Beyond Simplification: Equivalent Fractions
Understanding that simplifying a fraction doesn't change its value is essential. 2/6, 1/3, 4/12, and 6/18 are all equivalent fractions; they represent the same portion of a whole. Simplifying a fraction simply represents it in its most concise form.
Conclusion: Mastering Fraction Simplification
Mastering the art of simplifying fractions is crucial for success in mathematics and its various applications. By understanding the concept of the Greatest Common Divisor and employing different methods to find it, you can effectively reduce fractions to their lowest terms. Remember, simplification makes fractions easier to understand, compare, and use in calculations. Practice regularly with different fractions to build your proficiency and confidence in this fundamental mathematical skill. This comprehensive guide provides a solid foundation for tackling more complex fraction operations in the future.
Latest Posts
Latest Posts
-
Predict The Product S For The Following Reaction
Apr 11, 2025
-
A Bundle Of Axons In The Cns Is Called
Apr 11, 2025
-
Which Of The Following Statements Is True About Iron
Apr 11, 2025
-
Why Sound Waves Are Called Mechanical Waves
Apr 11, 2025
-
Why Is Fluorine A Better Oxidizer Than Oxygen
Apr 11, 2025
Related Post
Thank you for visiting our website which covers about Write 2 6 In Lowest Terms. . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.