Which Pair Of Triangles Must Be Similar
News Leon
Apr 01, 2025 · 6 min read
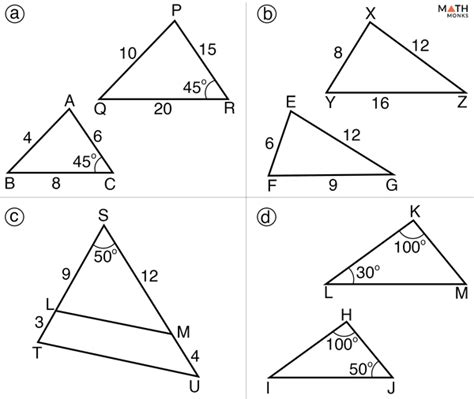
Table of Contents
Which Pairs of Triangles Must Be Similar? A Comprehensive Guide
Similar triangles are a fundamental concept in geometry, with far-reaching applications in various fields, from architecture and engineering to computer graphics and cartography. Understanding which pairs of triangles are definitively similar is crucial for solving numerous geometric problems and grasping the underlying principles of scaling and proportionality. This in-depth guide explores the different criteria that guarantee triangle similarity, providing clear explanations, illustrative examples, and practical applications.
Understanding Triangle Similarity
Before delving into the specific criteria, let's establish a clear understanding of what constitutes similar triangles. Two triangles are considered similar if their corresponding angles are congruent (equal) and their corresponding sides are proportional. This means that one triangle is essentially a scaled version of the other, with no change in shape. The symbol for similarity is ~. So, if triangle ABC is similar to triangle DEF, we write it as ∆ABC ~ ∆DEF.
Key characteristics of similar triangles:
- Corresponding angles are congruent: ∠A = ∠D, ∠B = ∠E, ∠C = ∠F
- Corresponding sides are proportional: AB/DE = BC/EF = AC/DF. The ratio of corresponding sides is called the scale factor.
It's important to note that if one of these conditions is met, the other automatically follows. This is the essence of triangle similarity theorems.
Criteria for Proving Triangle Similarity
Several postulates and theorems provide foolproof methods to determine if two triangles are similar. Mastering these criteria is essential for efficiently solving geometric problems. Let's explore each in detail:
1. Angle-Angle (AA) Similarity Postulate
This is arguably the most frequently used criterion for proving triangle similarity. The AA postulate states that if two angles of one triangle are congruent to two angles of another triangle, then the triangles are similar. Since the sum of angles in a triangle is always 180°, if two angles are congruent, the third angle must also be congruent.
Why it works: The angles determine the shape of the triangle. If the angles are the same, the triangles must have the same shape, even if they are different sizes.
Example: Consider two triangles, ∆ABC and ∆DEF. If ∠A = ∠D = 50° and ∠B = ∠E = 60°, then ∆ABC ~ ∆DEF by AA similarity.
2. Side-Side-Side (SSS) Similarity Theorem
The SSS similarity theorem states that if the three sides of one triangle are proportional to the three sides of another triangle, then the triangles are similar. This means that the ratio of corresponding sides must be constant.
Example: Let's say the sides of ∆ABC are AB = 6, BC = 8, AC = 10. And the sides of ∆DEF are DE = 3, EF = 4, DF = 5. Since 6/3 = 8/4 = 10/5 = 2, the ratio of corresponding sides is constant (2), therefore ∆ABC ~ ∆DEF by SSS similarity.
3. Side-Angle-Side (SAS) Similarity Theorem
The SAS similarity theorem states that if two sides of one triangle are proportional to two sides of another triangle, and the included angles are congruent, then the triangles are similar. "Included angle" refers to the angle formed by the two sides being compared.
Example: Suppose in ∆ABC, AB = 6, BC = 8, and ∠B = 50°. In ∆DEF, DE = 3, EF = 4, and ∠E = 50°. Since AB/DE = BC/EF = 2 and ∠B = ∠E, then ∆ABC ~ ∆DEF by SAS similarity.
Distinguishing between Similarity and Congruence
It's crucial to differentiate between similar and congruent triangles. While similar triangles have the same shape but can differ in size, congruent triangles are identical in both shape and size. Congruent triangles have congruent corresponding angles and congruent corresponding sides. The symbols for congruence is ≅.
Key Difference Summarized:
| Feature | Similar Triangles | Congruent Triangles |
|---|---|---|
| Shape | Same | Same |
| Size | Different | Same |
| Corresponding Angles | Congruent | Congruent |
| Corresponding Sides | Proportional | Congruent |
| Symbol | ~ | ≅ |
Applications of Similar Triangles
Similar triangles have a broad range of applications across various disciplines:
-
Surveying and Mapping: Surveyors use similar triangles to measure inaccessible distances. By creating similar triangles with known and unknown distances, they can calculate the unknown distances using proportions.
-
Architecture and Engineering: Scaling models are frequently used in architecture and engineering. These models are similar to the actual structures, allowing architects and engineers to test and visualize designs before construction.
-
Computer Graphics: Similar triangles are fundamental to computer graphics techniques like scaling and transforming images. Enlarging or shrinking an image involves creating a similar version of the original image.
-
Astronomy: Astronomers use similar triangles to estimate the distances to stars and planets. By comparing the angles subtended by the celestial object at different locations, they can calculate the distance.
-
Photography: The principles of similar triangles explain how a camera lens projects a three-dimensional world onto a two-dimensional image sensor. The image formed on the sensor is a similar, but scaled-down, version of the scene.
Solving Problems Involving Similar Triangles
To effectively solve problems involving similar triangles, follow these steps:
-
Identify similar triangles: Carefully examine the given information to determine which triangles are similar. Look for congruent angles or proportional sides.
-
Set up proportions: Once you've established similarity, set up proportions using the corresponding sides of the similar triangles.
-
Solve for unknowns: Solve the proportions to find the values of any unknown sides or angles.
Example Problem:
Two triangles, ∆ABC and ∆DEF, are similar. AB = 12, BC = 15, and DE = 8. Find the length of EF.
- Solution: Since ∆ABC ~ ∆DEF, the ratio of corresponding sides is constant. Therefore, AB/DE = BC/EF. Substituting the given values, we get 12/8 = 15/EF. Solving for EF, we find EF = 10.
Advanced Concepts and Further Exploration
While the AA, SSS, and SAS postulates/theorems are the fundamental criteria for proving triangle similarity, exploring more advanced topics can deepen your understanding. These include:
-
Indirect Measurement: This involves using similar triangles to measure distances that are difficult or impossible to measure directly.
-
Trigonometric Ratios in Similar Triangles: Trigonometric ratios (sine, cosine, tangent) are directly related to the ratios of sides in similar right-angled triangles.
-
Similar Triangles and Vectors: Vector geometry utilizes similar triangles in many proofs and derivations, particularly involving projections and transformations.
Conclusion
Understanding which pairs of triangles must be similar is a cornerstone of geometry with broad applications in diverse fields. Mastering the AA, SSS, and SAS criteria provides a powerful toolkit for solving geometric problems and grasping the principles of scaling and proportionality. By applying these principles, you can confidently tackle complex problems and appreciate the elegance and utility of similar triangles. Continued exploration of advanced concepts will further enhance your geometrical abilities and problem-solving skills. Remember to practice regularly with various problem types to solidify your understanding and build your confidence.
Latest Posts
Latest Posts
-
Which Statement Is True About Bacteria
Apr 03, 2025
-
Why Are Fleas Hard To Squish
Apr 03, 2025
-
How Many Chromosomes In A Daughter Cell
Apr 03, 2025
-
Why Do Bones Heal Quicker Than Cartilage
Apr 03, 2025
-
Can Magnitude Be Negative In Physics
Apr 03, 2025
Related Post
Thank you for visiting our website which covers about Which Pair Of Triangles Must Be Similar . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
