What Is The Equivalent Capacitance Of The Combination Shown
News Leon
Apr 06, 2025 · 6 min read
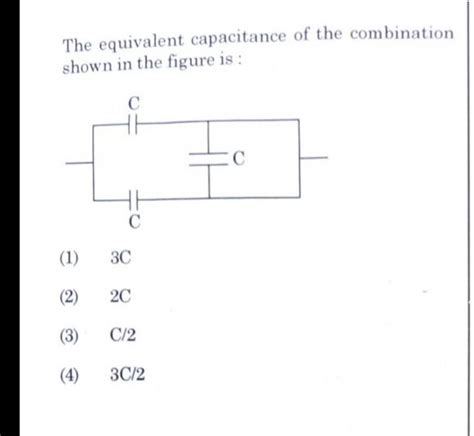
Table of Contents
What is the Equivalent Capacitance of the Combination Shown? A Deep Dive into Series and Parallel Capacitors
Understanding equivalent capacitance is crucial in electronics and circuit analysis. When multiple capacitors are connected together, they effectively act as a single capacitor with an equivalent capacitance. This equivalent capacitance depends entirely on how the capacitors are arranged: in series or in parallel. This article will provide a comprehensive guide to calculating the equivalent capacitance of various capacitor combinations, exploring both series and parallel connections, and delving into more complex scenarios involving combinations of series and parallel arrangements. We'll also touch upon practical applications and troubleshooting.
Understanding Capacitance
Before diving into combinations, let's revisit the fundamental concept of capacitance. Capacitance (C) is the ability of a capacitor to store electrical energy. It's measured in Farads (F), although microfarads (µF), nanofarads (nF), and picofarads (pF) are more commonly used due to the large magnitude of a Farad. A capacitor stores energy by accumulating charge on two conductive plates separated by an insulator (dielectric). The capacitance is directly proportional to the area of the plates and inversely proportional to the distance between them and the dielectric constant of the insulating material.
Key Formula: The basic formula for capacitance is:
C = εA/d
Where:
Cis the capacitanceεis the permittivity of the dielectric materialAis the area of the platesdis the distance between the plates
Equivalent Capacitance: Series Connection
When capacitors are connected in series, the charge on each capacitor is the same, but the voltage across each capacitor is different. This is because the same current flows through each capacitor, and the voltage across each is proportional to its capacitance. The equivalent capacitance (C<sub>eq</sub>) for capacitors connected in series is always less than the smallest individual capacitance. To calculate the equivalent capacitance for n capacitors connected in series, we use the following formula:
1/C<sub>eq</sub> = 1/C₁ + 1/C₂ + 1/C₃ + ... + 1/C<sub>n</sub>
This means we find the reciprocal of each individual capacitance, sum them, and then take the reciprocal of the result to obtain the equivalent capacitance.
Example: Series Capacitor Combination
Let's say we have three capacitors with capacitances of 10 µF, 20 µF, and 30 µF connected in series. To find the equivalent capacitance:
- Find the reciprocals: 1/10 µF = 0.1 µF⁻¹, 1/20 µF = 0.05 µF⁻¹, 1/30 µF = 0.0333 µF⁻¹
- Sum the reciprocals: 0.1 µF⁻¹ + 0.05 µF⁻¹ + 0.0333 µF⁻¹ ≈ 0.1833 µF⁻¹
- Take the reciprocal: 1 / 0.1833 µF⁻¹ ≈ 5.45 µF
Therefore, the equivalent capacitance of the series combination is approximately 5.45 µF. Notice it's smaller than the smallest individual capacitor (10 µF).
Equivalent Capacitance: Parallel Connection
When capacitors are connected in parallel, the voltage across each capacitor is the same, but the charge on each capacitor is different. The total charge stored is the sum of the charges on each capacitor. The equivalent capacitance (C<sub>eq</sub>) for capacitors connected in parallel is simply the sum of the individual capacitances. This is because the total charge stored increases proportionally with the addition of each capacitor.
C<sub>eq</sub> = C₁ + C₂ + C₃ + ... + C<sub>n</sub>
Example: Parallel Capacitor Combination
Consider three capacitors with capacitances of 10 µF, 20 µF, and 30 µF connected in parallel. The equivalent capacitance is:
C<sub>eq</sub> = 10 µF + 20 µF + 30 µF = 60 µF
The equivalent capacitance of the parallel combination is 60 µF, which is larger than any individual capacitor.
Complex Combinations: Series and Parallel Together
Many circuits involve more complex arrangements where capacitors are connected in both series and parallel combinations. To calculate the equivalent capacitance in such cases, we need to break down the circuit into smaller sections, calculating the equivalent capacitance for each section step by step. We start by simplifying the simplest series or parallel combinations first, and then continue combining the simplified sections until we reach a single equivalent capacitance for the entire circuit.
Example: A Mixed Series-Parallel Combination
Imagine a circuit with three capacitors: C₁ = 10 µF, C₂ = 20 µF, and C₃ = 30 µF. C₁ and C₂ are in series, and their combination is in parallel with C₃.
-
Calculate the equivalent capacitance of the series combination (C₁ and C₂):
1/C<sub>eq(series)</sub> = 1/10 µF + 1/20 µF = 0.15 µF⁻¹ C<sub>eq(series)</sub> = 1 / 0.15 µF⁻¹ ≈ 6.67 µF
-
Calculate the equivalent capacitance of the entire circuit (series combination in parallel with C₃):
C<sub>eq(total)</sub> = C<sub>eq(series)</sub> + C₃ = 6.67 µF + 30 µF ≈ 36.67 µF
Therefore, the equivalent capacitance of this mixed series-parallel combination is approximately 36.67 µF.
Practical Applications and Troubleshooting
Understanding equivalent capacitance is essential in various applications:
- Filtering: Capacitors are frequently used in filter circuits to block certain frequencies while allowing others to pass. The equivalent capacitance helps determine the filter's cutoff frequency.
- Energy Storage: In power supplies and energy storage systems, the equivalent capacitance influences the total energy storage capacity.
- Timing Circuits: In timing circuits like oscillators and timers, the equivalent capacitance plays a critical role in determining the timing intervals.
- Signal Processing: Capacitors are used extensively in signal processing circuits, where the equivalent capacitance affects the signal's behavior.
Troubleshooting capacitor circuits often involves checking for faulty capacitors. A faulty capacitor might exhibit a short circuit (zero capacitance), an open circuit (infinite capacitance), or a capacitance value significantly different from its nominal value. Measuring capacitance with a multimeter can help identify faulty components in a circuit. Furthermore, understanding equivalent capacitance is crucial in correctly diagnosing circuit issues when multiple capacitors are involved. Incorrect capacitance values in a combination can lead to malfunctioning circuits and inaccurate outputs.
Advanced Concepts and Considerations
- Tolerance: Capacitors have a tolerance range, meaning their actual capacitance may vary slightly from their marked value. When calculating equivalent capacitance, it's important to consider the tolerance of individual capacitors, especially in applications where precision is critical.
- Dielectric Absorption: Some dielectric materials exhibit dielectric absorption, a phenomenon where a capacitor retains a small amount of charge even after being discharged. This can affect the accuracy of capacitance measurements and calculations in some situations.
- Temperature Dependence: Capacitance can vary slightly with temperature changes. This temperature dependence needs to be considered in applications operating over a wide temperature range.
- Frequency Dependence: At higher frequencies, the parasitic inductance and resistance of the capacitor leads and dielectric can significantly impact its effective capacitance. This is particularly relevant in high-frequency applications.
Conclusion
Calculating the equivalent capacitance of a combination of capacitors is a fundamental concept in electrical engineering and circuit analysis. Mastering the techniques for calculating equivalent capacitance in both series and parallel connections, and handling more complex mixed combinations, is vital for designing, analyzing, and troubleshooting various electronic circuits. This article provides a comprehensive overview of the topic, including practical examples and considerations for real-world applications. Remember that careful attention to detail and consideration of factors like tolerance and frequency dependence are crucial for accurate calculations and successful circuit design.
Latest Posts
Latest Posts
-
What Is The Major Element Found In Chlorophyll
Apr 08, 2025
-
What Is The Electron Configuration For Arsenic
Apr 08, 2025
-
The Elements Found In Chlorophyll Are
Apr 08, 2025
-
What Type Of Rock Makes Up The Continental Crust
Apr 08, 2025
-
What Is The Reciprocal Of 4 9
Apr 08, 2025
Related Post
Thank you for visiting our website which covers about What Is The Equivalent Capacitance Of The Combination Shown . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.