Units For Rate Constant K Third Order
News Leon
Apr 07, 2025 · 5 min read
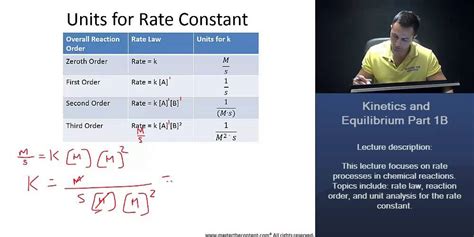
Table of Contents
Units for the Rate Constant k: A Deep Dive into Third-Order Reactions
Understanding the units of the rate constant, k, is crucial for correctly interpreting and applying rate laws in chemical kinetics. While the order of a reaction dictates the overall rate equation, the units of k are directly dependent on this order. This article delves deep into the units of the rate constant for third-order reactions, exploring the underlying principles and providing a comprehensive understanding of their derivation and significance.
Understanding Reaction Order and Rate Laws
Before focusing on third-order reactions, let's establish a firm foundation. The rate law expresses the relationship between the reaction rate and the concentrations of reactants. The overall reaction order is the sum of the exponents of the concentration terms in the rate law. A reaction can be zero-order, first-order, second-order, third-order, or even higher order, depending on its rate law.
First-Order Reactions
For a first-order reaction, A → products, the rate law is:
Rate = k[A]
Here, the rate constant k has units of s⁻¹ (inverse seconds) or time⁻¹. This is because the rate has units of concentration/time (e.g., mol L⁻¹ s⁻¹), and [A] has units of concentration (e.g., mol L⁻¹). Therefore, to maintain dimensional consistency, k must have units of s⁻¹.
Second-Order Reactions
Second-order reactions can have two forms:
- Type 1: 2A → products, with a rate law: Rate = k[A]²
In this case, k has units of L mol⁻¹ s⁻¹ (or M⁻¹s⁻¹, where M represents molarity). This ensures dimensional consistency, as the rate is concentration/time, and [A]² has units of (mol L⁻¹)².
- Type 2: A + B → products, with a rate law: Rate = k[A][B]
The units of k here are also L mol⁻¹ s⁻¹ (or M⁻¹s⁻¹), maintaining consistency with the rate and concentration terms.
Deriving the Units for Third-Order Rate Constants
Now, let's address the main focus: third-order reactions. Similar to second-order reactions, third-order reactions can take several forms, each influencing the units of k.
Third-Order Reactions: Different Forms and their Corresponding Units
Consider the following scenarios:
- Type 1: 3A → products
The rate law is: Rate = k[A]³
To find the units of k, we analyze the dimensions:
- Rate: mol L⁻¹ s⁻¹
- [A]³: (mol L⁻¹)³ = mol³ L⁻³
Therefore, to balance the units:
k = (mol L⁻¹ s⁻¹) / (mol³ L⁻³) = L² mol⁻² s⁻¹
- Type 2: 2A + B → products
The rate law is: Rate = k[A]²[B]
Analyzing the dimensions:
- Rate: mol L⁻¹ s⁻¹
- [A]²[B]: (mol L⁻¹)²(mol L⁻¹) = mol³ L⁻³
Therefore, the units of k are:
k = (mol L⁻¹ s⁻¹) / (mol³ L⁻³) = L² mol⁻² s⁻¹
- Type 3: A + B + C → products
The rate law is: Rate = k[A][B][C]
Analyzing the dimensions:
- Rate: mol L⁻¹ s⁻¹
- [A][B][C]: (mol L⁻¹)(mol L⁻¹)(mol L⁻¹) = mol³ L⁻³
Again, the units of k are:
k = (mol L⁻¹ s⁻¹) / (mol³ L⁻³) = L² mol⁻² s⁻¹
The Significance of Consistent Units
The consistent derivation of L² mol⁻² s⁻¹ (or M² s⁻¹) for all forms of third-order reactions highlights the importance of dimensional analysis in chemical kinetics. Incorrect units indicate an error in either the rate law or the experimental determination of the rate constant. Maintaining dimensional consistency is crucial for ensuring the accuracy and reliability of kinetic studies.
Practical Applications and Interpretation of Third-Order Rate Constants
The units of k, besides ensuring dimensional consistency, offer insights into the reaction mechanism and the likelihood of a third-order reaction occurring. Third-order reactions are relatively uncommon due to the low probability of three reactant molecules simultaneously colliding with the correct orientation and energy to react. However, they do exist, and understanding their rate constants is vital.
Experimental Determination of k
Experimentally determining k involves measuring the reaction rate at different reactant concentrations and fitting the data to the appropriate integrated rate law. The units of k obtained from this process should always be checked for consistency with the derived units based on the reaction order. Any discrepancy necessitates a re-examination of the experimental data or the proposed rate law.
Analyzing Reaction Mechanisms
The magnitude of k provides information about the reaction's speed and efficiency. A large value of k indicates a fast reaction, while a small value suggests a slow reaction. This information, combined with the understanding of the reaction mechanism (often obtained through mechanistic studies), allows for a comprehensive interpretation of the reaction kinetics.
Predicting Reaction Rates
Once the rate constant k is known, it can be used to predict the reaction rate at any given set of reactant concentrations. This is crucial for applications in various fields, including industrial chemistry, environmental science, and pharmacology, where controlling or predicting reaction rates is essential.
Beyond Third-Order Reactions: Generalizing the Concept
The method used to determine the units of k for third-order reactions can be generalized to reactions of any order. The general approach involves analyzing the units of the rate and the concentration terms in the rate law to obtain the units of the rate constant. For an nth-order reaction with a rate law of the form:
Rate = k[A]ⁿ
The units of k will be:
(concentration¹⁻ⁿ time⁻¹)
Conclusion: The Importance of Understanding Units in Chemical Kinetics
The units of the rate constant k are a fundamental aspect of chemical kinetics, providing insights into the reaction order, mechanism, and overall reaction rate. For third-order reactions, the consistent units of L² mol⁻² s⁻¹ (or M² s⁻¹) underscore the importance of dimensional analysis and accurate experimental data. Understanding these units is essential for accurate interpretations, predictions, and applications of reaction rate data across various scientific and engineering disciplines. Furthermore, a thorough understanding of the principles governing the units of rate constants facilitates a deeper appreciation of reaction mechanisms and the complexities of chemical kinetics as a whole. This detailed analysis of third-order reactions provides a solid foundation for tackling the intricacies of higher-order reactions and for advancing one's understanding of reaction dynamics in chemical systems.
Latest Posts
Latest Posts
-
The Left Gastric Artery Supplies
Apr 09, 2025
-
An Organisms Observable Traits Are Referred To As Its
Apr 09, 2025
-
It Is Then Cooled And
Apr 09, 2025
-
Which Of The Following Is Not A Function Of Packaging
Apr 09, 2025
-
Which Is True Regarding Blood Carried In The Veins
Apr 09, 2025
Related Post
Thank you for visiting our website which covers about Units For Rate Constant K Third Order . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.