Geometric Mean Of 9 And 4
News Leon
Apr 02, 2025 · 5 min read
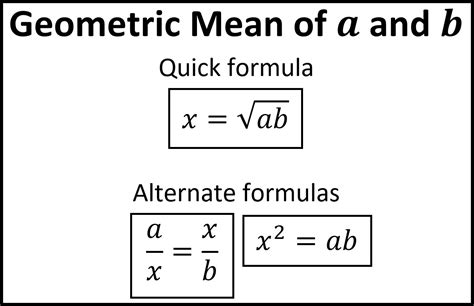
Table of Contents
Unveiling the Geometric Mean: A Deep Dive into the Relationship Between 9 and 4
The geometric mean, often overlooked in favor of its more familiar cousin, the arithmetic mean, holds a unique and powerful place in mathematics and various applications. While the arithmetic mean provides the average of a set of numbers, the geometric mean offers a different perspective, particularly useful when dealing with multiplicative relationships or rates of change. This article delves deep into the concept of the geometric mean, focusing specifically on the geometric mean of 9 and 4, illustrating its calculation, interpretation, and significance within a broader mathematical context.
Understanding the Geometric Mean
The geometric mean is a type of average that indicates the central tendency or typical value of a set of numbers by using the product of their values (as opposed to the arithmetic mean which uses their sum). For a set of n non-negative numbers, the geometric mean is calculated as the nth root of the product of those numbers. Formally:
Geometric Mean = ⁿ√(x₁ * x₂ * x₃ * ... * xₙ)
Where:
- x₁, x₂, x₃, ..., xₙ represent the individual numbers in the set.
- n represents the total number of numbers in the set.
This formula highlights a crucial difference between the geometric and arithmetic means: the geometric mean considers the multiplicative relationships between numbers, while the arithmetic mean focuses on their additive relationships. This distinction becomes especially relevant when dealing with percentages, rates of growth, or situations where the product of values is more meaningful than their sum.
Calculating the Geometric Mean of 9 and 4
Let's apply this concept to find the geometric mean of 9 and 4. In this case, we have two numbers (n = 2), x₁ = 9, and x₂ = 4. Substituting these values into the formula, we get:
Geometric Mean = √(9 * 4) = √36 = 6
Therefore, the geometric mean of 9 and 4 is 6.
Interpreting the Geometric Mean: Beyond Simple Calculation
The result, 6, isn't merely a numerical answer; it holds a specific meaning within the context of the numbers 9 and 4. Consider these interpretations:
-
Proportional Relationships: The geometric mean of 9 and 4 represents a value that maintains the same ratio between itself and the original numbers. Notice that 9/6 = 1.5 and 6/4 = 1.5. This consistent ratio signifies a balanced proportional relationship between the three numbers.
-
Geometric Sequences: Imagine a geometric sequence where the first term is 4 and the third term is 9. The geometric mean (6) represents the missing middle term in this sequence. This is because in a geometric sequence, the ratio between consecutive terms remains constant.
-
Growth Rates: In financial applications or scenarios involving exponential growth, the geometric mean provides a more accurate representation of average growth than the arithmetic mean. If a quantity grows by a factor of 9 in one period and by a factor of 4 in another, the geometric mean indicates the average growth factor per period.
Contrasting with the Arithmetic Mean
To further illustrate the difference, let's calculate the arithmetic mean of 9 and 4:
Arithmetic Mean = (9 + 4) / 2 = 6.5
The difference between the arithmetic mean (6.5) and the geometric mean (6) highlights the crucial distinction between additive and multiplicative relationships. The arithmetic mean focuses on the sum, while the geometric mean considers the product. In situations where multiplicative relationships dominate (e.g., compound interest, population growth), the geometric mean provides a more accurate and insightful representation of the average.
Applications of the Geometric Mean
The geometric mean finds applications in diverse fields:
1. Finance and Investment:
-
Average Rate of Return: Calculating the average return on investment over multiple periods, especially when dealing with fluctuating returns. The geometric mean avoids the overestimation that can occur with the arithmetic mean in volatile markets.
-
Compound Interest: Determining the average annual growth rate of an investment subject to compound interest.
2. Geometry:
-
Similar Triangles: Finding the average length of corresponding sides in similar triangles.
-
Mean Proportional: Establishing proportional relationships between geometric figures.
3. Statistics and Data Analysis:
-
Averaging Ratios: Calculating the average ratio across different samples, especially useful when the ratios are skewed.
-
Log-transformed Data: Dealing with data that follows a log-normal distribution.
4. Engineering and Physics:
-
Average Velocity: Calculating the average velocity over time intervals when the velocity changes non-linearly.
-
Signal Processing: Determining average signal strength.
The Geometric Mean in a Broader Mathematical Context
The concept of the geometric mean is intricately linked to other mathematical concepts. Understanding these connections enhances its overall significance:
-
Logarithms: The geometric mean can be conveniently calculated using logarithms. The logarithm of the geometric mean is the arithmetic mean of the logarithms of the individual numbers. This property simplifies calculations, especially when dealing with a large number of values.
-
Inequalities: The geometric mean is always less than or equal to the arithmetic mean (this is known as the AM-GM inequality). This inequality provides a powerful tool in mathematical proofs and problem-solving.
-
Weighted Geometric Mean: Similar to the weighted arithmetic mean, there exists a weighted geometric mean, providing flexibility to assign different weights to individual numbers according to their importance.
Advanced Concepts and Extensions
For those interested in exploring more advanced aspects, the following topics warrant further investigation:
-
Generalized Mean: The geometric mean is a special case of the generalized mean, which encompasses a family of means parameterized by a real number (including the arithmetic, geometric, and harmonic means).
-
Multivariate Geometric Mean: Extending the geometric mean to multiple dimensions, offering applications in vector analysis and higher-dimensional data analysis.
-
Geometric Mean of Functions: The concept of the geometric mean can also be extended to functions, opening doors to applications in calculus and functional analysis.
Conclusion: Embracing the Power of the Geometric Mean
The geometric mean, while seemingly simpler than the arithmetic mean, offers a profound understanding of multiplicative relationships and provides a valuable tool in diverse fields. Its ability to accurately capture average rates of change, proportional relationships, and growth factors makes it an indispensable concept in mathematics and various applications. By understanding its calculation, interpretation, and connections to other mathematical concepts, one unlocks its power and usefulness in analyzing data and modeling real-world phenomena. This detailed exploration of the geometric mean, with a specific focus on the geometric mean of 9 and 4, provides a comprehensive foundation for appreciating its significance and leveraging its applications. The seemingly simple calculation of 6 hides a wealth of mathematical depth and practical relevance.
Latest Posts
Latest Posts
-
Transcription Takes Place In The Nucleus Cytoplasm
Apr 03, 2025
-
Is Delta H Positive For Endothermic
Apr 03, 2025
-
Cytoplasm Of The Cell Is Being Divided
Apr 03, 2025
-
Which Elements Has Only One Valence Electron
Apr 03, 2025
-
Co2 Enters The Inner Spaces Of The Leaf Through The
Apr 03, 2025
Related Post
Thank you for visiting our website which covers about Geometric Mean Of 9 And 4 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
