Geometric Mean Of 9 And 16
News Leon
Apr 06, 2025 · 6 min read
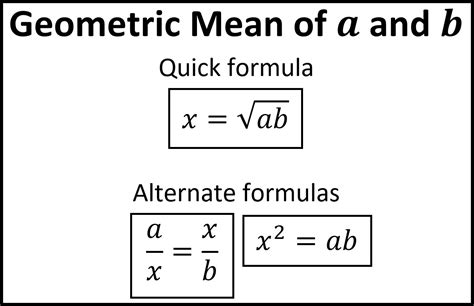
Table of Contents
Delving Deep into the Geometric Mean: A Comprehensive Exploration Using 9 and 16
The geometric mean (GM) might seem like a niche mathematical concept, but its applications are surprisingly widespread, extending far beyond the classroom. Understanding the geometric mean is crucial in various fields, from finance and investment analysis to statistics and even image processing. This article will provide a comprehensive exploration of the geometric mean, using the numbers 9 and 16 as a running example to illustrate its calculation, properties, and practical applications.
What is the Geometric Mean?
The geometric mean is a type of average that indicates the central tendency or typical value of a set of numbers by using the product of their values (as opposed to the arithmetic mean which uses their sum). It's particularly useful when dealing with positive numbers and is often preferred over the arithmetic mean when dealing with rates of change, ratios, or values that grow exponentially. For a set of n numbers, the geometric mean is calculated as the nth root of the product of those numbers.
For our example, let's consider the numbers 9 and 16. The geometric mean is calculated as follows:
√(9 * 16) = √144 = 12
Therefore, the geometric mean of 9 and 16 is 12.
Geometric Mean vs. Arithmetic Mean: Key Differences
It's important to understand the difference between the geometric mean and the arithmetic mean (AM). The arithmetic mean is simply the sum of the numbers divided by the count of numbers. For 9 and 16, the arithmetic mean is:
(9 + 16) / 2 = 12.5
Notice the difference? While in this case the difference is relatively small, the disparity can become significant when dealing with more diverse datasets or datasets with extreme values. The geometric mean is less sensitive to outliers and extreme values compared to the arithmetic mean.
When to Use the Geometric Mean over the Arithmetic Mean:
-
Proportional Growth: The geometric mean is ideal for calculating average growth rates over time, especially when dealing with compound interest or exponential growth. For instance, calculating the average annual return of an investment over several years, where each year's return is multiplicative, requires the geometric mean.
-
Rates and Ratios: When working with rates or ratios (e.g., speed, efficiency), the geometric mean provides a more accurate representation than the arithmetic mean.
-
Log-Normal Distributions: If your data follows a log-normal distribution (where the logarithm of the data follows a normal distribution), the geometric mean is a more appropriate measure of central tendency than the arithmetic mean. This is frequently encountered in various fields, including finance and biology.
-
Reducing the Impact of Outliers: As mentioned earlier, the geometric mean is less susceptible to distortion from extreme values or outliers present in a dataset compared to the arithmetic mean. This robustness makes it a preferred choice for analyzing data with potential outliers.
Applications of the Geometric Mean
The geometric mean's applications are extensive and span across various disciplines:
1. Finance and Investment:
-
Average Investment Returns: Investors often use the geometric mean to calculate the average annual return of an investment portfolio over a number of years. This is more accurate than using the arithmetic mean because it accounts for the compounding effect of returns.
-
Analyzing Stock Performance: The geometric mean can help determine the average growth rate of a stock over a period, providing a clearer picture of its long-term performance compared to the arithmetic mean which might be skewed by short-term fluctuations.
-
Measuring Portfolio Performance: Financial professionals use the geometric mean to compare the performance of different investment portfolios, accounting for the compounding effect of returns, offering a more accurate assessment of overall portfolio growth.
2. Statistics and Data Analysis:
-
Describing Central Tendency: When dealing with positively skewed data or data containing outliers, the geometric mean offers a more robust measure of central tendency compared to the arithmetic mean.
-
Log-Normal Distributions: As mentioned before, datasets following a log-normal distribution, common in various natural phenomena and financial markets, are best described using the geometric mean.
3. Engineering and Science:
-
Calculating Average Ratios: In engineering, the geometric mean is frequently used when calculating average ratios or proportions, offering a more meaningful average compared to the arithmetic mean in cases of proportional relationships.
-
Image Processing: The geometric mean is applied in image processing for tasks like image averaging, reducing noise, and enhancing contrast. It helps maintain the integrity of image data.
4. Other Applications:
The geometric mean finds its application in various other fields:
-
Sports Analytics: It can be utilized in sports analytics to analyze average player performance over multiple seasons, providing a more robust measure than the arithmetic mean.
-
Environmental Science: Environmental studies use the geometric mean to average various environmental indicators, like pollution levels, providing a more balanced view.
-
Public Health: Epidemiological studies may employ the geometric mean when analyzing the average rate of disease incidence or prevalence, offering a better representation.
Properties of the Geometric Mean
The geometric mean possesses several important mathematical properties:
-
Always Less Than or Equal to the Arithmetic Mean: For a given set of positive numbers, the geometric mean will always be less than or equal to the arithmetic mean. Equality holds only when all the numbers in the set are equal.
-
Scale Invariance: The geometric mean is unaffected by changes in scale. Multiplying each number in the set by a constant does not change the geometric mean.
-
Multiplicative Property: The geometric mean of the product of two sets of numbers is the product of their individual geometric means.
-
Relationship with Logarithms: The logarithm of the geometric mean is equal to the arithmetic mean of the logarithms of the individual numbers. This property allows for easier computation, especially when dealing with a large number of values.
Calculating the Geometric Mean for Larger Datasets
The formula for calculating the geometric mean for more than two numbers is:
GM = (x₁ * x₂ * x₃ * ... * xₙ)^(1/n)
Where:
- GM is the geometric mean.
- x₁, x₂, x₃, ..., xₙ are the numbers in the dataset.
- n is the number of numbers in the dataset.
For example, let's calculate the geometric mean of the numbers 2, 4, 8, and 16:
GM = (2 * 4 * 8 * 16)^(1/4) = (1024)^(1/4) = 4
Conclusion: The Importance of Understanding the Geometric Mean
The geometric mean, despite its seemingly specialized nature, is a powerful tool with applications across diverse fields. Its ability to provide a robust measure of central tendency, particularly when dealing with multiplicative relationships, growth rates, and datasets with outliers, makes it an indispensable mathematical concept. By understanding its calculation, properties, and applications, you can leverage its power for more accurate and insightful analysis in your own work. The examples using 9 and 16 served as a clear, introductory illustration of its basic calculation and its contrast with the arithmetic mean. As you delve deeper into more complex datasets and applications, you'll appreciate its value further. Remember, the correct choice of average—arithmetic or geometric—depends heavily on the context and the nature of the data being analyzed. Understanding this nuance is crucial for accurate and meaningful interpretation of results.
Latest Posts
Latest Posts
-
Where Does Glycolysis Occur In Eukaryotic Cells
Apr 09, 2025
-
Contain Carbon Hydrogen Oxygen And Nitrogen
Apr 09, 2025
-
What Is The Empirical Formula For Ibuprofen
Apr 09, 2025
Related Post
Thank you for visiting our website which covers about Geometric Mean Of 9 And 16 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.