Evaluate 3 To The Power Of 4
News Leon
Apr 07, 2025 · 5 min read
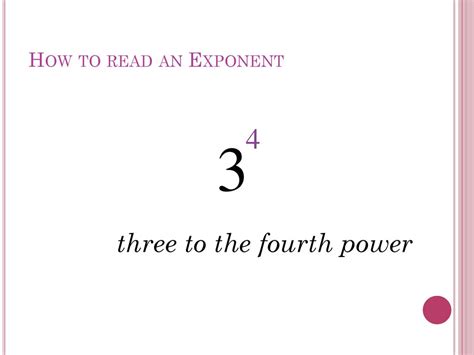
Table of Contents
Evaluating 3 to the Power of 4: A Deep Dive into Exponentiation
Evaluating 3 to the power of 4, or 3⁴, might seem like a simple task at first glance. However, understanding this seemingly basic calculation opens the door to a broader comprehension of exponentiation, its applications in various fields, and its significance in mathematics. This article will delve into the intricacies of this calculation, exploring its meaning, methods of solving it, and its implications within the wider mathematical landscape.
What Does 3⁴ Actually Mean?
Before we jump into the solution, let's solidify the fundamental concept. The expression 3⁴ represents exponential notation, a shorthand way of writing repeated multiplication. It means that the base number, 3, is multiplied by itself four times (the exponent). Therefore, 3⁴ is equivalent to 3 x 3 x 3 x 3.
Calculating 3⁴: The Simple Approach
The most straightforward way to calculate 3⁴ is through direct multiplication. We simply perform the following steps:
- 3 x 3 = 9
- 9 x 3 = 27
- 27 x 3 = 81
Therefore, 3⁴ = 81. This method is easily manageable for small exponents like 4, but it becomes increasingly cumbersome as the exponent grows larger.
Understanding the Properties of Exponents
Mastering exponentiation requires understanding its fundamental properties. These properties offer alternative methods for calculating exponents and simplify complex expressions. Let's look at a few relevant ones:
-
Product of Powers: When multiplying two numbers with the same base, you can add the exponents: aᵐ x aⁿ = aᵐ⁺ⁿ. For example, 3² x 3³ = 3²⁺³ = 3⁵ = 243.
-
Quotient of Powers: When dividing two numbers with the same base, you can subtract the exponents: aᵐ / aⁿ = aᵐ⁻ⁿ (where a ≠ 0). For example, 3⁵ / 3² = 3⁵⁻² = 3³ = 27.
-
Power of a Power: When raising a power to another power, you multiply the exponents: (aᵐ)ⁿ = aᵐⁿ. For example, (3²)³ = 3²ˣ³ = 3⁶ = 729.
-
Power of a Product: When raising a product to a power, you raise each factor to that power: (ab)ⁿ = aⁿbⁿ. For example, (3 x 2)² = 3² x 2² = 9 x 4 = 36.
-
Power of a Quotient: When raising a quotient to a power, you raise both the numerator and the denominator to that power: (a/b)ⁿ = aⁿ/bⁿ (where b ≠ 0). For example, (3/2)² = 3²/2² = 9/4 = 2.25.
Applying Properties to Calculate 3⁴
While direct multiplication is efficient for 3⁴, let's demonstrate the application of these properties. We can express 3⁴ using the product of powers:
3⁴ = 3² x 3² = 9 x 9 = 81
Beyond the Basics: Larger Exponents and Scientific Notation
As exponents increase, manual multiplication becomes less practical. Consider 3¹⁰. Direct calculation would be tedious. In such cases, calculators or computer programs become essential. For extremely large exponents, scientific notation becomes invaluable. Scientific notation expresses numbers in the form a x 10ᵇ, where 'a' is a number between 1 and 10, and 'b' is an integer exponent.
Applications of Exponentiation in Real-World Scenarios
Exponentiation isn't just a mathematical concept; it has widespread practical applications across diverse fields:
-
Compound Interest: The growth of money in a savings account with compound interest is governed by exponential functions. The formula A = P(1 + r/n)^(nt) illustrates this, where A is the future value, P is the principal amount, r is the annual interest rate, n is the number of times interest is compounded per year, and t is the time in years.
-
Population Growth: Modeling population growth often involves exponential functions. Factors like birth rates, death rates, and migration influence the rate of exponential growth or decline.
-
Radioactive Decay: The decay of radioactive materials follows an exponential decay model. The half-life of a radioactive substance, the time it takes for half of the substance to decay, is a key concept in this area.
-
Computer Science: Exponentiation plays a crucial role in algorithms and data structures. For example, the time complexity of certain algorithms can be expressed using exponential functions, indicating how the algorithm's runtime scales with the input size.
The Significance of the Base and Exponent
The choice of base and exponent significantly impacts the outcome. A change in either drastically alters the result. For instance, comparing 3⁴ (81) with 4³ (64) highlights this variability. Understanding this relationship is crucial for interpreting results in various applications.
Exploring Related Mathematical Concepts
Understanding 3⁴ lays a foundation for exploring related mathematical concepts:
-
Logarithms: Logarithms are the inverse function of exponentiation. The logarithm base 'b' of a number 'x' is the exponent to which 'b' must be raised to produce 'x'. In the context of 3⁴ = 81, the logarithm base 3 of 81 is 4 (log₃81 = 4).
-
Exponential Functions and Equations: Exponential functions are functions where the variable appears as an exponent. These functions are frequently used to model growth and decay processes.
-
Series and Sequences: Many mathematical series and sequences involve exponential terms, such as geometric series where each term is multiplied by a constant ratio.
Advanced Techniques for Evaluating Large Exponents
For extremely large exponents, numerical methods and approximation techniques are often necessary. These methods leverage computational power to handle calculations that are impractical to perform manually. These include:
-
Binary Exponentiation: This algorithm uses the binary representation of the exponent to reduce the number of multiplications needed.
-
Taylor Series Expansion: This method approximates the value of exponential functions using an infinite series.
Conclusion: The Power of Understanding 3⁴
While evaluating 3⁴ might seem elementary, it serves as a gateway to understanding a broader range of mathematical concepts. The principles of exponentiation, along with the properties of exponents, have widespread applications across numerous fields. Understanding these principles allows for tackling more complex problems and developing a deeper appreciation for the elegance and power of mathematics. From compound interest calculations to modeling population growth, the fundamental concept of raising a number to a power remains a cornerstone of mathematical and scientific understanding. The simple calculation of 3⁴ ultimately opens doors to a richer comprehension of the world around us.
Latest Posts
Latest Posts
-
How Many Electrons Can Occupy The 3d Subshell
Apr 09, 2025
-
Difference Between And Enzyme And A Hormone
Apr 09, 2025
-
Which Of The Following Is A Path Function
Apr 09, 2025
-
A 100ml Sample Of 0 1 M Mgcl2
Apr 09, 2025
-
What Is The Prime Factorization Of 360
Apr 09, 2025
Related Post
Thank you for visiting our website which covers about Evaluate 3 To The Power Of 4 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.