Does A Trapezoid Have Right Angles
News Leon
Apr 06, 2025 · 5 min read
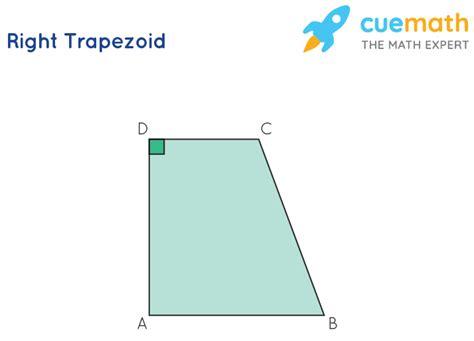
Table of Contents
Does a Trapezoid Have Right Angles? Exploring the Geometry of Trapezoids
Trapezoids, those quadrilateral shapes with at least one pair of parallel sides, often spark curiosity about their angles. A common question that arises is: Does a trapezoid have right angles? The simple answer is: not necessarily. While some trapezoids do possess right angles, it's not a defining characteristic of the shape. Let's delve deeper into the geometry of trapezoids, exploring the different types and uncovering the nuances of their angular properties.
Understanding the Definition of a Trapezoid
Before we dissect the presence (or absence) of right angles in trapezoids, let's establish a clear understanding of what constitutes a trapezoid. A trapezoid, also known as a trapezium in some regions, is a quadrilateral with at least one pair of parallel sides. These parallel sides are called bases, while the other two sides are known as legs or lateral sides.
It's crucial to distinguish between different types of trapezoids. The presence or absence of right angles significantly influences the categorization and properties of these shapes.
Types of Trapezoids and Their Angular Characteristics
Trapezoids can be broadly classified into several categories based on their angles and side lengths:
1. Isosceles Trapezoid:
An isosceles trapezoid is a trapezoid where the legs are congruent (equal in length). In an isosceles trapezoid, the base angles are congruent. This means that the angles adjacent to each base are equal. However, this doesn't automatically imply right angles. An isosceles trapezoid can have acute angles, obtuse angles, or a combination of both, as long as the base angles are congruent.
2. Right Trapezoid:
This is where the question of right angles becomes relevant. A right trapezoid is defined as a trapezoid with at least one right angle. Notice the "at least one" qualifier. A right trapezoid can have one, two, or even three right angles (although three right angles would imply a rectangle). The key is that it possesses at least one 90-degree angle.
3. Scalene Trapezoid:
A scalene trapezoid has no congruent sides. This implies that neither its bases nor its legs are equal in length. Furthermore, the angles of a scalene trapezoid will also be unequal. It is possible for a scalene trapezoid to have one right angle, but it's not guaranteed. The angles can be any combination of acute and obtuse angles, as long as the sum of interior angles remains 360 degrees.
Properties of Trapezoids: Angles and Sides
The sum of the interior angles of any quadrilateral, including a trapezoid, is always 360 degrees. This fundamental property helps determine the possible angle combinations in trapezoids. Knowing this helps us analyze scenarios where right angles might exist.
Let's consider a trapezoid ABCD, where AB is parallel to CD. If angle A is a right angle (90 degrees), then angle D must be supplementary to angle A (180 - 90 = 90 degrees), while angles B and C can be any combination that adds up to 180 degrees. Thus, a trapezoid can have two right angles, one at each of the parallel sides.
However, if we have a trapezoid with only one right angle, the other three angles would need to sum to 270 degrees. This would mean a combination of angles that include both acute and obtuse angles, showcasing the possible diversity in trapezoid angles.
Visualizing Trapezoids with and without Right Angles
Imagine different scenarios:
-
Right Trapezoid: Picture a trapezoid where one leg is perpendicular to both bases. This creates two right angles, forming a right-angled triangle and a rectangle if it's extended.
-
Isosceles Trapezoid with Acute Angles: Visualize a trapezoid with two parallel sides and two equal legs sloping inwards, creating acute base angles.
-
Isosceles Trapezoid with Obtuse Angles: Picture a trapezoid where the legs slope outwards, forming obtuse base angles.
-
Scalene Trapezoid with One Right Angle: Imagine a trapezoid with a right angle formed by one leg and one base. The remaining angles are a mix of acute and obtuse, illustrating the lack of uniformity.
-
Scalene Trapezoid with No Right Angles: Imagine a trapezoid with four unequal sides and no 90-degree angles, a completely unconstrained shape, except for the parallel bases.
Applying the Knowledge: Problem Solving with Trapezoids
The understanding of trapezoid angles is vital for solving geometric problems. For example, if you're given the lengths of the bases and the height of a right trapezoid, you can easily calculate its area using the formula: Area = (1/2) * (sum of bases) * height. However, if you are only given the lengths of all four sides, determining the angles and hence the area requires more complex calculations, possibly involving trigonometric functions.
Distinguishing Trapezoids from Other Quadrilaterals
It's important to differentiate trapezoids from other quadrilaterals:
-
Parallelogram: A parallelogram has two pairs of parallel sides, unlike a trapezoid which only needs one pair. All parallelograms (rectangles, squares, rhombuses) have properties that distinguish them from trapezoids.
-
Rectangle: A rectangle is a parallelogram with four right angles.
-
Square: A square is a rectangle with all sides equal.
-
Rhombus: A rhombus is a parallelogram with all sides equal.
The key distinction lies in the number of parallel sides and the presence of right angles.
Conclusion: The Variability of Trapezoid Angles
In conclusion, a trapezoid does not necessarily have right angles. While a right trapezoid is a valid subtype, the defining characteristic of a trapezoid is the presence of at least one pair of parallel sides. The angles of a trapezoid can be acute, obtuse, or a combination thereof, depending on the specific type and dimensions of the trapezoid. Understanding the different types of trapezoids and their angular properties is crucial for accurate geometric calculations and problem-solving. Remember to apply the fundamental property of the sum of interior angles (360 degrees) to check the validity of any angle combinations in a trapezoid. This detailed exploration should help clarify the sometimes-confusing nature of trapezoid angles and highlight the rich diversity within this fascinating geometrical shape.
Latest Posts
Latest Posts
-
Is Sound Wave A Mechanical Wave
Apr 08, 2025
-
Draw The Monomer For The Following Polymer
Apr 08, 2025
-
What Is The Primary Source Of Energy On Earth
Apr 08, 2025
-
During Which Process Is Chromosome Number Reduced
Apr 08, 2025
-
An Oxidizing Agent Is A Substance That
Apr 08, 2025
Related Post
Thank you for visiting our website which covers about Does A Trapezoid Have Right Angles . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.