An Oscillator Consists Of A Block Attached To A Spring
News Leon
Mar 26, 2025 · 5 min read
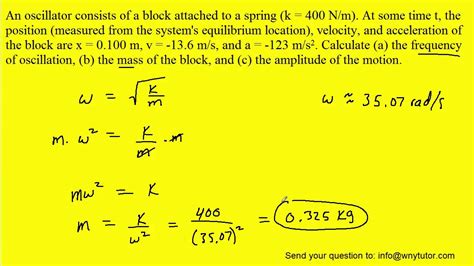
Table of Contents
An Oscillator: A Deep Dive into the Block-Spring System
The seemingly simple system of a block attached to a spring represents a fundamental concept in physics – the simple harmonic oscillator. Understanding its behavior unlocks the door to comprehending a vast range of phenomena, from the swinging of a pendulum to the vibrations of atoms within a molecule. This article delves deep into the mechanics, mathematics, and applications of this quintessential oscillatory system.
Understanding Simple Harmonic Motion (SHM)
At the heart of the block-spring system lies simple harmonic motion (SHM). SHM is defined by a restoring force that is directly proportional to the displacement from equilibrium and acts in the opposite direction. In our block-spring system, this restoring force is provided by the spring, obeying Hooke's Law:
F = -kx
Where:
- F is the restoring force exerted by the spring
- k is the spring constant (a measure of the spring's stiffness)
- x is the displacement from the equilibrium position
The negative sign indicates that the force always acts to return the block to its equilibrium position. When the block is displaced to the right (positive x), the spring pulls it to the left (negative force), and vice versa.
Analyzing the Motion Mathematically
The equation of motion for a simple harmonic oscillator can be derived using Newton's second law (F = ma), where 'a' is the acceleration. Substituting Hooke's Law, we get:
ma = -kx
This second-order differential equation describes the oscillatory behavior. Its solution is a sinusoidal function:
x(t) = A cos(ωt + φ)
Where:
- x(t) is the displacement as a function of time
- A is the amplitude (maximum displacement from equilibrium)
- ω is the angular frequency (related to the period and frequency of oscillation)
- φ is the phase constant (determines the initial position and velocity)
The angular frequency is related to the spring constant (k) and the mass (m) of the block by:
ω = √(k/m)
This equation highlights the crucial relationship between the system's properties and its oscillatory frequency. A stiffer spring (larger k) leads to a higher frequency, while a larger mass (larger m) leads to a lower frequency.
Key Characteristics of SHM
Several key characteristics define simple harmonic motion:
- Period (T): The time taken for one complete oscillation. It's related to the angular frequency by: T = 2π/ω
- Frequency (f): The number of oscillations per unit time. It's the reciprocal of the period: f = 1/T
- Amplitude (A): The maximum displacement from the equilibrium position. It determines the energy of the oscillator.
Beyond the Ideal: Damping and Driving Forces
The simple harmonic oscillator model discussed above is an idealization. Real-world systems often experience damping and are subjected to driving forces.
Damping: Energy Dissipation
Damping refers to the dissipation of energy from the oscillator, typically due to friction or air resistance. This energy loss causes the amplitude of oscillations to decrease over time. The equation of motion becomes more complex, incorporating a damping term. Different types of damping exist, leading to different decay patterns:
- Underdamped: Oscillations decay gradually.
- Critically damped: The system returns to equilibrium as quickly as possible without oscillating.
- Overdamped: The system returns to equilibrium slowly without oscillating.
Driving Forces: Maintaining Oscillations
Driving forces are external forces applied to the oscillator, often periodic in nature. These forces can counteract energy losses due to damping, maintaining oscillations or even increasing their amplitude. A classic example is a child pumping a swing – the rhythmic pushing acts as a driving force. The response of the oscillator to a driving force depends on the frequency of the force relative to the oscillator's natural frequency. Resonance occurs when the driving frequency matches the natural frequency, leading to a significant amplification of the amplitude.
Applications of the Block-Spring System
The simple harmonic oscillator, although a seemingly simple model, finds widespread applications across various fields:
1. Mechanical Systems:
- Clocks and watches: The precise timing in many mechanical clocks relies on the regular oscillations of a balance wheel and spring system.
- Shock absorbers: In vehicles, shock absorbers utilize damped harmonic oscillators to absorb energy from bumps and vibrations, providing a smoother ride.
- Musical instruments: The vibrations of strings in stringed instruments, or the air column in wind instruments, are examples of damped driven oscillators producing sound.
2. Electrical Systems:
- LC circuits: In electronics, an inductor (L) and a capacitor (C) form an LC circuit that exhibits simple harmonic oscillations of electrical charge and current.
- Quartz crystal oscillators: These oscillators utilize the piezoelectric properties of quartz crystals to generate highly stable and accurate frequencies, crucial in clocks, computers, and communication systems.
3. Atomic and Molecular Systems:
- Molecular vibrations: Atoms within molecules vibrate around their equilibrium positions, exhibiting simple harmonic motion (to a first approximation). This vibrational motion plays a crucial role in molecular spectroscopy.
- Atomic clocks: These highly precise clocks utilize the resonant frequencies of atoms to measure time with extraordinary accuracy.
Advanced Concepts and Extensions
The simple harmonic oscillator model serves as a foundation for understanding more complex oscillatory systems. Some extensions and advanced concepts include:
- Anharmonic oscillators: Systems where the restoring force is not directly proportional to the displacement. These systems exhibit more complex oscillatory behavior.
- Coupled oscillators: Systems where multiple oscillators interact with each other, leading to phenomena such as normal modes and energy transfer.
- Nonlinear oscillators: Systems where the equation of motion is nonlinear, resulting in chaotic behavior in certain parameter ranges.
Conclusion: A Fundamental Building Block of Physics
The seemingly simple system of a block attached to a spring reveals profound insights into the nature of oscillatory motion. Understanding simple harmonic motion, damping, and driving forces provides a foundational understanding for analyzing a wide range of physical phenomena across various disciplines. From the intricate workings of mechanical devices to the fundamental vibrations of atoms, the block-spring oscillator remains a critical concept in physics and engineering. Its simplicity belies its importance as a building block for understanding the complex oscillatory world around us. Further exploration into the advanced concepts mentioned above will unlock a deeper appreciation for the richness and complexity of oscillatory systems. The seemingly simple block-spring system is anything but simple when viewed through the lens of advanced physics and its diverse applications.
Latest Posts
Latest Posts
-
How Many Ones Are There Between 1 And 100
Mar 29, 2025
-
025 Expressed As A Percentage Is
Mar 29, 2025
-
What Is The Difference Between A Democracy And Dictatorship
Mar 29, 2025
-
Bill Is To Law As Larva Is To
Mar 29, 2025
-
Which Organelle Is Responsible For Cellular Respiration
Mar 29, 2025
Related Post
Thank you for visiting our website which covers about An Oscillator Consists Of A Block Attached To A Spring . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
