32 Is 40 Percent Of What Number
News Leon
Apr 15, 2025 · 4 min read
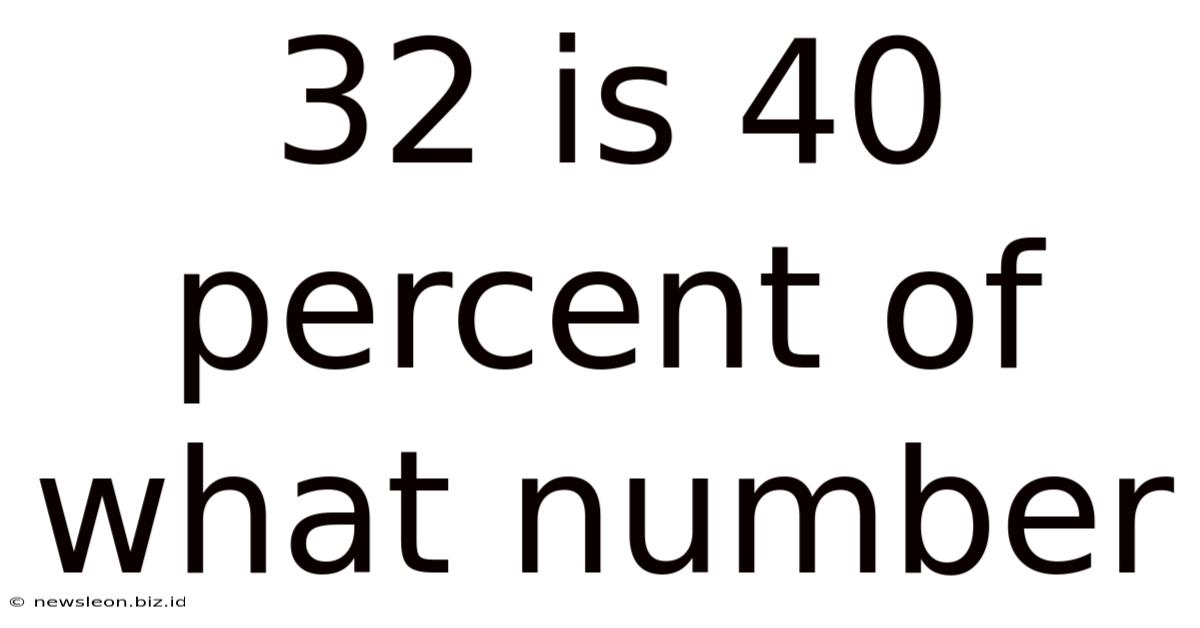
Table of Contents
32 is 40 Percent of What Number: A Comprehensive Guide to Percentage Calculations
Solving percentage problems is a fundamental skill applicable across various fields, from everyday budgeting to complex financial analysis. Understanding how to calculate percentages allows you to interpret data, make informed decisions, and communicate effectively using numerical representations. This article delves into the problem "32 is 40 percent of what number," providing a step-by-step solution, exploring different approaches, and offering practical examples to solidify your understanding.
Understanding Percentages
Before tackling the core problem, let's establish a clear understanding of what percentages represent. A percentage is a fraction or ratio expressed as a number out of 100. The symbol "%" signifies "per hundred." For example, 40% means 40 out of 100, which can be expressed as the fraction 40/100 or the decimal 0.40.
Method 1: Using the Percentage Formula
The most straightforward method to solve "32 is 40 percent of what number" involves utilizing the basic percentage formula:
Part = Percentage × Whole
In this equation:
- Part: Represents the portion of the whole we are given (in our case, 32).
- Percentage: Represents the rate or proportion expressed as a percentage (40% or 0.40).
- Whole: Represents the total or complete value we need to find.
To solve for the "Whole," we rearrange the formula:
Whole = Part / Percentage
Substituting the known values:
Whole = 32 / 0.40
Whole = 80
Therefore, 32 is 40 percent of 80.
Method 2: Setting up a Proportion
Another effective method involves setting up a proportion. A proportion is a statement that two ratios are equal. We can express the problem as follows:
32/x = 40/100
Where 'x' represents the unknown whole number.
To solve for 'x', we cross-multiply:
32 × 100 = 40 × x
3200 = 40x
Now, divide both sides by 40:
x = 3200 / 40
x = 80
Again, we arrive at the conclusion that 32 is 40 percent of 80.
Method 3: Using Decimal Equivalents
Percentages can easily be converted into decimal equivalents by dividing the percentage by 100. In this case, 40% becomes 0.40. The problem can then be rephrased as:
32 = 0.40 × x
To solve for 'x', we divide both sides by 0.40:
x = 32 / 0.40
x = 80
This method yields the same result: 32 is 40 percent of 80.
Practical Applications and Real-World Examples
Understanding percentage calculations extends beyond theoretical exercises. Here are several practical applications:
1. Sales and Discounts:
Imagine a store offers a 40% discount on an item originally priced at $80. The discount amount is calculated as:
Discount = 40% × $80 = $32
The final sale price would then be:
Sale Price = $80 - $32 = $48
This example demonstrates how our core calculation applies directly to real-world shopping scenarios.
2. Tax Calculations:
Suppose a sales tax rate is 40%, and the tax amount on a purchase is $32. To determine the pre-tax price, we can use the same percentage formula:
Pre-tax Price = Tax Amount / Tax Rate
Pre-tax Price = $32 / 0.40 = $80
This shows how percentage calculations are crucial for understanding the total cost of goods and services, including taxes.
3. Financial Analysis:
In finance, percentage changes are commonly used to track investment performance, profit margins, and other key indicators. Understanding percentage calculations is essential for interpreting financial statements and making informed investment decisions. For instance, if an investment grows by 40% and the growth in dollar terms is $32, the original investment value can be calculated using the same principle.
4. Grade Calculations:
Percentage calculations are frequently used to determine grades in educational settings. If a student scores 32 points out of a possible 80 points, their percentage score is:
(32 / 80) × 100% = 40%
This highlights the application of percentage calculations in assessing academic performance.
Expanding Your Understanding: Further Percentage Problems
Let's consider variations of the problem to reinforce your understanding:
- What is 25% of 120? (Solution: 30)
- If 15 is 75% of a number, what is the number? (Solution: 20)
- What percentage of 60 is 18? (Solution: 30%)
Solving these problems using the methods described earlier will strengthen your proficiency in percentage calculations.
Conclusion: Mastering Percentage Calculations
The ability to solve percentage problems like "32 is 40 percent of what number" is a highly valuable skill with widespread applications. This article has presented several methods for tackling such problems, emphasizing the importance of understanding the underlying principles. By mastering these techniques, you can confidently approach various percentage-related challenges in your personal and professional life, enhancing your analytical abilities and decision-making processes. Remember to practice regularly to build fluency and accuracy in your calculations. The more you practice, the more intuitive these processes will become. You'll find yourself effortlessly solving percentage problems in various contexts, from everyday budgeting to complex financial scenarios.
Latest Posts
Related Post
Thank you for visiting our website which covers about 32 Is 40 Percent Of What Number . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.