12.5 Of What Number Is 24
News Leon
Apr 07, 2025 · 4 min read
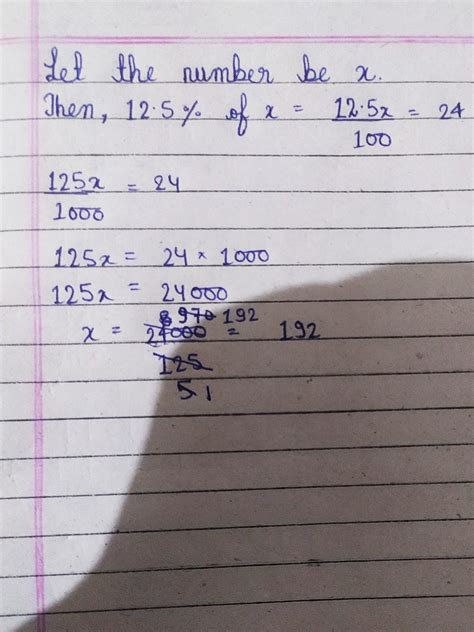
Table of Contents
12.5% of What Number is 24? Solving Percentage Problems
Finding the answer to "12.5% of what number is 24?" involves understanding and applying the fundamental principles of percentages. This seemingly simple question opens the door to a broader understanding of percentage calculations, their applications in various fields, and different approaches to solving them. This comprehensive guide will not only provide the solution but also delve into the underlying concepts, alternative methods, and practical examples.
Understanding Percentages
A percentage is a fraction or ratio expressed as a part of 100. The symbol "%" represents "per cent," meaning "out of one hundred." For instance, 50% means 50 out of 100, which is equivalent to the fraction 50/100 or the decimal 0.5. Understanding this fundamental concept is crucial for solving percentage problems.
Different Approaches to Solving Percentage Problems
There are several ways to solve percentage problems, and choosing the most efficient method depends on the specific problem and your comfort level with different mathematical approaches. Let's explore the most common methods:
Method 1: Using the Formula
The most direct way to solve "12.5% of what number is 24?" is to use the percentage formula:
Part / Whole = Percentage / 100
In this problem:
- Part: 24 (this is the given part of the whole)
- Percentage: 12.5 (this is the percentage we're working with)
- Whole: This is what we need to find (let's represent it with 'x')
Substituting these values into the formula, we get:
24 / x = 12.5 / 100
To solve for x, we can cross-multiply:
24 * 100 = 12.5 * x
2400 = 12.5x
Now, divide both sides by 12.5:
x = 2400 / 12.5
x = 192
Therefore, 12.5% of 192 is 24.
Method 2: Converting Percentage to Decimal
Another approach involves converting the percentage to its decimal equivalent. 12.5% is equal to 0.125 (divide 12.5 by 100). The problem can then be rewritten as:
0.125 * x = 24
To solve for x, divide both sides by 0.125:
x = 24 / 0.125
x = 192
This method offers a slightly simpler calculation compared to cross-multiplication.
Method 3: Using Proportions
This method utilizes the concept of ratios and proportions. We can set up a proportion as follows:
12.5 / 100 = 24 / x
Cross-multiplying, we get:
12.5x = 2400
Dividing both sides by 12.5:
x = 2400 / 12.5
x = 192
This method is conceptually similar to the formula method but visually presents the relationship between the parts and the whole more clearly.
Verifying the Solution
It's always good practice to verify your answer. Let's check if 12.5% of 192 is indeed 24:
(12.5 / 100) * 192 = 0.125 * 192 = 24
The calculation confirms our solution.
Practical Applications of Percentage Calculations
Percentage calculations are indispensable in various real-world scenarios. Here are a few examples:
- Finance: Calculating interest rates, discounts, taxes, profit margins, and investment returns.
- Retail: Determining sale prices, markups, and discounts.
- Science: Expressing experimental results, statistical data, and error margins.
- Everyday Life: Understanding tips, taxes, and calculating proportions of ingredients in recipes.
Understanding percentage calculations empowers you to make informed decisions in these and many other areas of life.
Advanced Percentage Problems and Concepts
While the problem "12.5% of what number is 24?" is relatively straightforward, understanding percentages extends to more complex scenarios. These include:
- Compound Interest: Interest earned not only on the principal amount but also on accumulated interest.
- Percentage Change: Calculating the increase or decrease in a quantity as a percentage.
- Percentage Point Difference: The difference between two percentages.
Tips for Solving Percentage Problems Effectively
Here are some tips to help you efficiently solve percentage problems:
- Understand the terminology: Make sure you clearly understand the meaning of "of," "is," and "what number" in the context of percentage problems.
- Choose the appropriate method: Select the method that best suits your understanding and the specific problem.
- Check your work: Always verify your answer to ensure accuracy.
- Practice regularly: Solving various percentage problems regularly will improve your speed and accuracy.
- Use a calculator: For more complex calculations, using a calculator is highly recommended.
Conclusion: Mastering Percentage Calculations
The seemingly simple question, "12.5% of what number is 24?", has led us on a journey through the world of percentage calculations. We explored different methods for solving the problem, highlighted the practical applications of percentages, and touched upon more advanced concepts. By understanding the fundamental principles and practicing regularly, you can confidently tackle various percentage problems encountered in academic, professional, and everyday life situations. Remember, the key to mastering percentages lies in understanding the underlying concepts and applying appropriate methods to solve different types of problems. The ability to perform these calculations efficiently and accurately is a valuable skill applicable across various domains. Keep practicing, and you'll soon become proficient in solving percentage-related challenges.
Latest Posts
Latest Posts
-
An Electron Has A Charge Of
Apr 10, 2025
-
How Does The Endoplasmic Reticulum Provide Mechanical Support
Apr 10, 2025
-
How To Calculate The Energy Released
Apr 10, 2025
-
Which Of These Constitutes The Pectoral Girdle
Apr 10, 2025
-
If Abc Dbc Then B Is The Midpoint Of Ad
Apr 10, 2025
Related Post
Thank you for visiting our website which covers about 12.5 Of What Number Is 24 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.