0.67 As A Fraction In Simplest Form
News Leon
Apr 07, 2025 · 4 min read
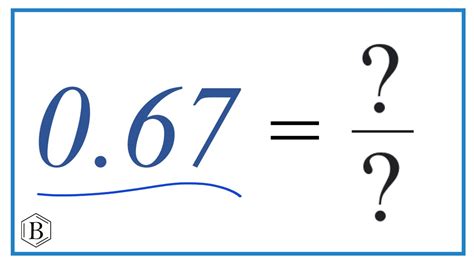
Table of Contents
0.67 as a Fraction in Simplest Form: A Comprehensive Guide
Converting decimals to fractions might seem daunting at first, but with a systematic approach, it becomes a straightforward process. This comprehensive guide will walk you through converting the decimal 0.67 into its simplest fraction form, explaining each step in detail and providing valuable context for similar conversions. We'll also delve into the broader concepts of decimal-to-fraction conversion and explore some related mathematical ideas.
Understanding Decimals and Fractions
Before we embark on the conversion, let's establish a clear understanding of decimals and fractions.
-
Decimals: Decimals represent numbers that are not whole numbers. They use a decimal point to separate the whole number part from the fractional part. The digits to the right of the decimal point represent fractions of powers of 10 (tenths, hundredths, thousandths, and so on).
-
Fractions: Fractions represent parts of a whole. They are expressed as a ratio of two integers, the numerator (top number) and the denominator (bottom number). The denominator indicates the total number of parts, and the numerator indicates how many of those parts are being considered.
Converting 0.67 to a Fraction: Step-by-Step
The process of converting a decimal to a fraction involves several key steps:
Step 1: Identify the place value of the last digit.
In 0.67, the last digit, 7, is in the hundredths place. This means that 0.67 represents 67 hundredths.
Step 2: Write the decimal as a fraction.
Based on Step 1, we can write 0.67 as the fraction 67/100. The numerator is 67 (the digits after the decimal point), and the denominator is 100 (since the last digit is in the hundredths place).
Step 3: Simplify the fraction (if possible).
This is the crucial step in finding the simplest form of the fraction. Simplifying a fraction means reducing it to its lowest terms by dividing both the numerator and the denominator by their greatest common divisor (GCD).
To find the GCD of 67 and 100, we can use the Euclidean algorithm or prime factorization. In this case, the GCD of 67 and 100 is 1. This means that 67/100 is already in its simplest form because 67 is a prime number and doesn't share any common factors with 100 other than 1.
Therefore, the simplest form of 0.67 as a fraction is 67/100.
Exploring Related Concepts
Understanding the conversion of 0.67 to a fraction provides a foundation for tackling other decimal-to-fraction conversions. Let's explore some related concepts and examples:
Converting Terminating Decimals
Terminating decimals, like 0.67, have a finite number of digits after the decimal point. The conversion process is similar for all terminating decimals:
- Write the decimal as a fraction with a denominator that is a power of 10. (e.g., 10, 100, 1000, etc.)
- Simplify the fraction to its lowest terms.
Example: Convert 0.25 to a fraction.
0.25 = 25/100
Simplifying 25/100 by dividing both numerator and denominator by 25, we get 1/4.
Converting Repeating Decimals
Repeating decimals have digits that repeat infinitely. Converting these to fractions requires a slightly different approach. We will not delve into this here as 0.67 is a terminating decimal.
Mixed Numbers and Improper Fractions
Sometimes, you might encounter decimals greater than 1. In such cases, the resulting fraction might be an improper fraction (where the numerator is greater than the denominator) which can then be expressed as a mixed number (a whole number and a proper fraction).
Example: Convert 1.25 to a fraction.
1.25 = 125/100 = 5/4 = 1 ¼
Practical Applications
Understanding decimal-to-fraction conversions is essential in various fields:
- Baking and Cooking: Recipes often require precise measurements, and converting fractions to decimals or vice versa is common.
- Engineering and Construction: Accurate calculations are critical, and fractions are sometimes preferred for precision.
- Finance: Working with percentages and interest rates involves converting decimals to fractions frequently.
- Data Analysis: Understanding the relationship between decimals and fractions aids in interpreting data represented in different formats.
Advanced Concepts: Continued Fractions
For those interested in delving deeper, the concept of continued fractions offers another way to represent numbers, including decimals. A continued fraction expresses a number as a sum of integers and fractions of integers. While not directly related to simplifying 0.67, understanding continued fractions provides a richer perspective on number representation.
Conclusion
Converting 0.67 to its simplest fraction form, 67/100, is a straightforward process involving identifying the place value of the last digit, writing it as a fraction, and simplifying the fraction to its lowest terms. This fundamental concept extends to other decimal-to-fraction conversions, providing valuable skills applicable across various disciplines. By mastering this conversion process, you enhance your mathematical proficiency and improve your ability to handle numerical data effectively. Remember that practice is key to developing fluency in converting decimals to fractions and understanding the underlying mathematical principles.
Latest Posts
Latest Posts
-
Which Of The Following Is Not A Strong Acid
Apr 08, 2025
-
Every Rhombus Is A Quadrilateral True Or False
Apr 08, 2025
-
Find The Geometric Mean Of 9 And 16
Apr 08, 2025
-
Can A Compound Be Separated By Physical Means
Apr 08, 2025
-
Find The Magnitude Of The Electric Dipole Moment
Apr 08, 2025
Related Post
Thank you for visiting our website which covers about 0.67 As A Fraction In Simplest Form . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.